Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-20T06:32:32.138Z Has data issue: false hasContentIssue false
  • English
  • Français

Zeros of linear combinations of orthogonal polynomials

Published online by Cambridge University Press:  24 October 2008

Franz Peherstorfer
Franz Peherstorfer
Affiliation:
Institut für Mathematik, J. KeplerUniversität Linz, A-4040 Linz, Austria
Get access Rights & Permissions [Opens in a new window]

Abstract

Let ψ be a distribution function on [−1,1] from the Szegö-class, which contains in particular all Jacobi weights, and let (pn) be the monic polynomials orthogonal with respect to dψ. Let m(n)∈ℕ, n∈ℕ, be non-decreasing with limn → ∞ (n − m(n)) = ∞, l(n)∈ℕ with 0 ≤ l(n) ≤ m(n), and μj, n ∈ℝ for j = 0, …, m(n), n∈ℕ. It is shown that for each sufficiently large n, has n−l(n) simple zeros in (−1, 1)and l(n) zeros in ℂ\[−1,1] if for n ≥ n0, 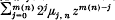has m(n) − l(n) zeros in the disc |z| ≤ r < 1, l(n) zeros outside of the disc |z| ≥ R > 1 and 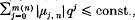where q > 2 max {r, 1/R}. If m(n) is constant for n ≥ n0 then the statement holds even for such polynomials (pn) orthogonal with respect to a distribution dψ satisfying the weak assumption ψ′ > 0 a.e. on [−1, 1]. For linear combinations of polynomials orthogonal on the unit circle corresponding results are derived.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 117 , Issue 3 , May 1995 , pp. 533 - 544
Copyright
Copyright © Cambridge Philosophical Society 1995

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Erdös, P. and Turan, P.. On interpolation I. Annals Math. 38 (1937), 142–155.CrossRefGoogle Scholar
[2]Fejer, L.. Mechanische Quadraturen mit positiven Cotesschen Zahlen. Math. Zeit. 37 (1933), 287–309.CrossRefGoogle Scholar
[3]Freud, G.. Orthogonale Polynome (Birkhäuser Verlag, 1969).Google Scholar
[4]Geronimus, Ya. L.. Polynomials orthogonal on a circle and their applications. Zap. Nauchn. Issled. Inst. Mat. Mekh. Khar'kov Mat. Obsc. (4), 19 (1948), 35–120; Amer. Math. Soc. Transl. Ser. 13 (1962), 1–78.Google Scholar
[5]Geronimus, Ya. L.. Polynomials orthogonal on a circle and interval (Pergamon Press, 1960).Google Scholar
[6]Iserles, A. and Saff, E. B.. Zeros of expansions in orthogonal polynomials. Math. Proc. Camb. Phil. Soc. 105 (1989), 559–573.CrossRefGoogle Scholar
[7]Iserles, A., Norsett, S. and Safe, E.. On transformations and zeros of polynomials. Rocky Mntn J. Math. 21 (1991), 331–357.Google Scholar
[8]Marden, M.. Geometry of polynomials (American Mathematical Society, 1966).Google Scholar
[9]Micchelli, C. A. and Rivlin, T. J.. Numerical integration rules near gaussian quadrature. Israel J. Math. 16 (1973), 267–299.CrossRefGoogle Scholar
[10]Obreschkoff, N.. Verteilung und Berechnung der Nullstellen reeller Polynome (VEB Deutscher Verlag der Wissenschaften, 1963).Google Scholar
[11]Peherstorfer, F.. Characterizations of quadrature formulas II, SIAM J. Math. Anal. 15 (1984), 1021–1030.Google Scholar
[12]Peherstorfer, F.. Linear combinations of orthogonal polynomials generating positive quadrature formulas. Math. Comp. 55 (1990), 231–241.CrossRefGoogle Scholar
[13]Peherstorfer, F.. On the asymptotic behaviour of functions of the second kind and Stieltjes polynomials and on the Gauss-Kronrod quadrature formulas. J. Approx. Theory 70 (1992), 156–190.CrossRefGoogle Scholar
[14]Peherstorfer, F.. A special class of polynomials orthogonal on the unit circle including the associated polynomials, Constr. Approx., to appear.Google Scholar
[15]Polya, G.. Über die Nullstellen gewisser ganzer Funktionen Math. Zeit. 2 (1918), 352–383.Google Scholar
[16]Rakhmanov, A.. On the asymptotics of the ratio of orthogonal polynomials II. Math. USSR Sbornik 46 (1983), 105–117.Google Scholar
[17]Shohat, J.. On mechanical quadratures in particular, with positive coefficients. Trans. Amer. Math. Soc. 42 (1937), 461–196.CrossRefGoogle Scholar
[18]Specht, W.. Die Lage der Nullstellen eines Polynoms. Math. Nachr. 15 (1956), 353–374.Google Scholar
[19]Specht, W.. Die Lage der Nullstellen eines Polynoms II. Math. Nachr. 16 (1957), 257–263.Google Scholar
[20]Specht, W.. Die Lage der Nullstellen eines Polynoms III. Math. Nachr. 16 (1957), 369–389.CrossRefGoogle Scholar
[21]Specht, W.. Algebraische Gleichungen mit reellen oder komplexen Koeffizienten (Enzyklopädie der Mathematischen Wissenschaften, Band I, Heft 3, B. G. Teubner Verlag, 1958).Google Scholar
[22]Szegö, G.. Orthogonal Polynomials, 4th edition (American Mathematical Society, 1975).Google Scholar
[23]Turan, P.. Sur l'algêbre fonctionelle. Comptes Rendus du Premier Congrès des Mathematiciens Hongrois, 267–290, Akademiai Kiado Budapest 1952.Google Scholar
[24]Turan, P.. Hermite-expansion and strips for zeros of polynomials. Arch. Math. (Basel) 5 (1954), 148–152.CrossRefGoogle Scholar