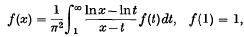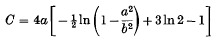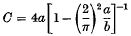Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Leppington, F. G.
and
Levine, H.
1972.
Some axially symmetric potential problems.
Proceedings of the Edinburgh Mathematical Society,
Vol. 18,
Issue. 1,
p.
55.
Love, E. R.
and
Collins, W. D.
1976.
20.—Inequalities for the Capacity of an Electrified Conducting Annular Disc.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 74,
Issue. ,
p.
257.
Selim, Elsayed O.
and
Waters, Ronald T.
1980.
Static Probe for Electrostatic Field Measurement in the Presence of Space Charge.
IEEE Transactions on Industry Applications,
Vol. IA-16,
Issue. 3,
p.
458.
Waters, R. T.
1983.
Electrical Breakdown and Discharges in Gases.
p.
203.
Davis, A. M. J.
1991.
Stokes drag on a narrow annular disk sedimenting in the presence of fixed boundaries or other disks.
Physics of Fluids A: Fluid Dynamics,
Vol. 3,
Issue. 2,
p.
249.
Suehiro, J
Yamamoto, K
and
Hara, M
1991.
Mobilities of charge carriers generated by corona discharge in low temperature helium gas.
Journal of Physics D: Applied Physics,
Vol. 24,
Issue. 8,
p.
1360.
Davis, A. M. J.
1993.
A hydrodynamic model of the oscillating screen viscometer.
Physics of Fluids A: Fluid Dynamics,
Vol. 5,
Issue. 9,
p.
2095.
Xin-Min Shi
Yumoto, M.
and
Sakai, T.
1999.
On Tassicker's measurement formula in the boundary biased-probe method.
IEEE Transactions on Industry Applications,
Vol. 35,
Issue. 3,
p.
549.
Singh, B. M.
Rokne, J. G.
and
Dhaliwal, R. S.
2007.
Two-Dimensional Electrostatic Problem in a Plane with Earthed Elliptic Cavity due to One or Two Collinear Charged Electrostatic Strips.
International Journal of Mathematics and Mathematical Sciences,
Vol. 2007,
Issue. ,
p.
1.
Nouri, H.
and
Zebboudj, Y.
2010.
Analysis of positive corona in wire-to-plate electrostatic precipitator.
The European Physical Journal Applied Physics,
Vol. 49,
Issue. 1,
p.
11001.
Ait Said, H.
Nouri, H.
and
Zebboudj, Y.
2015.
Effect of air flow on corona discharge in wire-to-plate electrostatic precipitator.
Journal of Electrostatics,
Vol. 73,
Issue. ,
p.
19.
Nouri, Hamou
Achouri, Imed Eddine
Said, Hakim Ait
Aissou, Massinissa
and
Zebboudj, Youcef
2019.
Analysis of Temperature Effect in laboratory scale electrostatic precipitator.
p.
1.