No CrossRef data available.
Published online by Cambridge University Press: 24 October 2008
If X is a space with base point, let ΩX denote the space of loops of X based at its base point. We will denote the homology and cohomology suspensions by σ* and σ*, respectively, 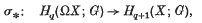 ,
, 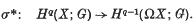 . Let K(π, n) be the Eilenberg-MacLane complex with only one non-vanishing homotopy group πn(K(π, n))= π, πi(K(π, n)) = 0 for i ≠ n. If π is abelian K(π, n) = ΩK(π, n+1). We will assume that π is a finitely generated abelian group throughout this note. Let K(π, n) × K(π, n) → K(π, n) denote the multiplication in K(π, n).
. Let K(π, n) be the Eilenberg-MacLane complex with only one non-vanishing homotopy group πn(K(π, n))= π, πi(K(π, n)) = 0 for i ≠ n. If π is abelian K(π, n) = ΩK(π, n+1). We will assume that π is a finitely generated abelian group throughout this note. Let K(π, n) × K(π, n) → K(π, n) denote the multiplication in K(π, n).