Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-26T07:36:09.488Z Has data issue: false hasContentIssue false
  • English
  • Français

Relations in the tautological ring derived from combinatorial classes and hyperelliptic fatgraphs

Published online by Cambridge University Press:  01 March 2008

ALEX JAMES BENE
ALEX JAMES BENE*
Affiliation:
Department of Mathematics, University of Southern California, 3620 S. Vermont Avenue, Los Angeles, CA 90089-2532, U.S.A. e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A closed formula is obtained for the integral 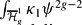of tautological classes over the locus of hyperelliptic Weier points in the moduli space of curves. As a corollary, a relation between Hodge integrals is obtained.

The calculation utilizes the homeomorphism between the moduli space of curves 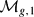and the combinatorial moduli space , a PL-orbifold whose cells are enumerated by fatgraphs. This cell decomposition can be used to naturally construct combinatorial PL-cycles 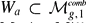whose homology classes are essentially the Poin duals of the Mumford–Morita–Miller classes κa. In this paper we construct another PL-cycle 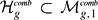representing the locus of hyperelliptic Weier points and explicitly describe the chain level intersection of this cycle with W1. Using this description of 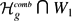, the duality between Witten cycles Wa and the κa classes, and the Kontsevich--Penner method of integration, scheme of integrating ε classes, the integral 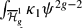is reduced to a weighted sum over graphs and is evaluated by the enumeration of trees.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 144 , Issue 2 , March 2008 , pp. 369 - 395
Copyright
Copyright © Cambridge Philosophical Society 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Arbarello, E. and Cornalba, M.Combinatorial and algebro-geometric cohomology classes on the moduli space of curves. J. Algebraic Geom. 5 (1996), 705–749.Google Scholar
[2]Bertin, J. and Romagny, M. Champs de Hurwitz. pre-print (2006)Google Scholar
[3]Conant, J. and Vogtmann, K.On a theorem of Kontsevich. Algebr. Geom. Topol. 3 (2003), 1167–1224.CrossRefGoogle Scholar
[4]Faber, C. and Pandharipande, R.Hodge integrals and Gromov–Witten theory. Invent. Math. 139 (2000), 173–199.CrossRefGoogle Scholar
[5]Fulton, W.Intersection Theory 2nd edition (Springer-Verlag, 1998).CrossRefGoogle Scholar
[6]Fulton, W.Introduction to toric varieties. Ann. Math. Stud., 131. (Princeton University Press, 1993).CrossRefGoogle Scholar
[7]Goulden, I. and Jackson, D.Combinatorial enumeration (John Wiley & Sons Inc., 1983).Google Scholar
[8]Harer, J.The virtual cohomological dimension of the mapping class group of an orientable surface. Invent. Math. 84 (1986), 157–176.CrossRefGoogle Scholar
[9]Harer, J. and Zagier, D.The Euler characteristic of the moduli space of curves. Invent. Math. 85 (1986), 457–485.CrossRefGoogle Scholar
[10]Harris, J. and Morgan, J.Moduli of curves (Springer-Verlag, 1998).Google Scholar
[11]Igusa, K.Combinatorial Miller–Morita–Mumford classes and Witten cycles. Algebr. Geom. Topol. 4 (2004), 473–520.CrossRefGoogle Scholar
[12]Igusa, K.Graph cohomology and Kontsevich cycles. Topology 43 (2004), 1469–1510.CrossRefGoogle Scholar
[13]Igusa, K. and Kleber, M.Increasing trees and Kontsevich cycles. Geom. Topol. 8 (2004), 969–1012.CrossRefGoogle Scholar
[14]Kontsevich, M.Intersection theory on the moduli space of curves and the matrix Airy function. Comm. Math. Phys. 147 (1992), 763–787.CrossRefGoogle Scholar
[15]Looijenga, E. Cellular decompositions of compactified moduli spaces of pointed curves. The moduli space of curves (Birkhauser Boston, 1995), 369–400.CrossRefGoogle Scholar
[16]Mondello, G.. Combinatorial classes on g,n are tautological. Int. Math. Res. Not. 44 (2004), 2329–2390.CrossRefGoogle Scholar
[17]Mumford, D. Towards an enumerative geometry of the moduli space of curves. Arithemetic and Geometry, vol. 2. (Birkhauser, 1983), 271–328.CrossRefGoogle Scholar
[18]Penner, R.The decorated Teichmüller space of puctured surfaces. Comm. Math. Phys. 113 (1987), 299–339.CrossRefGoogle Scholar
[19]Penner, R.Perturbative series and the moduli space of Riemann surfaces. J. Differential Geom. 27 (1988), 35–53.CrossRefGoogle Scholar
[20]Penner, R.Weil–Petersson volumes. J. Differential Geom. 35 (1992), 559–608.CrossRefGoogle Scholar
[21]Penner, R.The Poincare dual of the Weil–Petersson Kähler two-form. Comm. Anal. Geom. 1 (1993), 43–69.CrossRefGoogle Scholar
[22]Penner, R.Cell decomposition and compactification of Riemann's moduli space in decorated Teichmüller theory. Woods Hole mathematics, series on knots and everything 34 (World Sci. Publ., 2004), 263–301.CrossRefGoogle Scholar
[23]Strebell, K.Quadratic differentials (Springer-Verlag, 1984).CrossRefGoogle Scholar
[24]Witten, E.Two-dimensional gravity and intersection theory on moduli space. Surv. Differ. Geom. 1 (1991), 243–310.CrossRefGoogle Scholar
[25]Zvonkine, D. Strebel differentials on stable curves and Kontsevich's proof of Witten's conjecture. (2004) e-print: math.AG/0209071Google Scholar