Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-30T19:46:48.571Z Has data issue: false hasContentIssue false
  • English
  • Français

On the lines passing through two conjugates of a Salem number

Published online by Cambridge University Press:  01 January 2008

ARTŪRAS DUBICKAS  and
CHRIS SMYTH
ARTŪRAS DUBICKAS
Affiliation:
Department of Mathematics and Informatics, Vilnius University, Naugarduko 24, Vilnius LT- 03225, Lithuania. e-mail: [email protected]
CHRIS SMYTH
Affiliation:
School of Mathematics and Maxwell Institute for Mathematical Sciences, University of Edinburgh, James Clerk Maxwell Bld., King's Buildings, Mayfield Road Edinburgh EH 9 3JZ, Scotland. e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that the number of distinct non-parallel lines passing through two conjugates of an algebraic number α of degree d ≥ 3 is at most [d2/2]-d+2, its conjugates being in general position if this number is attained. If, for instance, d ≥ 4 is even, then the conjugates of α ∈ 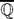of degree d are in general position if and only if α has 2 real conjugates, d-2 complex conjugates, no three distinct conjugates of α lie on a line and any two lines that pass through two distinct conjugates of α are non-parallel, except for d/2-1 lines parallel to the imaginary axis. Our main result asserts that the conjugates of any Salem number are in general position. We also ask two natural questions about conjugates of Pisot numbers which lead to the equation α1+α2=α3+α4 in distinct conjugates of a Pisot number. The Pisot number 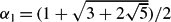shows that this equation has such a solution.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 144 , Issue 1 , January 2008 , pp. 29 - 37
Copyright
Copyright © Cambridge Philosophical Society 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Mignotte, M.. Sur les conjugées des nombres de Pisot. C. R. Acad. Sci. Paris Sér. I. Math 298 (1984), 21.Google Scholar
[2]Serre, J.-P.. Topics in Galois Theory. Research Notes in Mathematics 1 (Jones & Bartlett, 1992).Google Scholar
[3]Smyth, C. J.. The conjugates of algebraic integers. Amer. Math. Monthl 82 (1975), 86.CrossRefGoogle Scholar
[4]Smyth, C. J.. Conjugate algebraic numbers on conics. Acta Arith. 40 (1982), 333–346.CrossRefGoogle Scholar
[5]Salem, R.. Algebraic Numbers and Fourier Analysis (D. C. Heath, 1963).Google Scholar