Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-26T13:50:37.490Z Has data issue: false hasContentIssue false
  • English
  • Français

On Self-reciprocal functions for Fourier-Bessel integral transforms

Published online by Cambridge University Press:  24 October 2008

Afzal Ahmad  and
V. Lakshmikanth
Afzal Ahmad
Affiliation:
Osmania UniversityHyderabad (A.P.), India
V. Lakshmikanth
Affiliation:
Osmania UniversityHyderabad (A.P.), India
Get access Rights & Permissions [Opens in a new window]

Extract

Following Hardy and Titchmarsh(1) a function f(x) is said to be self-reciprocal if it satisfies the Fourier-Bessel integral transform

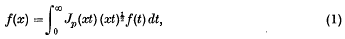
where Jp(x) is a Bessel function of order P ≥ –½. This integral is denoted by Rp. The special cases P ½ and P ½, we denote by Rs and Rc, respectively.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 57 , Issue 4 , October 1961 , pp. 778 - 781
Copyright
Copyright © Cambridge Philosophical Society 1961

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Hardy, G. H. and Titchmarsh, E. C., Self reciprocal functions. Quart. J. Math. (2), 1 (1930), 196–231.Google Scholar
(2)Lakshmikanth, V. On the relation between the self-reciprocal functions and kernels (submitted for publication).Google Scholar
(3)Lakshmikanth, V., Some self-reciprocal functions. Proc. Nat. Acad. Sci. (India), Part A, 28 (1959), 246–8.Google Scholar
(4)Lakshmikanth, V., Some self-reciprocal functions and kernels. Proc. Camb. Phil. Soc. 57 (1961), 690–2.Google Scholar
(5)Wills, H. F., A formula for expanding an integral as series. Phil. Mag. (7), 39 (1948), 455–9.Google Scholar
(6)Watson, G. N., Theory of Bessel Functions (Cambridge, 1922).Google Scholar