Published online by Cambridge University Press: 24 October 2008
We give a ‘picture’ proof to a theorem of M. Freedman (2) which shows the failure of the 4-dimensional homology surgery theory and the homology splitting theorem. Our proof employs the language of the framed links (3) and it involves calculating Casson-Gordon invariant of a certain algebraically slice knot. We use framed links to represent 1-connected 4-manifolds with boundary by attaching 2-handles along them onto B4 via the framings. We adapt the notation ≈ for diffeomorphisms, and 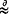 for diffeomorphisms between the boundaries of manifolds. Here manifolds refer to smooth manifolds.
for diffeomorphisms between the boundaries of manifolds. Here manifolds refer to smooth manifolds.