1. Consider the constant-coefficient fifth-order differential equation:

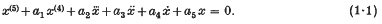
It is known from the general theory that the trivial solution of (1·1) is unstable if, and only if, the associated (auxiliary) equation:

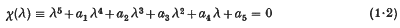
has at least one root with a positive real part. The existence of such a root naturally depends on (though not always all of) the coefficients a1, a2,…, a5. For example, if

it is clear from a consideration of the fact that the sum of the roots of (1·2) equals ( – a1) that at least one root of (1·2) has a positive real part for arbitrary values of a2,…, a5. A similar consideration, combined with the fact that the product of the roots of (1·2) equals ( – a5) will show that at least one root of (1·2) has a positive real part if

for arbitrary a2, a3 and a4. The condition a1 = 0 here in (1·4) is however superfluous when

for then X(0) = a5 < 0 and X(R) > 0 if R > 0 is sufficiently large thus showing that there is a positive real root of (1·2) subject to (1·5) and for arbitrary a1, a2, a3 and a4.