Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-19T22:01:51.679Z Has data issue: false hasContentIssue false
  • English
  • Français

The correlations between relatives in a random mating diploid population

Published online by Cambridge University Press:  24 October 2008

G. B. Trustrum  and
J. H. Williamson
G. B. Trustrum
Affiliation:
Trinity Hall, Cambridge
Get access Rights & Permissions [Opens in a new window]

Extract

Malecot(4) under certain conditions derived the formula for the covariance of the genotypic values of a quantitative character in two individuals AI and AII, which were related but not by direct descent. This generalized some results of Fisher (l). Kempthorne (2) extended the theory to multiple allelic systems with any degree of epistacy (i.e. interlocular genie interaction) but without linkage. He gave the formula 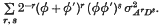Here is the item in the population variance which can be attributed to the interaction of additive gene effects at r loci and dominance gene effects at s loci. φ and φ′ are the coefficients of relation between the two individuals. The various assumptions normally included under random mating equilibrium were made, i.e. no selection, mutations, maternal effects or differential viability. Kempthorne (2), (3) gave two rather different proofs of this important result. His second proof was the more straightforward, but it was somewhat condensed.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 57 , Issue 2 , April 1961 , pp. 315 - 320
Copyright
Copyright © Cambridge Philosophical Society 1961

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Fisher, R. A., On the correlation between relatives on the supposition of Mendelian inheritance. Trans. Roy. Soc. Edinb. 52 (1918), 399–433.CrossRefGoogle Scholar
(2)Kempthorne, O., The correlation between relatives in a random mating population. Proc. Roy. Soc. B, 143 (1954), 103–13.Google Scholar
(3)Kempthorne, O., Introduction to genetic statistics, chapters 15, 19 (London, 1957).Google Scholar
(4)Malecot, G., Les Mathématiques de l'hérédité (Paris, 1948).Google Scholar
(5)Owen, A. R. G., Lectures (unpublished, 1959).Google Scholar
(6)Wright, Sewell., Coefficients of inbreeding and relationship. Amer. Nat. 56 (1922), 330–8.Google Scholar