Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Baues, Hans Joachim
and
Conduché, Daniel
1991.
On the tensor algebra of a non-abelian group and applications.
K-Theory,
Vol. 5,
Issue. 6,
p.
531.
Hernández, Luis Javier
and
Porter, Timothy
1992.
An embedding theorem for proper n-types.
Topology and its Applications,
Vol. 48,
Issue. 3,
p.
215.
Baues, H.G.
and
Brown, R.
1993.
On relative homotogy groups of the product filtration, the James construction, and a formula of Hopf.
Journal of Pure and Applied Algebra,
Vol. 89,
Issue. 1-2,
p.
49.
Bullejos, M.
Carrasco, P.
and
Cegarra, A. M.
1993.
Cohomology with coefficients in symmetric cat-groups. An extension of Eilenberg–MacLane's classification theorem.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 114,
Issue. 1,
p.
163.
Fenn, Roger
Rourke, Colin
and
Sanderson, Brian
1993.
Topics in Knot Theory.
p.
33.
Brown, Ronald
and
Mucuk, Osman
1994.
Covering groups of non-connected topological groups revisited.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 115,
Issue. 1,
p.
97.
Fenn, Roger
Rourke, Colin
and
Sanderson, Brian
1995.
Trunks and classifying spaces.
Applied Categorical Structures,
Vol. 3,
Issue. 4,
p.
321.
Brown, Ronald
and
Salleh, Abdul Razak
1999.
Free Crossed Resolutions of Groups and Presentations of Modules of Identities among Relations.
LMS Journal of Computation and Mathematics,
Vol. 2,
Issue. ,
p.
28.
Brown, Ronald
and
Wensley, Christopher D.
2003.
Computation and homotopical applications of induced crossed modules.
Journal of Symbolic Computation,
Vol. 35,
Issue. 1,
p.
59.
Tonks, A.P.
2003.
On the Eilenberg–Zilber theorem for crossed complexes.
Journal of Pure and Applied Algebra,
Vol. 179,
Issue. 1-2,
p.
199.
Bullejos, M.
Faro, E.
and
García-Muñoz, M.A.
2005.
Postnikov invariants of crossed complexes.
Journal of Algebra,
Vol. 285,
Issue. 1,
p.
238.
Muro, Fernando
and
Tonks, Andrew
2007.
The 1-type of a Waldhausen K-theory spectrum.
Advances in Mathematics,
Vol. 216,
Issue. 1,
p.
178.
Brown, Ronald
2009.
Handbook of Algebra - Volume 6.
Vol. 6,
Issue. ,
p.
83.
Faria Martins, João
2009.
The fundamental crossed module of the complement of a knotted surface.
Transactions of the American Mathematical Society,
Vol. 361,
Issue. 9,
p.
4593.
Carrasco, Pilar
Cegarra, Antonio M.
and
Garzón, Antonio R.
2010.
The classifying space of a categorical crossed module.
Mathematische Nachrichten,
Vol. 283,
Issue. 4,
p.
544.
Martins, João Faria
and
Picken, Roger
2011.
Surface holonomy for non-abelian 2-bundles via double groupoids.
Advances in Mathematics,
Vol. 226,
Issue. 4,
p.
3309.
Ara, Dimitri
2013.
Strict ∞-groupoids are Grothendieck ∞-groupoids.
Journal of Pure and Applied Algebra,
Vol. 217,
Issue. 12,
p.
2298.
Marquis, Jean-Pierre
2014.
Mathematical Models of Abstract Systems: Knowing abstract geometric forms.
Annales de la Faculté des sciences de Toulouse : Mathématiques,
Vol. 22,
Issue. 5,
p.
969.
Basu, Samik
and
Sen, Debasis
2015.
Representing Bredon cohomology with local coefficients.
Journal of Pure and Applied Algebra,
Vol. 219,
Issue. 9,
p.
3992.
Prasma, Matan
2015.
Higher descent data as a homotopy limit.
Journal of Homotopy and Related Structures,
Vol. 10,
Issue. 2,
p.
189.


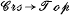 from the category of crossed complexes to the category of spaces.
from the category of crossed complexes to the category of spaces.