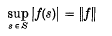Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
1981.
Complex Analysis in Locally Convex Spaces.
Vol. 57,
Issue. ,
p.
433.
Aron, Richard M.
and
Globevnik, Josip
1988.
Interpolation by analytic functions on c0.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 104,
Issue. 2,
p.
295.
Moraes, Luiza Amália
and
Romero Grados, Luis
2003.
Boundaries for algebras of holomorphic functions.
Journal of Mathematical Analysis and Applications,
Vol. 281,
Issue. 2,
p.
575.
Moraes, L.A.
and
Grados, L.-Romero
2004.
BOUNDARIES FOR AN ALGEBRA OF BOUNDED HOLOMORPHIC FUNCTIONS.
Journal of the Korean Mathematical Society,
Vol. 41,
Issue. 1,
p.
231.
Choi, Yun Sung
Garcia, Domingo
Kim, Sung Guen
and
Maestre, Manuel
2004.
Norm or numerical radius attaining polynomials on C(K).
Journal of Mathematical Analysis and Applications,
Vol. 295,
Issue. 1,
p.
80.
Choi, Yun Sung
and
Han, Kwang Hee
2006.
Boundaries for algebras of holomorphic functions on Marcinkiewicz sequence spaces.
Journal of Mathematical Analysis and Applications,
Vol. 323,
Issue. 2,
p.
1116.
Acosta, María D.
Moraes, Luiza A.
and
Romero Grados, Luis
2007.
On boundaries on the predual of the Lorentz sequence space.
Journal of Mathematical Analysis and Applications,
Vol. 336,
Issue. 1,
p.
470.
Acosta, María D.
and
Kim, Sung Guen
2009.
Numerical boundaries for some classical Banach spaces.
Journal of Mathematical Analysis and Applications,
Vol. 350,
Issue. 2,
p.
694.
Kim, Jaegil
and
Lee, Han Ju
2009.
Strong peak points and strongly norm attaining points with applications to denseness and polynomial numerical indices.
Journal of Functional Analysis,
Vol. 257,
Issue. 4,
p.
931.
Choi, Yun Sung
Lee, Han Ju
and
Song, Hyun Gwi
2010.
Bishop’s theorem and differentiability of a subspace of C b (K).
Israel Journal of Mathematics,
Vol. 180,
Issue. 1,
p.
93.
Kim, Sung Guen
and
Lee, Han Ju
2010.
Numerical peak holomorphic functions on Banach spaces.
Journal of Mathematical Analysis and Applications,
Vol. 364,
Issue. 2,
p.
437.
Jordá, Enrique
2013.
Weighted Vector-Valued Holomorphic Functions on Banach Spaces.
Abstract and Applied Analysis,
Vol. 2013,
Issue. ,
p.
1.
Acosta, M. D.
Galindo, P.
and
Lourenço, M. L.
2014.
Boundaries for algebras of analytic functions on function module Banach spaces.
Mathematische Nachrichten,
Vol. 287,
Issue. 7,
p.
729.
Alves, Thiago R
and
Botelho, Geraldo
2019.
Holomorphic functions with distinguished properties on infinite dimensional spaces.
The Quarterly Journal of Mathematics,
Vol. 70,
Issue. 3,
p.
797.
Dimant, Verónica
Lassalle, Silvia
and
Maestre, Manuel
2025.
Fibers and Gleason parts for the maximal ideal space of $${\mathcal {A}}_u(B_{\ell _p})$$.
Banach Journal of Mathematical Analysis,
Vol. 19,
Issue. 1,