1. The conformal mapping of a strip domain in the z-plane on to a parallel strip— parallel, say, to the real axis of the ζ ( = ξ + iμ)-plane—brings about a certain distortion. More precisely: consider a cross-cut on the line ℜz = c joining the two sides of the frontier of the strip domain (in these introductory remarks we suppose for simplicity that there is only one such cross-cut on that line), and denote by ξ1(c) and ξ2(c) the lower and upper bounds of ξ on the image in the ζ-plane. The theorem of Ahlfors (1), now classical, states that

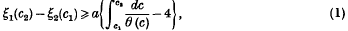
provided that

where a is the width of the parallel strip and θ(c) the length of the cross-cut.