Published online by Cambridge University Press: 24 October 2008
It is well known that, if a trigonometric series
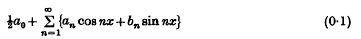
converges to zero in 0 ≤ x < 2π then all the coefficients are zero. To generalize this property of the series, sets of uniqueness have been defined. A point-set E in 0 ≤ x < 2π is a set of uniqueness if every series (0·1), converging to zero in [0, 2π) − E, has zero coefficients. Otherwise E is a set of multiplicity. For example, every enumerable set is a set of uniqueness. An account of the theory may be found in Zygmund (2), chapter 11, pp. 267 et seq.