Published online by Cambridge University Press: 24 October 2008
A quadratic complex Q is the set of lines in 3-dimensional projective space 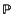 3 given by a single non-trivial quadratic equation in the Plücker coordinates. The lines of the complex which pass through a fixed point of
3 given by a single non-trivial quadratic equation in the Plücker coordinates. The lines of the complex which pass through a fixed point of 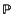 3 are, in general, the generators of a quadric cone; this cone degenerates for the points of a sub variety K of
3 are, in general, the generators of a quadric cone; this cone degenerates for the points of a sub variety K of 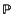 3. Thus one can associate with Q a fibration with base
3. Thus one can associate with Q a fibration with base 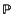 3 - K and fibre isomorphic to
3 - K and fibre isomorphic to 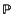 1, and ask whether this fibration is associated with an algebraic vector bundle of rank 2. When the base field is
1, and ask whether this fibration is associated with an algebraic vector bundle of rank 2. When the base field is 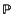 and Q is non-singular, the answer is negative; this was proved some years ago by Narasimhan and Ramanan ((8), proposition 8·1), and has the consequence that there is no universal family of stable vector bundles of rank 2 and degree 0 over a curve of genus 2.
and Q is non-singular, the answer is negative; this was proved some years ago by Narasimhan and Ramanan ((8), proposition 8·1), and has the consequence that there is no universal family of stable vector bundles of rank 2 and degree 0 over a curve of genus 2.