No CrossRef data available.
Article contents
Forming groups with 4 × 4 matrices
Published online by Cambridge University Press: 23 January 2015
Extract
The three Pauli matrices are normally given [1] as the 2 × 2 matrices:
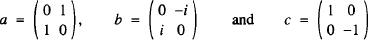
where ‘i’ is the usual complex number imaginary unit.
These matrices obey the relations a2 = I = b2 = c2(where I is the 2 × 2 identity matrix), as well as the anticommutation relations:
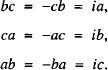
Within the quantities ia,ib and ic,i is a scalar multiplier of the 2 × 2 Pauli matrices and, of course, commutes with each of a, b, c.
- Type
- Articles
- Information
- Copyright
- Copyright © The Mathematical Association 2010
References
1.
Maxwell, E. A., Algebraic structure and matrices, Cambridge University Press (1965) p. 192.Google Scholar
2.
Copson, E. T., Theory of functions of a complex variable, Oxford University Press (1935) pp. 1–5.Google Scholar
4.
Ledermann, W., Introduction to the theory of finite groups (4th edn.), Oliver & Boyd (1961).Google Scholar


