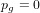 $p_{g}=0$,
$p_{g}=0$, 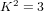 $K^{2}=3$ and 5-torsion
$K^{2}=3$ and 5-torsionPublished online by Cambridge University Press: 01 February 2016
If  $S$ is a quintic surface in
$S$ is a quintic surface in 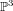 $\mathbb{P}^{3}$ with singular set 15 3-divisible ordinary cusps, then there is a Galois triple cover
$\mathbb{P}^{3}$ with singular set 15 3-divisible ordinary cusps, then there is a Galois triple cover 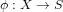 ${\it\phi}:X\rightarrow S$ branched only at the cusps such that
${\it\phi}:X\rightarrow S$ branched only at the cusps such that 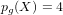 $p_{g}(X)=4$,
$p_{g}(X)=4$, 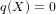 $q(X)=0$,
$q(X)=0$,  $K_{X}^{2}=15$ and
$K_{X}^{2}=15$ and 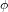 ${\it\phi}$ is the canonical map of
${\it\phi}$ is the canonical map of 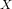 $X$. We use computer algebra to search for such quintics having a free action of
$X$. We use computer algebra to search for such quintics having a free action of 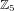 $\mathbb{Z}_{5}$, so that
$\mathbb{Z}_{5}$, so that 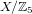 $X/\mathbb{Z}_{5}$ is a smooth minimal surface of general type with
$X/\mathbb{Z}_{5}$ is a smooth minimal surface of general type with 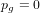 $p_{g}=0$ and
$p_{g}=0$ and 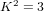 $K^{2}=3$. We find two different quintics, one of which is the van der Geer–Zagier quintic; the other is new.
$K^{2}=3$. We find two different quintics, one of which is the van der Geer–Zagier quintic; the other is new.
We also construct a quintic threefold passing through the 15 singular lines of the Igusa quartic, with 15 cuspidal lines there. By taking tangent hyperplane sections, we compute quintic surfaces with singular sets 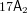 $17\mathsf{A}_{2}$,
$17\mathsf{A}_{2}$, 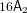 $16\mathsf{A}_{2}$,
$16\mathsf{A}_{2}$, 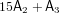 $15\mathsf{A}_{2}+\mathsf{A}_{3}$ and
$15\mathsf{A}_{2}+\mathsf{A}_{3}$ and 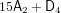 $15\mathsf{A}_{2}+\mathsf{D}_{4}$.
$15\mathsf{A}_{2}+\mathsf{D}_{4}$.