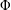Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-24T17:03:20.607Z Has data issue: false hasContentIssue false
  • English
  • Français

$\unicode[STIX]{x1D6F7}$-CARLESON MEASURES AND MULTIPLIERS BETWEEN BERGMAN–ORLICZ SPACES OF THE UNIT BALL OF 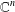$\mathbb{C}^{n}$

Part of: Linear function spaces and their duals Holomorphic functions of several complex variables

Published online by Cambridge University Press:  22 March 2017

BENOÎT F. SEHBA Open the ORCID record for BENOÎT F. SEHBA [Opens in a new window]
BENOÎT F. SEHBA*
Affiliation:
Department of Mathematics, University of Ghana, Legon, P.O. Box LG 62, Legon Accra, Ghana email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We define the notion of $\unicode[STIX]{x1D6F7}$-Carleson measures, where 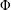$\unicode[STIX]{x1D6F7}$ is either a concave growth function or a convex growth function, and provide an equivalent definition. We then characterize 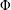$\unicode[STIX]{x1D6F7}$-Carleson measures for Bergman–Orlicz spaces and use them to characterize multipliers between Bergman–Orlicz spaces.

Keywords

Bergman–Orlicz spacesCarleson measuresmultipliers

MSC classification

Primary: 32A36: Bergman spaces 46E30: Spaces of measurable functions ($L^p$-spaces, Orlicz spaces, Köthe function spaces, Lorentz spaces, rearrangement invariant spaces, ideal spaces, etc.)
Secondary: 32A35: $H^p$-spaces, Nevanlinna spaces
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 104 , Issue 1 , February 2018 , pp. 63 - 79
Copyright
© 2017 Australian Mathematical Publishing Association Inc. 

References

Attele, K. R. M., ‘Analytic multipliers of Bergman spaces’, Michigan Math. J. 31 (1984), 307–319.Google Scholar
Axler, S., ‘Multiplication operators on Bergman spaces’, J. reine angew. Math. 336 (1982), 26–44.Google Scholar
Axler, S., ‘Zero-multipliers of Bergman spaces’, Canad. Math. Bull. 28 (1985), 237–242.CrossRefGoogle Scholar
Carleson, L., ‘An interpolation problem for bounded analytic functions’, Amer. J. Math. 80 (1958), 921–930.CrossRefGoogle Scholar
Carleson, L., ‘Interpolation by bounded analytic functions and corona problem’, Ann. of Math. (2) 76 (1962), 547–559.CrossRefGoogle Scholar
Charpentier, S., ‘Composition operators on weighted Bergman–Orlicz spaces on the ball’, Complex Anal. Oper. Theory 7(1) (2013), 43–68.Google Scholar
Charpentier, S. and Sehba, B. F., ‘Carleson measure theorems for large Hardy–Orlicz and Bergman–Orlicz spaces’, J. Funct. Spaces Appl. 2012 (2012), 792763, 21 pages.Google Scholar
Cima, J. A. and Wogen, W., ‘A Carleson measure theorem for the Bergman space on the unit ball of ℂ n ’, J. Operator Theory 7(1) (1982), 157–165.Google Scholar
Duren, P. L., ‘Extension of a theorem of Carleson’, Bull. Amer. Math. Soc. (N.S.) 75 (1969), 143–146.Google Scholar
Hastings, W., ‘A Carleson measure theorem for Bergman spaces’, Proc. Amer. Math. Soc. 52 (1975), 237–241.Google Scholar
Hörmander, L., ‘ L p -estimates for (pluri)subharmonic functions’, Math. Scand. 20 (1967), 65–78.CrossRefGoogle Scholar
Luecking, D., ‘A technique for characterizing Carleson measures on Bergman spaces’, Proc. Amer. Math. Soc. 87 (1983), 656–660.Google Scholar
Luecking, D., ‘Forward and reverse Carleson inequalities for functions in Bergman spaces and their derivatives’, Amer. J. Math. 107(1) (1985), 85–111.Google Scholar
Luecking, D., ‘Multipliers of Bergman spaces into Lebesgue spaces’, Proc. Edingb. Math. Soc. (2) 29 (1986), 125–131.Google Scholar
Luecking, D., ‘Embedding theorems for spaces of analytic functions via Khinchine’s inequality’, Michigan Math. J. 40(2) (1993), 333–358.Google Scholar
Power, S. C., ‘Hörmander’s Carleson theorem for the ball’, Glasg. Math. J. 26(1) (1985), 13–17.Google Scholar
Sehba, B. F. and Stevic, S., ‘On some product-type operators from Hardy–Orlicz and Bergman–Orlicz spaces to weighted-type spaces’, Appl. Math. Comput. 233 (2014), 565–581.Google Scholar
Sehba, B. F. and Tchoundja, E., ‘Hankel operators on holomorphic Hardy–Orlicz spaces’, Integral Equations Operator Theory 73(3) (2012), 331–349.Google Scholar
Stoll, M., Invariant Potential Theory in the Unit Ball of ℂ n (Cambridge University Press, Cambridge, 1994).Google Scholar
Ueki, S., ‘Weighted composition operators between weighted Bergman spaces in the unit ball of ℂ n ’, Nihonkai Math. J. 16(1) (2005), 31–48.Google Scholar
Videnskii, I. V., ‘On an analogue of Carleson measures’, Dokl. Akad. Nauk SSSR 298 (1988), 1042–1047; Soviet Math. Dokl. 37 (1988), 186–190.Google Scholar
Vukotić, D., ‘Pointwise multiplication operators between Bergman spaces on simply connected domains’, Indiana Univ. Math. J. 48 (1999), 793–803.CrossRefGoogle Scholar
Zhao, R., ‘Pointwise multipliers from weighted Bergman spaces and Hardy spaces to weighted Bergman spaces’, Ann. Acad. Sci. Fenn. Math. 29 (2004), 139–150.Google Scholar
Zhao, R. and Zhu, K., ‘Theory of Bergman spaces in the unit ball of ℂ n ’, Mém. Soc. Math. Fr. (N.S.) 115 (2008), 103 pages.Google Scholar