Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-24T13:00:27.691Z Has data issue: false hasContentIssue false
  • English
  • Français

The Lp-Lp mapping properties of convolution operators with the affine arclength measure on space curves

Part of: Harmonic analysis in several variables

Published online by Cambridge University Press:  09 April 2009

Youngwoo Choi
Youngwoo Choi
Affiliation:
Department of Mathematics Ajou UniversitySuwon 442-749Korea e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The Lp-improving properties of convolution operators with measures supported on space curves have been studied by various authors. If the underlying curve is non-degenerate, the convolution with the (Euclidean) arclength measure is a bounded operator from L3/2()3 into L2(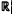3). Drury suggested that in case the underlying curve has degeneracies the appropriate measure to consider should be the affine arclength measure and the obtained a similar result for homogeneous curves t→(t, t2, tk), t >0 for k ≥ 4. This was further generalized by Pan to curves t → (t, tk, tt), t > 0 for l < k < l, k+l ≥ 5. In this article, we will extend Pan's result to (smooth) compact curves of finite type whose tangents never vanish. In addition, we give an example of a flat curve with the same mapping properties.

Keywords

convolutionaffine arclength measureflat curve

MSC classification

Secondary: 42B15: Multipliers 42B10: Fourier and Fourier-Stieltjes transforms and other transforms of Fourier type
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 75 , Issue 2 , October 2003 , pp. 247 - 262
Copyright
Copyright © Australian Mathematical Society 2003

References

[1]Choi, Y., ‘Convolution operators with the affine arclength measure on plane curves’, J. Korean Math. Soc. 36 (1999), 193–207.Google Scholar
[2]Christ, M., ‘Endpoint bounds for singular fractional integral operators’, Preprint, 1988.Google Scholar
[3]Drury, S. W., ‘Degenerate curves and harmonic analysis’, Math. Proc. Cambridge Philos. Soc. 108 (1990), 89–96.CrossRefGoogle Scholar
[4]Littman, W., ‘L p-L q-estimates for singular integral operators arising from hyperbolic equations’, in: Proc. Sympos. Pure Math. XXIII (Amer. Math. Soc., Providence, RI, 1973) pp. 479–481.Google Scholar
[5]Oberlin, D., ‘Convolution estimates for some measures on curves’, Proc. Amer. Math. Soc. 99 (1987), 56–60.CrossRefGoogle Scholar
[6]Pan, Y., ‘A remark on convolution with measures supported on curves’, Canad. Math. Bull. 36 (1993), 245–250.CrossRefGoogle Scholar
[7]Pan, Y., ‘Convolution estimates for some degenerate curves’, Math. Proc. Cambridge Philos. Soc. 116 (1994), 143–146.CrossRefGoogle Scholar
[8]Pan, Y., ‘L p-improving properties for some measures supported on curves’, Math. Scand. 78 (1996), 121–132.CrossRefGoogle Scholar
[9]Ricci, F. and Stein, E. M., ‘Harmonic analysis on nilpotent groups and singular integrals. III. Fractional integration along manifolds’, J. Funct. Anal. 86 (1989), 360–389.CrossRefGoogle Scholar
[10]Secco, S., ‘Fractional integration along homogeneous curves in 3’, Math. Scand. 85 (1999), 259–270.CrossRefGoogle Scholar
[11]Stein, E. M., Singular integrals and differentiability properties of functions (Princeton University Press, Princeton, NJ, 1970).Google Scholar
[12]Stein, E. M., Harmonic analysis: real-variable methods, orthogonality, and oscillatory integrals (Princeton University Press, Princeton, NJ, 1993).Google Scholar
[13]Stein, E. M. and Weiss, G., Introduction to Fourier analysis in Euclidean spaces (Princeton University Press, Princeton, NJ, 1971).Google Scholar
[14]Tao, T. and Wright, J., ‘L p improving bounds for averages along curves’, J. Amer. Math. Soc. 16 (2003), 605–638.CrossRefGoogle Scholar
[15]Zygmund, A., Trigonometric series. Vols. I, II, 2nd Edition (Cambridge University Press, New York, NY, 1959).Google Scholar