Article contents
Locally defined Fitting classes
Published online by Cambridge University Press: 09 April 2009
Extract
Fitting classes of finite solvable groups were first considered by Fischer, who with Gäschutz and Hartley (1967) showed in that in each finite solvable group there is a unique conjugacy class of “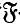 -injectors”, for
-injectors”, for 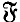 a Fitting class. In general the behaviour of Fitting classes and injectors seems somewhat mysterious and hard to determine. This is in contrast to the situation for saturated formations and
a Fitting class. In general the behaviour of Fitting classes and injectors seems somewhat mysterious and hard to determine. This is in contrast to the situation for saturated formations and 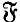 -projectors of finite solvable groups which, because of the equivalence saturated formations and locally defined formations, can be studied in a much more detailed way. However for those Fitting classes
-projectors of finite solvable groups which, because of the equivalence saturated formations and locally defined formations, can be studied in a much more detailed way. However for those Fitting classes 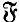 that are “locally defined” the theory of
that are “locally defined” the theory of 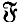 -injectors can be made more explicit by considering various centralizers involving the local definition of
-injectors can be made more explicit by considering various centralizers involving the local definition of 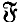 , giving results analogous to some of those concerning locally defined formations. Particular attention will be given to the subgroup B(
, giving results analogous to some of those concerning locally defined formations. Particular attention will be given to the subgroup B(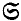 ) defined by
) defined by 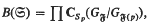 where the set {
where the set {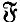 (p)} of Fitting classes locally defines
(p)} of Fitting classes locally defines 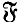 , and the Sp are the Sylow p-subgroups associated with a given Sylow system
, and the Sp are the Sylow p-subgroups associated with a given Sylow system 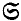 − B(
− B(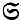 ) plays a role very much like that of Graddon's
) plays a role very much like that of Graddon's 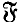 -reducer in Graddon (1971). An
-reducer in Graddon (1971). An  -injector of B(
-injector of B(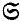 ) is an
) is an 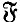 -injector of G, and for certain simple
-injector of G, and for certain simple  B(
B(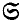 ) is an
) is an 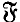 -injector of G.
-injector of G.
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 1975
References
- 12
- Cited by


