A linear mapping D of the algebra of polynomial functions P[0, 1] into the algebra of all continuous complex-valued functions C[0,1] is called a derivation provided D(fg) = fD(g) + gD(f) for all polynomials f and g. The derivations of P[0, 1] into C[0,1] are easily seen to be all mappings of the form Dw where w is a continuous function on [0, 1] and Dw (f) = wf' (f' denotes the ordinary derivative of f). In fact, w = D(x) where x is the coordinate function. Let Dw be such a derivation, and let ∥ · ∥ denote the supremum norm on C[0,1]. Then Dw gives rise to an algebra norm ∥ · ∥w on P[0,1] denned by 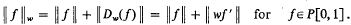.