Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-22T17:48:02.303Z Has data issue: false hasContentIssue false
  • English
  • Français

The proof-theoretic analysis of transfinitely iterated quasi least fixed points

Published online by Cambridge University Press:  12 March 2014

Dieter Probst
Dieter Probst*
Affiliation:
Universität Bern, Institut Für Informatik Und Angewandte Mathematik Neubrückstrasse 10, CH-3012 Bern, Switzerland.E-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The starting point of this article is an old question asked by Feferman in his paper on Hancock's conjecture [6] about the strength of . This theory is obtained from the well-known theory ID1 by restricting fixed point induction to formulas that contain fixed point constants only positively. The techniques used to perform the proof-theoretic analysis of also permit to analyze its transfinitely iterated variants 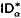. Thus, we eventually know that

Keywords

Fixed pointsIterationPseudo-hierarchies
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 71 , Issue 3 , September 2006 , pp. 721 - 746
Copyright
Copyright © Association for Symbolic Logic 2006

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Aczel, Peter, The strength of Martin-Löf's type theory with one universe, Technical report, Department of Philosophy, University of Helsinki, 1977.Google Scholar
[2]Avigad, Jeremy, On the relationship between ATR0 and , this Journal, vol. 61 (1996), no. 3, pp. 768–779.Google Scholar
[3]Buchholz, Wilfried, Feferman, Solomon, Pohlers, Wolfram, and Sieg, Wilfried, Iterated inductive definitions and subsystems of analysis: Recent proof-theoretical studies, Lecture Notes in Mathematics, vol. 897, Springer, 1981.Google Scholar
[4]Cantini, Andrea, A note on a predicatively reducible theory of iterated elementary induction, Bollettino Unione Mathematica Italiana, vol. 4-B (1985), no. 6, pp. 413–430.Google Scholar
[5]Cantini, Andrea, On the relationship between choice and comprehension principles in second order arithmetic, this Journal, vol. 51 (1986), pp. 360–373.Google Scholar
[6]Feferman, Solomon, Iterated inductive fixed-point theories: Application to Hancock's conjecture, The Patras Symposion (Metakides, G., editor), North Holland, 1982, pp. 171–196.CrossRefGoogle Scholar
[7]Feferman, Solomon, Reflecting on incompleteness, this Journal, vol. 56 (1991), no. 1, pp. 1–49.Google Scholar
[8]Friedman, Harvey, Theories of inductive definition, 1969, Unpublished notes.Google Scholar
[9]Jäger, Gerhard, Kahle, Reinhard, Setzer, Anton, and Strahm, Thomas, The proof-theoretic analysis of transfinitely iterated fixed point theories, this Journal, vol. 64 (1999), no. 1, pp. 53–67.Google Scholar
[10]Jäger, Gerhard and Probst, Dieter, Variation on a theme of Schütte, Mathematical Logic Quarterly, vol. 50 (2004), no. 3, pp. 258–264.CrossRefGoogle Scholar
[11]Kreisel, G., Mathematical Logic, Lectures on modern mathematics (Saaty, Thomas Lorie, editor), vol. III, Wiley, 1965, pp. 95–195.Google Scholar
[12]Pohlers, Wolfram, Proof Theory: An Introduction, Lecture Notes in Mathematics, vol. 1407, Springer, 1989.CrossRefGoogle Scholar
[13]Probst, Dieter, On the relationship between fixed points and iteration in admissible set theory without foundation, Archive for Mathematical Logic, (2005), no. 44, pp. 561–580.Google Scholar
[14]Probst, Dieter, Pseudo-hierarchies in admissible set theories without foundation and explicit mathematics, Ph.D. thesis, Universität Bern, 2005.Google Scholar
[15]Rüede, Christian, The proof-theoretic analysis of transfinite dependent choice, Annals of Pure and Applied Logic, vol. 121 (2003), no. 1, pp. 195–234.CrossRefGoogle Scholar
[16]Schütte, Kurt, Proof Theory, Springer, 1977.CrossRefGoogle Scholar
[17]Schwichtenberg, Helmut, Proof theory: Some applications of cut-elimination, Handbook of Mathematical Logic (Barwise, J., editor), North Holland, 1977, pp. 867–895.CrossRefGoogle Scholar
[18]Simpson, Stephen G., Subsystems of Second Order Arithmetic, Perspectives in Mathematical Logic, Springer, 1998.Google Scholar