Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-22T20:48:19.901Z Has data issue: false hasContentIssue false
  • English
  • Français

P versus NP and computability theoretic constructions in complexity theory over algebraic structures

Published online by Cambridge University Press:  12 March 2014

Gunther Mainhardt
Gunther Mainhardt*
Affiliation:
Universität Greifswald, F.-L.-Jahnstr. 15A, 17487 Greifswald, Germany, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that there is a structure of countably infinite signature with P = N2P and a structure of finite signature with P = N1P and N1P ≠ N2P. We give a further example of a structure of finite signature with P ≠ N1P and N1P ≠ N1P. Together with a result from [10] this implies that for each possibility of P versus NP over structures there is an example of countably infinite signature. Then we show that for some finite 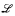the class of 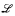-structures with P = N1P is not closed under ultraproducts and obtain as corollaries that this class is not ∆-elementary and that the class of 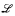-structures with P ≠ N1P is not elementary. Finally we prove that for all ƒ dominating all polynomials there is a structure of finite signature with the following properties: P ≠ N1P, N1P ≠ N2P, the levels N2TIME(ni) of N2P and the levels N1TIME(ni) of N1P are different for different i, indeed DTIME(ni′) ⊈ N2TIME(ni) if i ′ > i; DTIME(ƒ) ⊈ N2P, and N2P ⊈ DEC. DEC is the class of recognizable sets with recognizable complements. So this is an example where the internal structure of N2P is analyzed in a more detailed way. In our proofs we use methods in the style of classical computability theory to construct structures except for one use of ultraproducts.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 69 , Issue 1 , March 2004 , pp. 39 - 64
Copyright
Copyright © Association for Symbolic Logic 2004

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Baker, T., Gill, J., and Solovay, R.. Relativizations of the P = NP question, SIAM Journal on Computing, vol. 4 (1975), pp. 431–442.CrossRefGoogle Scholar
[2]Balcazar, J. L., Diaz, J., and Gabarro, J., Structural complexity 1, 2, Springer, Berlin et al., 1988, 1990.CrossRefGoogle Scholar
[3]Bell, J. L. and Slomson, A. B., Models and ultraproducts, North Holland, 1969.Google Scholar
[4]Chang, C. C. and Keisler, H. J., Model theory, North Holland, 1990.Google Scholar
[5]Ebbinghaus, H. D., Flum, J., and Thomas, W., Mathematical logic, Springer, 1984.Google Scholar
[6]Hemmerling, A., Computability and complexity over structures of finite type, Preprint, 1995, Universität Greifswald, Reihe Mathematik, Nr. 2.Google Scholar
[7]Hemmerling, A., Computability of string functions over algebraic structures, Mathematical Logic Quarterly, vol. 44 (1998), no. 1, pp. 1–44.Google Scholar
[8]Hemmerling, A., Computability over structures of infinite signature, Mathematical Logic Quarterly, vol. 44 (1998), no. 3, pp. 394–416.Google Scholar
[9]Hemmerling, A., On P versus NP for parameter-free programs over algebraic structures, Mathematical Logic Quarterly, vol. 47 (2001), no. 1, pp. 67–92.Google Scholar
[10]Koiran, P., Computing over the reals with addition and order, Theoretical Computer Science, vol. 133 (1994), no. 1, pp. 35–48.Google Scholar
[11]Mainhardt, G., On structures with proper polynomial time hierarchy, Preprint, 2002, Reihe Mathematik, Universität Greifswald, http://sun-10.math-inf.uni-greifswald.de.Google Scholar
[12]Prunescu, M., A model-theoretic proof for P ≠ NP over all infinite abelian groups, this Journal, vol. 67 (2002), no. 1, pp. 235–238.Google Scholar
[13]Soare, R. I., Recursively enumerable sets and degrees, Springer, 1987.CrossRefGoogle Scholar