Hostname: page-component-745bb68f8f-b6zl4 Total loading time: 0 Render date: 2025-01-08T08:57:56.630Z Has data issue: false hasContentIssue false
  • English
  • Français

A new proof of a theorem of Shelah

Published online by Cambridge University Press:  12 March 2014

John W. Rosenthal
John W. Rosenthal*
Affiliation:
State University of New York, Stony Brook, Long Island, New York 11790
Get access Rights & Permissions [Opens in a new window]

Extract

In [10, §0, E), 5)] Shelah states using the proofs of 7.9 and 6.9 in [9] it is possible to prove that if a countable first-order theory T is ℵ0-stable (totally transcendental) and not ℵ1-categorical, then it has at least ∣1 + α∣ models of power ℵα.

In this note we will give a new proof of this theorem using the work of Baldwin and Lachlan [1]. Our original proof used the generalized continuum hypothesis (GCH). We are indebted to G. E. Sacks for suggesting that the notions of ℵ0-stability and ℵ1-categoricity are absolute, and that consequently our use of GCH was eliminable [8]. Routine results from model theory may be found, e.g. in [2].

Proof (with GCH). In the proof of Theorem 3 of [1] Baldwin and Lachlin show 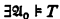of power ℵα such that there is a countable definable subset in 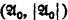. Let B0 be such a subset. Say 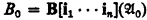. We will give by transfinite induction an elementary chain 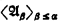of models of T of power ℵα such that B[i1 … in] 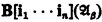has power ℵβ and such that every infinite definable subset of 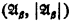has power ≥ℵβ. This clearly suffices.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 37 , Issue 1 , March 1972 , pp. 133 - 134
Copyright
Copyright © Association for Symbolic Logic 1972

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Baldwin, J. T. and Lachlan, A. H., On strongly minimal sets, this Journal vol. 36 (1971), pp. 79–96.Google Scholar
[2]Bell, J. L. and Slomson, A. B., Models and ultraproducts, North-Holland, Amsterdam, 1969.Google Scholar
[3]Keisler, H. J., Good ideals infields of sets, Annals of Mathematics, vol. 7 (1964), pp. 338–359.CrossRefGoogle Scholar
[4]Keisler, H. J., Ultraproducts and saturated models, Indagationes Matkematicae, vol. 26 (1964), pp. 178–186.CrossRefGoogle Scholar
[5]Morley, M., Categoricity in power, Transactions of the American Mathematical Society, vol. 114 (1965), pp. 514–538.CrossRefGoogle Scholar
[6]Morley, M. and Vaught, R. L., Homogeneous universal models, Mathematica Scandinavica, vol. 11 (1962), pp. 37–57.CrossRefGoogle Scholar
[7]Mostowski, A., Constructible sets with applications, North-Holland, Amsterdam, 1969.Google Scholar
[8]Sacks, G. E., Saturated model theory, Benjamin, New York, 1972.Google Scholar
[9]Shelah, S., Finite diagrams stable in power, Annals of Mathematical Logic, vol. 2 (1970), pp. 69–118.CrossRefGoogle Scholar
[10]Shelah, S., Stability, the F.c.p. and super stability; model theoretic properties of formulas in first order theory (to appear).Google Scholar