Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-22T20:08:02.505Z Has data issue: false hasContentIssue false
  • English
  • Français

Limit ultrapowers and abstract logics

Published online by Cambridge University Press:  12 March 2014

Paolo Lipparini
Paolo Lipparini*
Affiliation:
Department of Mathematics, University of Tor Vergata, Rome, Italy
Get access Rights & Permissions [Opens in a new window]

Abstract

We associate with any abstract logic L a family F(L) consisting, intuitively, of the limit ultrapowers which are complete extensions in the sense of L.

For every countably generated [ω, ω]-compact logic L, our main applications are:

(i) Elementary classes of L can be characterized in terms of ≡L only.

(ii) If 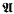and 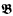are countable models of a countable superstable theory without the finite cover property, then .

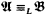(iii) There exists the “largest” logic M such that complete extensions in the sense of M and L are the same; moreover M is still [ω, ω]-compact and satisfies an interpolation property stronger than unrelativized ⊿-closure.

(iv) If L = Lωω(Qx), then cf(ωx) > ω and λω < ωx, for all λ < ωx.

We also prove that no proper extension of Lωω generated by monadic quantifiers is compact. This strengthens a theorem of Makowsky and Shelah. We solve a problem of Makowsky concerning Lκλ-compact cardinals. We partially solve a problem of Makowsky and Shelah concerning the union of compact logics.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 52 , Issue 2 , June 1987 , pp. 437 - 454
Copyright
Copyright © Association for Symbolic Logic 1987

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[BF]Barwise, J. and Feferman, S. (editors), Model-theoretic logics, Perspectives in Mathematical Logic, vol. 6, Springer-Verlag, Berlin, 1985.Google Scholar
[Ca]Caicedo, X., Failure of interpolation for quantifiers of monadic type, Methods in mathematical logic (proceedings of the sixth Latin American symposium in mathematical logic; Di Prisco, C. A., editor), Lecture Notes in Mathematics, Vol. 1130, Springer-Verlag, Berlin, 1985, pp. 1–12.Google Scholar
[CK]Chang, C. C. and Keisler, J., Model theory, 2nd ed., Studies in Logic and the Foundations of Mathematics, vol. 73, North-Holland, Amsterdam, 1977.Google Scholar
[Eb]Ebbinghaus, H. D., Extended logics: the general framework, in [BF], pp. 25–76.CrossRefGoogle Scholar
[Ke]Keisler, J., On cardinalities of ultraproducts, Bulletin of the American Mathematical Society, Vol. 70 (1964), pp. 644–647.CrossRefGoogle Scholar
[Ko]Kopperman, R., Model theory and its applications, Allyn and Bacon, Boston, Massachusetts, 1972.Google Scholar
[Li]Lindström, P., On extensions of elementary logic, Theoria, vol. 35 (1969), pp. 1–11.CrossRefGoogle Scholar
[Lp1]Lipparini, P., Duality for compact logics and substitution in abstract model theory, Zeitschrift für Mathematische Logik und Grundlagen der Mathematik, vol. 31 (1985), pp. 517–532.CrossRefGoogle Scholar
[Lp2]Lipparini, P., Logics closed under first order unary operations (abstract), this Journal, vol. 51 (1986), p. 491.Google Scholar
[Lp3]Lipparini, P., Robinson equivalence relations through limit ultrapowers, Bollettino dell'Unione Matematica Italiana, vol. 4-B (1985), pp. 569–583.Google Scholar
[Ma]Makowsky, J. A., Vopěnka's principle and compact logics, this Journal, vol. 50 (1985), pp. 42–48.Google Scholar
[MS1]Makowsky, J. A. and Shelah, S., The theorems of Beth and Craig in abstract model theory. I: The abstract setting, Transactions of the American Mathematical Society, vol. 256 (1979), pp. 215–239.Google Scholar
[MS2]Makowsky, J. A. and Shelah, S., Positive results in abstract model theory: a theory of compact logics, Annals of Pure and Applied Logic, vol. 25 (1983), pp. 263–299.CrossRefGoogle Scholar
[Mu1]Mundici, D., Interpolation, compactness and JEP in soft model theory, Archiv für Mathematische Logik und Grundlagenforschung, vol. 22 (1982), pp. 61–67.CrossRefGoogle Scholar
[Mu2]Mundici, D., Duality between logics and equivalence relations, Transactions of the American Mathematical Society, vol. 270 (1982), pp. 111–129.CrossRefGoogle Scholar
[Sh]Shelah, S., Classification theory and the number of nonisomorphic models, Studies in Logic and the Foundations of Mathematics, vol. 92, North Holland, Amsterdam, 1978.Google Scholar