No CrossRef data available.
Published online by Cambridge University Press: 12 March 2014
With the definition of effective operations as stated in the paper cited above (this Journal, vol. 35 (1970), p. 217), the last step of Lemma 1 does not appear to extend to types ≥ 3. This may be rectified by altering the second clause of the definition of effective operation (loc. cit. p. 217) to read:
ℱ is an effective operation of type i + 2 with Gödel number f∈E i+2, if for every g∈E i+1 there is an effective operation  of type i + 1 (not depending on ℱ and f) with Gödel number g such that ℱ(
of type i + 1 (not depending on ℱ and f) with Gödel number g such that ℱ( ) = p(f, g).
) = p(f, g).
With this definition the proofs go through without alteration.
There are two other variants for representing an effective operation by higher type objects.
I. We adopt the definition of effective as given in the text, and require the terms 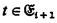 to satisfy the additional condition
to satisfy the additional condition
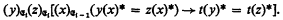
II. Here we add to the definition of effective operations given in the text the condition:
If ℱ and ℱ1 are effective operations of type i + 2 and  for all effective operations
for all effective operations  of type i + 1, then ℱ = ℱ1.
of type i + 1, then ℱ = ℱ1.
We now require terms s, t ∈ fi to satisfy the additional condition
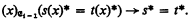
The proof of Lemmas 1 and 2 are easily modified to establish I and II.
Perhaps II may be regarded as giving the most natural characterisation since in this case all terms represent continuous functionals.