Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-26T20:41:59.837Z Has data issue: false hasContentIssue false
  • English
  • Français

Actions by the classical Banach spaces

Published online by Cambridge University Press:  12 March 2014

G. Hjorth
G. Hjorth*
Affiliation:
Department of Mathematics, University of California, Los Angeles, Los Angeles, California 90095-1555, USA, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

The study of continuous group actions is ubiquitous in mathematics, and perhaps the most general kinds of actions for which we can hope to prove theorems in just ZFC are those where a Polish group acts on a Polish space.

For this general class we can find works such as [29] that build on ideas from ergodic theory and examine actions of locally compact groups in both the measure theoretic and topological contexts. On the other hand a text in model theory, such as [12], will typically consider issues bearing on the actions by the symmetric group of all permutations of the integers. More generally [1] shows that the orbit equivalence relations induced by closed subgroups of the infinite symmetric group can be reduced to the isomorphism relation on corresponding classes of countable models.

This paper considers a third category formed by the continuous actions of separable Banach spaces on Polish spaces. These examples cannot be subsumed under the two earlier headings, and it is known from [10] that the Borel cardinalities of the quotient spaces that arise from such actions are incomparable with the equivalence relations induced by the symmetric group or any locally compact Polish group action.

One of the first things to be addressed concerns the complexity of these equivalence relations. This question for 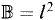appears in [1].

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 65 , Issue 1 , March 2000 , pp. 392 - 420
Copyright
Copyright © Association for Symbolic Logic 2000

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Becker, H. and Kechris, A. S., The descriptive set theory of Polish group actions, to appear in the London Mathematical Society Lecture Notes Series.CrossRefGoogle Scholar
[2]Dougherty, R. and Hjorth, G., Reducibility and nonreducibility between lp equivalence relations, to appear in the Transactions of the American Mathematical Society.Google Scholar
[3]Effros, E., Transformation groups and C*-algebras, Annals of Mathematics, vol. 81 (1975), pp. 38–55.CrossRefGoogle Scholar
[4]Farah, I., Basis problem for turbulent actions, preprint, York University, Toronto, 1998.Google Scholar
[5]Feldman, J., Hahn, P., and Moore, C. C., Orbit structure and countable sections for actions of countable groups, Advances in Mathematics, vol. 28 (1978), pp. 186–230.CrossRefGoogle Scholar
[6]Harrington, L. A., A powerless proof of a theorem by Silver, handwritten note, UC Berkeley, 1976.Google Scholar
[7]Harrington, L. A., Kechris, A. S., and Louveau, A., A Glimm-Effros dichotomy for Borel equivalence relations, Journal of the American Mathematical Society, vol. 3 (1990), pp. 903–928.CrossRefGoogle Scholar
[8]Harrington, L. A., Marker, D., and Shelah, S., Borel orderings, Transactions of the American Mathematical Society, vol. 310 (1988), pp. 292–302.CrossRefGoogle Scholar
[9]Hjorth, G., An absoluteness principle for Borel sets, preprint, Caltech, 1996.Google Scholar
[10]Hjorth, G., Classification and orbit equivalence relations, manuscript, UCLA, 1997.Google Scholar
[11]Hjorth, G., Kechris, A. S., and Louveau, A., Borel equivalence relations induced by actions of the symmetric group, preprint, Caltech, 1996.Google Scholar
[12]Hodges, W., Model theory, Cambridge University Press, Cambridge, 1993.CrossRefGoogle Scholar
[13]Jackson, S., Kechris, A. S., and Louveau, A., On countable Borel equivalence relations, preprint, Caltech, 1994.Google Scholar
[14]Jech, T., Set theory, Academic Press, New York, 1978.Google Scholar
[15]Kechris, A. S., Definable equivalence relations and Polish group actions, unpublished manuscript.Google Scholar
[16]Kechris, A. S., Countable ordinals and the analytic hierarchy, II, Annals of Mathematical Logic, vol. 15 (1978), pp. 193–223.CrossRefGoogle Scholar
[17]Kechris, A. S., The structure of Borel equivalence relations in Polish spaces, Set theory of the continuum (Judah, H., Just, W., and Woodin, W. H., editors), MSRI Publication, no. 26, Springer-Verlag, New York, 1990, pp. 75–84.Google Scholar
[18]Kechris, A. S., Countable sections for locally compact group actions, Journal of Ergodic Theory and Dynamical Systems, vol. 12 (1992), pp. 283–295.CrossRefGoogle Scholar
[19]Kechris, A. S., Classical descriptive set theory, Graduate Texts in Mathematics, Springer-Verlag, Berlin, 1995.CrossRefGoogle Scholar
[20]Kechris, A. S., Notes on turbulence, typewritten notes, Caltech, 1996.Google Scholar
[21]Kechris, A. S., Rigidity properties of Borel ideals on the integers, in the 8th Prague Topological Symposium on general topology and its relations to modern analysis and algebra (1996), Topology and its Applications, vol. 85 (1998), pp. 195–205.CrossRefGoogle Scholar
[22]Kechris, A. S. and Louveau, A., The classification of hypersmooth Borel equivalence relations, Journal of the American Mathematical Society, vol. 10 (1997), pp. 215–242.CrossRefGoogle Scholar
[23]Louveau, A. and Velickovic, B., A note on Borel equivalence relations, Proceedings of the American Mathematical Society, vol. 120 (1994), pp. 255–259.CrossRefGoogle Scholar
[24]Moschovakis, Y. N., Descriptive set theory, North-Holland, Amsterdam, 1980.Google Scholar
[25]Silver, J., Counting the number of equivalence classes of Borel and co-analytic equivalence relation, Annals of Mathematical Logic, vol. 18 (1980), pp. 1–28.CrossRefGoogle Scholar
[26]Solecki, S., dissertation, Caltech, 1995.Google Scholar
[27]Solecki, S., Analytic ideals, Bulletin of Symbolic Logic, vol. 2 (1996), pp. 339–348.CrossRefGoogle Scholar
[28]Solecki, S., Actions of non-compact and non-locally compact Polish groups, preprint, University of Indiana, Bloomington, 1998.Google Scholar
[29]Zimmer, R., Ergodic theory and semi-simple groups, BirkhÄuser, Basel, 1984.CrossRefGoogle Scholar