Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-26T10:55:52.901Z Has data issue: false hasContentIssue false
  • English
  • Français

Strongly representable atom structures of cylindric algebras

Published online by Cambridge University Press:  12 March 2014

Robin Hirsch  and
Ian Hodkinson
Robin Hirsch
Affiliation:
Department of Computer Science, University College London, London, WC1E 6BT, U.K., E-mail: [email protected], URL: http://www.cs.ucl.ac.Uk/staff/R.Hirsch/
Ian Hodkinson
Affiliation:
Department of Computing, Imperial College London, London, Sw7 2Az, U.K., E-mail: [email protected], URL: http://www.doc.ic.ac.uk/~imh
Get access Rights & Permissions [Opens in a new window]

Abstract

A cylindric algebra atom structure is said to be strongly representable if all atomic cylindric algebras with that atom structure are representable. This is equivalent to saying that the full complex algebra of the atom structure is a representable cylindric algebra. We show that for any finite n ≥ 3, the class of all strongly representable n-dimensional cylindric algebra atom structures is not closed under ultraproducts and is therefore not elementary.

Our proof is based on the following construction. From an arbitrary undirected, loop-free graph Γ, we construct an n-dimensional atom structure 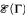, and prove, for infinite Γ, that 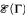is a strongly representable cylindric algebra atom structure if and only if the chromatic number of Γ is infinite. A construction of Erdős shows that there are graphs Γk(k < ω) with infinite chromatic number, but having a non-principal ultraproduct ΠDΓk whose chromatic number is just two. It follows that is strongly representable (each k < ω) but is not.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 74 , Issue 3 , September 2009 , pp. 811 - 828
Copyright
Copyright © Association for Symbolic Logic 2009

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Andréka, H., Complexity of equations valid in algebras of relations, Part I: Strong non-finitizability, Annals of Pure and Applied Logic, vol. 89 (1997), pp. 149–209.CrossRefGoogle Scholar
[2]Andréka, H., Németi, I., and Ahmed, T. Sayed, Omitting types for finite variable fragments and complete representations of algebras, this Journal, vol. 73 (2008), pp. 65–89.Google Scholar
[3]Chang, C. C. and Keisler, H. J., Model theory, 3rd ed., North-Holland, Amsterdam, 1990.Google Scholar
[4]Diestel, R., Graph theory, Graduate Texts in Mathematics, vol. 173, Springer-Verlag, Berlin. 1997.Google Scholar
[5]Erdős, P., Graph theory and probability, Canadian Journal of Mathematics, vol. 11 (1959), pp. 34–38.CrossRefGoogle Scholar
[6]Goldblatt, R., Varieties of complex algebras, Annals of Pure and Applied Logic, vol. 44 (1989), pp. 173–242.CrossRefGoogle Scholar
[7]Goldblatt, R., Elementary generation and canonicity for varieties of boolean algebras with operators, Algebra Universalis, vol. 34 (1995), pp. 551–607.CrossRefGoogle Scholar
[8]Henkin, L., Monk, J. D., and Tarski, A., Cylindric algebras part I, North-Holland, 1971.Google Scholar
[9]Henkin, L., Monk, J. D., and Tarski, A., Cylindric algebras part II, North-Holland, 1985.Google Scholar
[10]Henkin, L., Monk, J. D., Tarski, A., Andréka, H., and Németi, I., Cylindric set algebras, Lecture Notes in Mathematics, vol. 883, Springer, Berlin, 1981.CrossRefGoogle Scholar
[11]Hirsch, R. and Hodkinson, I., Complete representations in algebraic logic, this Journal, vol. 62 (1997), no. 3, pp. 816–847.Google Scholar
[12]Hirsch, R. and Hodkinson, I., Relation algebras by games, Studies in Logic and the Foundations of Mathematics, vol. 147, North-Holland, Amsterdam, 2002.Google Scholar
[13]Hirsch, R. and Hodkinson, I., Strongly representable atom structures of relation algebras, Proceedings of the American Mathematical Society, vol. 130 (2002), pp. 1819–1831.CrossRefGoogle Scholar
[14]Hodkinson, I., Atom structures of cylindric algebras and relation algebras, Annals of Pure and Applied Logic, vol. 89 (1997), pp. 117–148.CrossRefGoogle Scholar
[15]Hodkinson, I. and Venema, Y., Canonical varieties with no canonical axiomatisation, Transactions of the American Mathematical Society, vol. 357 (2005), pp. 4579–4605.CrossRefGoogle Scholar
[16]Jónsson, B. and Tarski, A., Boolean algebras with operators I, American Journal of Mathematics, vol. 73 (1951), pp. 891–939.CrossRefGoogle Scholar
[17]Monk, J. D., Nonfinitizability of classes of representable cylindric algebras, this Journal, vol. 34 (1969), pp. 331–343.Google Scholar
[18]Monk, J. D., Completions of boolean algebras with operators, Mathematische Nachrichten, vol. 46 (1970), pp. 47–55.CrossRefGoogle Scholar
[19]Monk, J. D., An introduction to cylindric set algebras, Logic Journal of the IGPL, vol. 8 (2000), no. 4, pp. 451–496.CrossRefGoogle Scholar
[20]Ramsey, F. P., On a problem of formal logic, Proceedings of the London Mathematical Society, vol. 30 (1930), pp. 264–286.CrossRefGoogle Scholar
[21]Ahmed, T. Sayed, Martin's axiom, omitting types, and complete representations in algebraic logic, Studia Logica, vol. 72 (2002), no. 2, pp. 285–309.CrossRefGoogle Scholar
[22]Tarski, A., Contributions to the theory of models, III, Koninklijke Nederlandse Akademie van Wetenschappen. Indagationes Mathematicae, vol. 58 (1955), pp. 56–64, (= Indag. Math. 17).Google Scholar
[23]Venema, Y., Atom structures, Advances in modal logic '96 (Kracht, M., de Rijke, M., Wansing, H., and Zakharyaschev, M., editors), CSLI Publications, Stanford, 1997, pp. 291–305.Google Scholar
[24]Venema, Y., Atom structures and Sahlqvist equations, Algebra Universalis, vol. 38 (1997), pp. 185–199.CrossRefGoogle Scholar