Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-26T11:09:02.176Z Has data issue: false hasContentIssue false
  • English
  • Français

A polynomial translation of S4 into intuitionistic logic

Published online by Cambridge University Press:  12 March 2014

David Fernandez
David Fernandez*
Affiliation:
Department of Mathematics, Stanford University, Stanford, California 94305, USA.E-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

It is known that both S4 and the Intuitionistic prepositional calculus Int are P-SPACE complete. This guarantees that there is a polynomial translation from each system into the other.

However, no sound and faithful polynomial translation from S4 into Int is commonly known. The problem of finding one was suggested by Dana Scott during a very informal gathering of logicians in February 2005 at UCLA. Grigori Mints then brought it to my attention, and in this paper I present a solution. It is based on Kripke semantics and describes model-checking for S4 using formulas of Int.

A simple translation from Int into S4, the Gödel-Tarski translation, is wellknown; given a formula φ of Int, one obtains 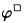by prefixing □ to every subformula. For example,

.

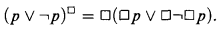That the translation is sound and faithful can be seen by considering topological semantics, which assign open sets both to □-formulas of S4 and arbitrary formulas of Int; the interpretation of φ and 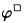turn out to be identical. See Tarski's paper [6] for details. Gödel's original paper can be found in [3].

In [2], Friedman and Flagg present a kind of inverse to Gödel-Tarski. Given a formula φ of S4 and a finite set of formulas Γ of Int, for each ε ∈ Γ one gets an intuitionistic formula 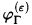given recursively by

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 71 , Issue 3 , September 2006 , pp. 989 - 1001
Copyright
Copyright © Association for Symbolic Logic 2006

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Blackburn, Patrick, De Rijke, Maarten, and Venema, Yde, Modal logic, Cambridge University Press, 2001.CrossRefGoogle Scholar
[2]Friedman, H. and Flagg, R. C., Epistemic and intuitionistic formal systems, Annals of Pure and Applied Logic, vol. 32 (1986), pp. 53–60.Google Scholar
[3]Gödel, Kurt, Collected works (Feferman, Solomon, ed.), Oxford University Press, 1986.Google Scholar
[4]Kripke, Saul, Semantical analysis of intuitionistic logicI, Crossley Formal Systems And Recursive Functions, (1965), pp. 92–130.CrossRefGoogle Scholar
[5]Mints, G. E., A short introduction to intuitionistic logic, Plenum Publishers, 2000.Google Scholar
[6]Tarski, Alfred, Der Aussagenkalkül und die Topologie, Fundamenta Mathematica, vol. 31 (1938), pp. 103–134.CrossRefGoogle Scholar