Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-26T10:13:19.852Z Has data issue: false hasContentIssue false
  • English
  • Français

Microstructure characterization and phase field analysis of dendritic crystal growth of γ-U and BCC-Mo dendrite in U–33 at.% Mo fast reactor fuel

Published online by Cambridge University Press:  09 November 2017

Sibasis Chakraborty ,
Gargi Choudhuri ,
Perepa Subramanya Somayajulu ,
Renu Agarwal  and
Kirity Bhusan Khan
Sibasis Chakraborty*
Affiliation:
Homi Bhabha National Institute, Mumbai-400094, Maharashtra, India; and Radiometallurgy Division, Bhabha Atomic Research Centre, Mumbai-400085, India
Gargi Choudhuri
Affiliation:
Quality Assurance Division, Bhabha Atomic Research Centre, Mumbai-400085, India
Perepa Subramanya Somayajulu
Affiliation:
Radiometallurgy Division, Bhabha Atomic Research Centre, Mumbai-400085, India
Renu Agarwal
Affiliation:
Fuel Chemistry Division, Bhabha Atomic Research Centre, Mumbai-400085, India
Kirity Bhusan Khan
Affiliation:
Radiometallurgy Division, Bhabha Atomic Research Centre, Mumbai-400085, India
*
a)Address all correspondence to this author. e-mail: [email protected]
Get access

Abstract

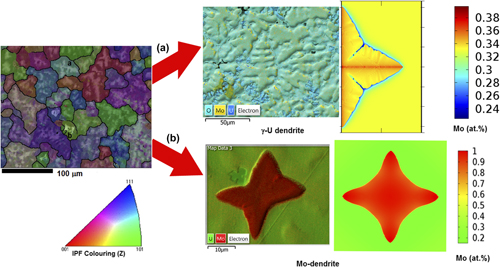
U–Mo metallic alloy is considered as an advanced fast reactor and research reactor fuel material. U–33 at.% Mo has a higher melting point than that of pure uranium metal. This provides a higher safety margin against fuel melting and diminishes fuel and clad interaction. The metallic fuels are fabricated through a melting-casting route, and the cast microstructure of U–33 at.% Mo has been characterized using optical microscope, scanning electron microscopy—energy dispersive spectroscopy, and Electron back scattered diffraction. These microstructures show dendrites of two different morphologies: (i) the γ-(U) dendrite with secondary branches and (ii) the equiaxed (Mo) dendrite without secondary branches and surrounded by a peritectic reaction product. In this article, for the first time, a phase field model has been developed for U–Mo alloys to understand the morphological evolution and the associated microsegregation of γ-(U) dendrites in the U–33 at.% Mo alloy. The evolution of the concentration and temperature field with the time and the effect of undercooling on the growth velocity of γ-(U) and (Mo) dendrites has been studied.

Keywords

fast reactorU–Modendrite
Type
Articles
Information
Journal of Materials Research , Volume 33 , Issue 2 , 29 January 2018 , pp. 225 - 238
Check for updates
Copyright
Copyright © Materials Research Society 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Contributing Editor: Michele Manuel

References

REFERENCES

Meyer, M.K., Gan, J., Jue, J.F., Keisar, D.D., Perez, E., Robinson, A., Wachs, D.M., Woolstenhulme, N., Hofman, G.L., and Kim, Y.S.: Irradiation performance of U–Mo monolithic fuel. Nucl. Eng. Technol. 46(2), 169 (2014).CrossRefGoogle Scholar
Mariani, R.D., Porter, D.L., Blackwood, V.S., Jones, Z.S., Olson, D.L., Mishra, B., Kennedy, J.R., and Hayes, S.L.: International Conference on Fast Reactors and Related Fuel Cyles: Safe Technologies and Sustainable Scenarios (FR13), Paris, France, 4–7 March (International Atomic Energy Agency, Vienna, 2013). IAEA-CN–199/366; ISSN 0074–1884.Google Scholar
Chakraborty, S., Choudhuri, G., Banerjee, J., Agarwal, R., Khan, K.B., and Kumar, A.: Micro-structural study and rietveld analysis of fast reactor fuels: U–Mo fuels. J. Nucl. Mater. 467, 618 (2015).CrossRefGoogle Scholar
Chang, Y.I.: Technical rationale for metal fuel in fast reactors. Nucl. Eng. Technol. 39(3), 161 (2007).CrossRefGoogle Scholar
Mariani, R.D., Porter, D.L., Hayes, S.L., and Kennedy, J.R.: Metallic fuels: The EBR-II legacy and recent advances. Process Chem. 7, 513–520 (2012).CrossRefGoogle Scholar
Landa, A., Soderlind, P., Grabowski, B., Turchi, P.E.A., Ruban, A.V., and Vitos, L.: Ab Initio Study of Advanced Metallic Nuclear Fuels for Fast Breeder Reactors, MRS Spring Meeting, San Francisco, CA, USA, April 9–13 (2012); published in the MRS Proceedings on “Actinides—Basic Science, Applications, and Technology”, 14 pages (LLNL-CONF-552336).Google Scholar
Zhang, X., Cui, Y.F., Xu, G.L., Zhu, W.J., Liu, H.S., Yin, B.Y., and Jin, Z.P.: Thermodynamic assessment of the U–Mo–Al system. J. Nucl. Mater. 402, 15 (2010).CrossRefGoogle Scholar
Velikanova, T., Bodar, A., Artyukh, L., Bilous, O., Firstov, S., and Miracle, D.: Titanium-boride composites: Influence of alloying on constitution and properties of titanium–boride eutectic alloys. In Metallic Materials with High Structural Efficiency, Senkov, O.N., Miracle, D.B., and Fristov, S.A., eds. (Kluwer Academic Publishers, Dordrecht, the Netherlands 2004); p. 260.Google Scholar
Schaffnit, P., Stallybrass, C., Konrad, J., Stein, F., and Weinberg, M.: A Scheil–Gulliver model dedicated to the solidification of steel. Calphad 48, 184 (2015).CrossRefGoogle Scholar
Lavernia, E.J. and Srivatsan, T.S.: The rapid solidification processing of materials: Science, principles, technology, advances, and applications. J. Mater. Sci. 45, 287 (2010).CrossRefGoogle Scholar
Schwarz, M., Arnold, C.B., Aziz, M.J., and Herlach, D.M.: Dendritic growth velocity and diffusive speed in solidification undercooled dilute Ni–Zr melts. Mater. Sci. Eng., A 226–228, 420 (1997).CrossRefGoogle Scholar
McFadden, G.B., Wheeler, A.A., Braun, R.J., Coriell, S.R., and Sekerka, R.F.: Phase-field models for anisotropic interfaces. Phys. Rev. E 48(3), 2016 (1993).CrossRefGoogle ScholarPubMed
Wheeler, A.A., Murry, B.T., and Schaefer, R.J.: Computation of dendrites using a phase field model. Phys. D 66, 243 (1993).CrossRefGoogle Scholar
Wheeler, A.A., Boettinger, W.J., and McFaden, G.B.: Phase-field model of solute trapping during solidification. Phys. Rev. E 47(3), 1893 (1993).CrossRefGoogle ScholarPubMed
Wang, S.L., Sekerka, R.F., Wheeler, A.A., Murry, B.T., Coriell, S.R., Braun, R.J., and McFaden, G.B.: Thermodynamically-consistent phase field models for solidification. Phys. D 69, 189 (1993).CrossRefGoogle Scholar
Boettinger, W.J., Wheeler, A.A., Murry, B.T., and McFadden, G.B.: Prediction of solute trapping at high solidification rates using a diffuse interface phase-field theory of alloy solidification. Mater. Sci. Eng., A 178, 217 (1994).CrossRefGoogle Scholar
Boettinger, W.J. and Warren, J.A.: The phase field method: Simulation of alloy dendritic solidification during recalescence. Metall. Mater. Trans. A 27, 657 (1996).CrossRefGoogle Scholar
Dinsdale, A.T.: SGTE data for pure elements. Calphad 15, 317 (1991).CrossRefGoogle Scholar
Thermophysical Properties of Materials for Nuclear Engineering: A tutorial and collection of data, Chapter: Metallic fuel, Uranium, Kirikov, P.L., ed. (International Atomic Energy Agency, Vienna, 2006); p. 15.Google Scholar
Determining the thermophysical properties of molybdenum, by NETZSCH-Geratebau GmbH, 26th June 2013, on internet site, Available at: www.azom.com/article.aspx?ArticleID=9384 (accessed November 12, 2016).Google Scholar
Chemical engineering division research highlights: May 1962-April 1963, ANL-6766, Research reports, 38.Google Scholar
Rothman, S.J.: Diffusion in Uranium, Its Alloys and Compounds, 1961, ANL-5700, part C.CrossRefGoogle Scholar
Palinov, V., Nakonechnikov, A.I., and Bykov, V.N.: Diffusion of uranium in molybdenum, niobium, zirconium and titanium. At. Energ. 19(6), 521 (1965).Google Scholar
Huang, K., Keiser, D.D. Jr., and Sohn, Y.: Interdiffusion intrinsic diffusion, atomic mobility, and vacancy wind effect in c(bcc) uranium–molybdenum alloy. Metall. Mater. Trans. A 44, 738 (2013).CrossRefGoogle Scholar
Askill, J. and Tomlin, D.H.: Self-diffusion in molybdenum. Philos. Mag. 8(90), 997 (1963).CrossRefGoogle Scholar
Loginova, S. and Singer, H.M.: The phase field technique for modeling multiphase materials. Rep. Prog. Phys. 71, 106501 (2008).CrossRefGoogle Scholar
Hoyt, J.J., Asta, M., and Karma, A.: Atomistic and continuum modeling of dendritic solidification. Mater. Sci. Eng., R 41, 121 (2003).CrossRefGoogle Scholar
Hoyt, J.J., Asta, M., and Karma, A.: Atomistic simulation methods for computing the kinetic coefficient in solid-liquid systems. Interface Sci. 10, 181 (2002).CrossRefGoogle Scholar
Coriell, S.R. and Turnbull, D.: Relative roles of heat transport and interface rearrangement rates in the rapid growth of crystals in undercooled melts. Acta Metall. 30, 2135 (1982).CrossRefGoogle Scholar
Vinet, B., Magnusson, L., Fredriksson, H., and Desre, P.J.: Correlations between surface and interface energies with respect to crystal nucleation. J. Colloid Interface Sci. 255(4), 363 (2002).CrossRefGoogle ScholarPubMed
Glicksman, M.E., Lowengrub, J.S., and Li, S.: Non-monotone temperature boundary conditions in dendritic growth. In Modelling of Casting, Welding and Advanced Solidification Processing XI, Available at: www.math.uci.edu/∼lowengrb/RESEARCH/publications/MCWASP_XI_8MEG.pdf (accessed December 3, 2016).Google Scholar
Glicksman, M.E.: Mechanism of dendritic branching. Metall. Mater. Trans. A 43, 391 (2012).CrossRefGoogle Scholar
Mullis, A.M.: Deterministic side-branching during thermal dendritic growth. Mater. Sci. Eng. 84, 012071 (2015).Google Scholar
Mullins, W. and Sekerka, R.: Stability of a planar interface during solidification of a dilute binary alloy. J. Appl. Phys. 35, 444 (1964).CrossRefGoogle Scholar