In this paper, we use asymptotic theory and numerical methods to study resonant triad interactions among discrete internal wave modes at a fixed frequency (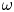 $\omega$) in a two-dimensional, uniformly stratified shear flow. Motivated by linear internal wave generation mechanisms in the ocean, we assume the primary wave field as a linear superposition of various horizontally propagating vertical modes at a fixed frequency
$\omega$) in a two-dimensional, uniformly stratified shear flow. Motivated by linear internal wave generation mechanisms in the ocean, we assume the primary wave field as a linear superposition of various horizontally propagating vertical modes at a fixed frequency 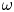 $\omega$. The weakly nonlinear solution associated with the primary wave field is shown to comprise superharmonic (frequency
$\omega$. The weakly nonlinear solution associated with the primary wave field is shown to comprise superharmonic (frequency 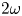 $2\omega$) and zero frequency wave fields, with the focus of this study being on the former. When two interacting primary modes
$2\omega$) and zero frequency wave fields, with the focus of this study being on the former. When two interacting primary modes 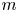 $m$ and
$m$ and  $n$ are in triadic resonance with a superharmonic mode
$n$ are in triadic resonance with a superharmonic mode 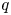 $q$, it results in the divergence of the corresponding superharmonic secondary wave amplitude. For a given modal interaction
$q$, it results in the divergence of the corresponding superharmonic secondary wave amplitude. For a given modal interaction 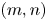 $(m, n)$, the superharmonic wave amplitude is plotted on the plane of primary wave frequency
$(m, n)$, the superharmonic wave amplitude is plotted on the plane of primary wave frequency 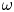 $\omega$ and Richardson number
$\omega$ and Richardson number 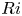 $Ri$, and the locus of divergence locations shows how the resonance locations are influenced by background shear. In the limit of weak background shear (
$Ri$, and the locus of divergence locations shows how the resonance locations are influenced by background shear. In the limit of weak background shear (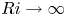 $Ri\to \infty$), using an asymptotic theory, we show that the horizontal wavenumber condition
$Ri\to \infty$), using an asymptotic theory, we show that the horizontal wavenumber condition 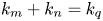 $k_m + k_n = k_q$ is sufficient for triadic resonance, in contrast to the requirement of an additional vertical mode number condition (
$k_m + k_n = k_q$ is sufficient for triadic resonance, in contrast to the requirement of an additional vertical mode number condition (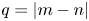 $q = |m-n|$) in the case of no shear. As a result, the number of resonances for an arbitrarily weak shear is significantly larger than that for no shear. The new resonances that occur in the presence of shear include the possibilities of resonance due to self-interaction and resonances that occur at the seemingly trivial limit of
$q = |m-n|$) in the case of no shear. As a result, the number of resonances for an arbitrarily weak shear is significantly larger than that for no shear. The new resonances that occur in the presence of shear include the possibilities of resonance due to self-interaction and resonances that occur at the seemingly trivial limit of 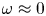 $\omega \approx 0$, both of which are not possible in the no shear limit. Our weak shear limit conclusions are relevant for other inhomogeneities such as non-uniformity in stratification as well, thus providing an understanding of several recent studies that have highlighted superharmonic generation in non-uniform stratifications. Extending our study to finite shear (finite
$\omega \approx 0$, both of which are not possible in the no shear limit. Our weak shear limit conclusions are relevant for other inhomogeneities such as non-uniformity in stratification as well, thus providing an understanding of several recent studies that have highlighted superharmonic generation in non-uniform stratifications. Extending our study to finite shear (finite 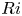 $Ri$) in an ocean-like exponential shear flow profile, we show that for cograde–cograde interactions, a significant number of divergence curves that start at
$Ri$) in an ocean-like exponential shear flow profile, we show that for cograde–cograde interactions, a significant number of divergence curves that start at 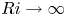 $Ri\to \infty$ will not extend below a cutoff
$Ri\to \infty$ will not extend below a cutoff 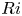 $Ri$
$Ri$ $\sim O(1)$. In contrast, for retrograde–retrograde interactions, the divergence curves extend all the way from
$\sim O(1)$. In contrast, for retrograde–retrograde interactions, the divergence curves extend all the way from 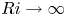 $Ri\to \infty$ to
$Ri\to \infty$ to 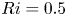 $Ri = 0.5$. For mixed interactions, new divergence curves appear at
$Ri = 0.5$. For mixed interactions, new divergence curves appear at  $\omega = 0$ for
$\omega = 0$ for  $Ri\sim O(10)$ and extend to other primary wave frequencies for smaller
$Ri\sim O(10)$ and extend to other primary wave frequencies for smaller 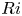 $Ri$. Consequently, the total (
$Ri$. Consequently, the total (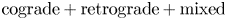 $\text {cograde} + \text {retrograde} + \text {mixed}$) number of resonant triads is of the same order for small
$\text {cograde} + \text {retrograde} + \text {mixed}$) number of resonant triads is of the same order for small 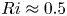 $Ri\approx 0.5$ as in the limit of weak shear (
$Ri\approx 0.5$ as in the limit of weak shear (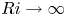 $Ri\to \infty$), although it attains a maximum at
$Ri\to \infty$), although it attains a maximum at 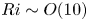 $Ri\sim O(10)$.
$Ri\sim O(10)$.