Published online by Cambridge University Press: 10 November 2023
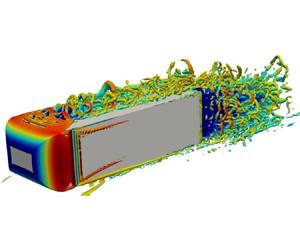
The turbulent wake past a square-back Ahmed body in close proximity to the ground experiences random side-to-side switching between two asymmetric positions, a phenomenon known as bi-modality. It has been observed to be sensitive to the dynamics of the upstream boundary layers formed along the body surfaces. Close to the body fore end, these separate and reattach, with hairpin vortices emanating from the reattachment points and growing along the surfaces before breaking down upstream of the base. This study uses wall-resolved large eddy simulations to investigate the effect of using suction to suppress these upstream boundary layer separations on the wake bi-modality. It is seen that, in the unforced flow (in the absence of suction), the smaller top and side surface vortices resulting from breakdown interact as they convect downstream. Steady suction is confirmed to suppress the boundary layer separations on the different body surfaces. When the boundary layer separations on the two side (vertical) surfaces are suppressed, it is found that horizontal bi-modality is completely inhibited with weak vertical asymmetry preserved. The interaction of the small top/side surface vortices is interrupted, damping boundary layer fluctuations just upstream of the base. Applying suction on different combinations of side/top/bottom boundary layer separations is found to have different effects on the underbody flow and the wake vertical balance, with bi-modality suppression dependent on side surface suction. This confirms that bi-modality is triggered, at least in part, by boundary layer disturbances on the surfaces perpendicular to the switching direction.