Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-23T00:53:25.889Z Has data issue: false hasContentIssue false
  • English
  • Français

Minimal surfaces on mirror-symmetric frames: a fluid dynamics analogy

Published online by Cambridge University Press:  19 June 2020

Mars M. Alimov Open the ORCID record for Mars M. Alimov [Opens in a new window] ,
Alexander V. Bazilevsky  and
Konstantin G. Kornev Open the ORCID record for Konstantin G. Kornev [Opens in a new window]
Mars M. Alimov
Affiliation:
Kazan Federal University, Kazan420008, Russia
Alexander V. Bazilevsky
Affiliation:
Ishlinsky Institute for Problems in Mechanics of the Russian Academy of Sciences, Moscow 119526, Russia
Konstantin G. Kornev*
Affiliation:
Department of Materials Science and Engineering, Clemson University, Clemson, SC 29634-0971, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

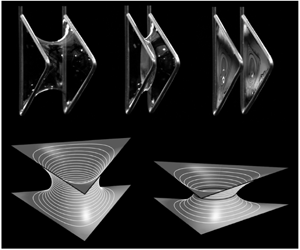
Chaplygin’s hodograph method of classical fluid mechanics is applied to explicitly solve the Plateau problem of finding minimal surfaces. The minimal surfaces are formed between two mirror-symmetric polygonal frames having a common axis of symmetry. Two classes of minimal surfaces are found: the class of regular surfaces continuously connecting the supporting frames forming a tube with complex shape; and the class of singular surfaces which have a partitioning film closing the tube in between. As an illustration of the general solution, minimal surfaces supported by triangular frames are fully described. The theory is experimentally validated using soap films. The general solution is compared with the known particular solutions obtained by the Weierstrass inverse method.

JFM classification

Complex Fluids: Foams Interfacial Flows (free surface): Thin films Mathematical Foundations: General fluid mechanics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 897 , 25 August 2020 , A36
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramowitz, M. & Stegun, I. A. 1965 Handbook of Mathematical Functions: With Formulas, Graphs, and Mathematical Tables, 9th edn, p. 1046. Dover.Google Scholar
Alimov, M. M. & Kornev, K. G. 2014 Meniscus on a shaped fibre: singularities and hodograph formulation. Proc. R. Soc. Lond. A 470 (2168), 20140113.CrossRefGoogle ScholarPubMed
Alimov, M. M. & Kornev, K. G. 2016 Piercing the water surface with a blade: singularities of the contact line. Phys. Fluids 28 (1), 012102.CrossRefGoogle Scholar
Alimov, M. M. & Kornev, K. G. 2019 Analysis of the shape hysteresis of a soap film supported by two circular rings. Fluid Dyn. 54 (1), 42–55.CrossRefGoogle Scholar
Andersson, S., Hyde, S. T., Larsson, K. & Lidin, S. 1988 Minimal surfaces and structures – from inorganic and metal crystals to cell membranes and biopolymers. Chem. Rev. 88 (1), 221–242.CrossRefGoogle Scholar
Arfken, G. B., Weber, H. J. & Harris, F. E. 2012 Mathematical Methods for Physicists, 7th edn, p. 1220. Elsevier.Google Scholar
Batchelor, G. K. 2000 An Introduction to Fluid Dynamics. Cambridge University Press.CrossRefGoogle Scholar
Bers, L. 1951a Boundary value problems for minimal surfaces with singularities at infinity. Trans. Am. Math. Soc. 70 (May), 465–491.CrossRefGoogle Scholar
Bers, L. 1951b Isolated singularities of minimal surfaces. Ann. Maths 53 (2), 364–386.CrossRefGoogle Scholar
Bers, L. 2016 Mathematical Aspects of Subsonic and Transonic Gas Dynamics. Dover.Google Scholar
Buckingham, R. & Bush, J. W. M. 2001 Fluid polygons. Phy. Fluids 13, S10.CrossRefGoogle Scholar
Carrier, J. F. & Krook, M. 2005 Functions of a Complex Variable: Theory and Technique. Society for Industrial and Applied Mathematics.CrossRefGoogle Scholar
Chaplygin, S. A. 1904 Gas jets. Uchenie Zapiski Imperatorskogo Moskovskogo Universiteta 21, 1–121.Google Scholar
Chaplygin, S. A.1944 Gas jets. NACA Tech. Memo. 1063.Google Scholar
Chen, Y. J. & Steen, P. H. 1997 Dynamics of inviscid capillary breakup: collapse and pinchoff of a film bridge. J. Fluid Mech. 341, 245–267.CrossRefGoogle Scholar
Clanet, C. 2001 Dynamics and stability of water bells. J. Fluid Mech. 430, 111–147.CrossRefGoogle Scholar
Courant, R. 2005 Dirichlet’s Principle, Conformal Mapping, and Minimal Surfaces. Dover.Google Scholar
Cvijovic, D. & Klinowski, J. 1992a The T and CLP families of triply periodic minimal surfaces. 2. The properties and computation of T-surfaces. J. Phys. I 2 (12), 2191–2205.Google Scholar
Cvijovic, D. & Klinowski, J. 1992b The T and CLP families of triply periodic minimal surfaces. 3. The properties and computation of CLP surfaces. J. Phys. I 2 (12), 2207–2220.Google Scholar
Dierkes, U., Hildebrandt, S. & Tromba, A. J. 2010 Global Analysis of Minimal Surfaces, vol. 341, pp. 3–529. Springer.CrossRefGoogle Scholar
Dressaire, E., Courbin, L., Delancy, A., Roper, M. & Stone, H. A. 2013 Study of polygonal water bells: inertia-dominated thin-film flows over microtextured surfaces. J. Fluid Mech. 721, 46–57.CrossRefGoogle Scholar
Goldstein, R. V. & Entov, V. M. 1994 Qualitative Methods in Continuum Mechanics. Longman & Wiley.Google Scholar
Fogden, A. 1993 Parametrization of triply periodic minimal surfaces. 3. General algorithm and specific examples for the irregular class. Acta Crystallogr. A 49, 409–421.CrossRefGoogle Scholar
Fogden, A. & Hyde, S. T. 1992a Parametrization of triply periodic minimal surfaces. 1. Mathematical basis of the construction algorithm for the regular class. Acta Crystallogr. A 48, 442–451.CrossRefGoogle Scholar
Fogden, A. & Hyde, S. T. 1992b Parametrization of triply periodic minimal surfaces. 2. Regular class solutions. Acta Crystallogr. A 48, 575–591.CrossRefGoogle Scholar
Han, L. & Che, S. A. 2018 An overview of materials with triply periodic minimal surfaces and related geometry: from biological structures to self-assembled systems. Adv. Mater. 30 (17), 22.CrossRefGoogle ScholarPubMed
Hildebrandt, S. & Tromba, A. 1986 Mathematics and Optimal Form. W. H. Freeman.Google Scholar
Karcher, H. 1989 Construction of minimal surfaces. In Surveys in Geometry, vol. 12, pp. 1–96. University of Tokyo.Google Scholar
Karcher, H. & Polthier, K. 1996 Construction of triply periodic minimal surfaces. Phil. Trans. R. Soc. Math. Phys. Engng Sci. 354 (1715), 2077–2104.Google Scholar
Khristianovich, S. A. 1940 Flow of groundwater not obeying Darcy’s law. Prikl. Mat. Mekh. 4 (1), 33–52.Google Scholar
Klinowski, J., Mackay, A. L. & Terrones, H. 1996 Curved surfaces in chemical structure. Phil. Trans. R. Soc. Math. Phys. Engng Sci. 354 (1715), 1975–1987.Google Scholar
Lidin, S. 1988 Ring-like minimal surfaces. J. Phys. 49 (3), 421–427.CrossRefGoogle Scholar
Lord, E. A., Mackay, A. L. & Ranganathan, S. 2006 New Geometries for New Materials. p. 258. Cambridge University Press.Google Scholar
Nitsche, J. C. C. 2011 Lectures on Minimal Surfaces, reissue edn, vol. 1. Cambridge University Press.Google Scholar
Plateau, J. 1863 Experimental and theoretical researches on the figures on equilibrium of a liquid mass withdrawn from the action of gravity. In Annual Report of the Board of Regents of the Smithsonian Institution, pp. 207–285. Government Printing Office.Google Scholar
Plateau, J. 1873 Statique Experimentale et Theorique des Liquides Soumis aux Seules Forces Moleculaires. Gauthier-Villars.Google Scholar
Salkin, L., Schmit, A., Panizza, P. & Courbin, L. 2014 Influence of boundary conditions on the existence and stability of minimal surfaces of revolution made of soap films. Am. J. Phys. 82 (9), 839–847.CrossRefGoogle Scholar
Sett, S., Sinha-Ray, S. & Yarin, A. L. 2013 Gravitational drainage of foam films. Langmuir 29, 4934–4947.CrossRefGoogle ScholarPubMed
Sokolovsky, V. V. 1949 On non-linear filtration of underground water. Prikl. Mat. Mekh. 13, 525–536.Google Scholar
Thi, D. T. & Fomenko, A. T. 1991 Minimal Surfaces, Stratified Multivarifolds, and the Plateau Problem, vol. 84, p. 404. American Mathematical Society.CrossRefGoogle Scholar
Whittaker, E. T. & Watson, G. N. 1996 A Course of Modern Analysis, 4th edn. p. 620. Cambridge University Press.CrossRefGoogle Scholar

Alimov et al. supplementary movie

Three dimensional configuration of a periodic cell of the Schwarz' H triply periodic minimal surface obtained using Chaplygin's hodograph method.

Download Alimov et al. supplementary movie(Video)
Video 5.6 MB
Supplementary material: File

Alimov et al. supplementary material

Supplementary data

Download Alimov et al. supplementary material(File)
File 1.3 MB