Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-26T23:23:55.107Z Has data issue: false hasContentIssue false
  • English
  • Français

Electrically modulated dynamics of a compound droplet in a confined microfluidic environment

Published online by Cambridge University Press:  11 November 2019

Somnath Santra ,
Sayan Das  and
Suman Chakraborty Open the ORCID record for Suman Chakraborty [Opens in a new window]
Somnath Santra
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Kharagpur, Kharagpur, West Bengal – 721302, India
Sayan Das
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Kharagpur, Kharagpur, West Bengal – 721302, India
Suman Chakraborty*
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Kharagpur, Kharagpur, West Bengal – 721302, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

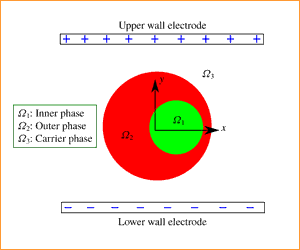
Compound droplets confined in a microfluidic channel often exhibit intriguing shapes, primarily attributable to complex hydrodynamic interactions over small scales. Here, we show that the effect of electrohydrodynamic interactions may modulate the shape evolution of the same in a somewhat non-trivial manner. By adopting a phase field formalism, our studies reveal that the combined influence of electrohydrodynamics and fluidic confinement eventually culminates towards influencing the droplet transients, distortion of the local field, as well as droplet stabilization or destabilization, allowing one to develop different regimes of shape evolution that are distinct from the ones reported in earlier studies on single droplet dynamics. Under the assumption of negligible fluid inertia and small shape deformation, we also develop an asymptotic model to predict the transient as well as the steady-state behaviour of the compound droplet for the limiting case of an unbounded suspending medium. The relative magnitude of the permittivity and conductivity of the system, in conjunction with the channel confinement, is seen to play an important role in altering the deformation characteristics of either of the interfaces. We further observe that, depending on these electrical properties, the inner droplet, if eccentrically located, may exhibit a to-and-fro or a simple translational motion. Remarkably, below a threshold confinement dimension, we unravel the onset of a definitive translational motion of the inner droplet instead of a more intuitive to-and-fro motion, thereby rendering its migration characteristics to be independent of any electrical properties. Furthermore, the viscosity contrast of the fluid phase also plays a vital role in controlling the motion and deformation dynamics of the droplet in both confined and unbounded domains. These results may bear far-reaching consequences towards understanding the control of cellular dynamics in confined in vivo physiological passages by introducing embedded electrical chips, as well as electrohydrodynamically modulated lab-on-a-chip devices for medical diagnostics on a cellular level.

JFM classification

Drops and Bubbles: Drops Drops and Bubbles: Electrohydrodynamic effects
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 882 , 10 January 2020 , A23
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Araki, T. & Tsukube, H. 1990 Liquid Membranes: Chemical Applications. CRC Press.Google Scholar
Badalassi, V. E., Ceniceros, H. D. & Banerjee, S. 2003 Computation of multiphase systems with phase field models. J. Comput. Phys. 190 (2), 371–397.Google Scholar
Baygents, J. C., Rivette, N. J. & Stone, H. A. 1998 Electrohydrodynamic deformation and interaction of drop pairs. J. Fluid Mech. 368, 359–375.Google Scholar
Behjatian, A. & Esmaeeli, A. 2013 Electrohydrodynamics of a liquid column under a transverse electric field in confined domains. Intl J. Multiphase Flow 48, 71–81.Google Scholar
Behjatian, A. & Esmaeeli, A. 2015 Transient electrohydrodynamics of compound drops. Acta Mech. 226 (8), 2581–2606.Google Scholar
Bei, Z.-M., Jones, T. B., Tucker-Schwartz, A. & Harding, D. R. 2008 Electric field mediated droplet centering. Appl. Phys. Lett. 93 (18), 184101.Google Scholar
Das, D. & Saintillan, D. 2017a A nonlinear small-deformation theory for transient droplet electrohydrodynamics. J. Fluid Mech. 810, 225–253.Google Scholar
Das, D. & Saintillan, D. 2017b Electrohydrodynamics of viscous drops in strong electric fields: numerical simulations. J. Fluid Mech. 829, 127–152.Google Scholar
Esmaeeli, A. & Behjatian, A. 2012 Electrohydrodynamics of a liquid drop in confined domains. Phys. Rev. E 86 (3), 036310.Google Scholar
Esmaeeli, A. & Sharifi, P. 2011 The transient dynamics of a liquid column in a uniform transverse electric field of small strength. J. Electrostat. 69 (6), 504–511.Google Scholar
Feng, J. Q. 1999 Electrohydrodynamic behaviour of a drop subjected to a steady uniform electric field at finite electric Reynolds number. Proc. R. Soc. Lond. A 455 (1986), 2245–2269.Google Scholar
Feng, J. Q. & Scott, T. C. 1996 A computational analysis of electrohydrodynamics of a leaky dielectric drop in an electric field. J. Fluid Mech. 311, 289–326.Google Scholar
Gouz, H. N. & Sadhal, S. S. 1989 Fluid dynamic and stability analysis of a compound droplet in an electric field. Q. J. Mech. Appl. Maths 42 (1), 65–83.Google Scholar
Ha, J.-W. & Yang, S.-M. 1999 Fluid dynamics of a double emulsion droplet in an electric field. Phys. Fluids 11 (5), 1029–1041.Google Scholar
Halim, M. A. & Esmaeeli, A. 2013 Computational studies on the transient electrohydrodynamics of a liquid drop. Fluid Dyn. Mater. Process. 9 (4), 435–460.Google Scholar
Jacqmin, D. 1999 Calculation of two-phase Navier–Stokes flows using phase-field modeling. J. Comput. Phys. 155 (1), 96–127.Google Scholar
Kan, H.-C., Udaykumar, H. S., Shyy, W. & Tran-Son-Tay, R. 1998 Hydrodynamics of a compound drop with application to leukocyte modeling. Phys. Fluids 10 (4), 760–774.Google Scholar
Lac, E. & Homsy, G. M. 2007 Axisymmetric deformation and stability of a viscous drop in a steady electric field. J. Fluid Mech. 590, 239–264.Google Scholar
Lanauze, J. A., Walker, L. M. & Khair, A. S. 2015 Nonlinear electrohydrodynamics of slightly deformed oblate drops. J. Fluid Mech. 774, 245–266.Google Scholar
Li, N. N. 1971 Separation of hydrocarbons by liquid membrane permeation. Ind. Engng Chem. Process Des. Dev. 10 (2), 215–221.Google Scholar
Li, N. N. & Asher, W. J. 1973 Blood Oxygenation by Liquid Membrane Permeation, pp. 1–14. American Chemical Society.Google Scholar
Li, N. N. & Shrier, A. 1972 Recent Development in Separation Science (ed. Norman, N. L.). CRC Press.Google Scholar
Li, Y., Jun-Yan Suen, J., Prince, E., Larin, E. M., Klinkova, A., Thérien-Aubin, H., Zhu, S., Yang, B., Helmy, A. S., Lavrentovich, O. D. et al. 2016 Colloidal cholesteric liquid crystal in spherical confinement. Nat. Commun. 7, 12520.Google Scholar
Lin, Y., Skjetne, P. & Carlson, A. 2012 A phase field model for multiphase electro-hydrodynamic flow. Intl J. Multiphase Flow 45, 1–11.Google Scholar
López-Herrera, J. M., Popinet, S. & Herrada, M. A. 2011 A charge-conservative approach for simulating electrohydrodynamic two-phase flows using volume-of-fluid. J. Comput. Phys. 230 (5), 1939–1955.Google Scholar
Mahlmann, S. & Papageorgiou, D. T. 2009 Numerical study of electric field effects on the deformation of two-dimensional liquid drops in simple shear flow at arbitrary Reynolds number. J. Fluid Mech. 626, 367–393.Google Scholar
Mandal, S., Bandopadhyay, A. & Chakraborty, S. 2016a The effect of uniform electric field on the cross-stream migration of a drop in plane Poiseuille flow. J. Fluid Mech. 809, 726–774.Google Scholar
Mandal, S., Chaudhury, K. & Chakraborty, S. 2014 Transient dynamics of confined liquid drops in a uniform electric field. Phys. Rev. E 89 (5), 1–17.Google Scholar
Mandal, S., Ghosh, U. & Chakraborty, S. 2016b Effect of surfactant on motion and deformation of compound droplets in arbitrary unbounded Stokes flows. J. Fluid Mech. 803, 200–249.Google Scholar
Mandal, S., Sinha, S., Bandopadhyay, A. & Chakraborty, S. 2018 Drop deformation and emulsion rheology under the combined influence of uniform electric field and linear flow. J. Fluid Mech. 841, 408–433.Google Scholar
Mataumoto, S. & Kang, W. W. 1989 Formation and applications of multiple emulsions. J. Dispers. Sci. Technol. 10 (4–5), 455–482.Google Scholar
Melcher, J. R. & Taylor, G. I. 1969 Electrohydrodynamics: a review of the role of interfacial shear stresses. Annu. Rev. Fluid Mech. 1 (1), 111–146.Google Scholar
Mhatre, S., Vivacqua, V., Ghadiri, M., Abdullah, A. M., Hassanpour, A., Hewakandamby, B., Azzopardi, B. & Kermani, B. 2015 Electrostatic phase separation: a review. Chem. Engng Res. Des. 96, 177–195.Google Scholar
Nganguia, H. & Layton, A. T. 2016 Electrohydrodynamics of a viscous drop with inertia. Phys. Rev. E 93, 53114.Google Scholar
Nganguia, H., Young, Y.-N., Layton, A. T., Lai, M.-C. & Hu, W.-F. 2016 Electrohydrodynamics of a viscous drop with inertia. Phys. Rev. E 93 (5), 53114.Google Scholar
Reddy, M. N. & Esmaeeli, A. 2009 The EHD-driven fluid flow and deformation of a liquid jet by a transverse electric field. Intl J. Multiphase Flow 35 (11), 1051–1065.Google Scholar
Salipante, P. F. & Vlahovska, P. M. 2010 Electrohydrodynamics of drops in strong uniform dc electric fields. Phys. Fluids 22 (11), 112110.Google Scholar
Santra, S., Mandal, S. & Chakraborty, S. 2018 Electrohydrodynamics of confined two-dimensional liquid droplets in uniform electric field. Phys. Fluids 30 (6), 62003.Google Scholar
Santra, S., Mandal, S. & Chakraborty, S. 2019a Confinement effect on electrically induced dynamics of a droplet in shear flow. Phys. Rev. E 100 (3), 33101.Google Scholar
Santra, S., Sen, D., Das, S. & Chakraborty, S. 2019b Electrohydrodynamic interaction between droplet pairs in a confined shear flow. Phys. Fluids 31 (3), 32005.Google Scholar
Saville, D. A. 1997 Electrohydrodynamics: the Taylor–Melcher leaky dielectric model. Annu. Rev. Fluid Mech. 29 (1), 27–64.Google Scholar
Sibillo, V., Pasquariello, G., Simeone, M., Cristini, V. & Guido, S. 2006 Drop deformation in microconfined shear flow. Phys. Rev. Lett. 97, 1–4.Google Scholar
Soni, P., Juvekar, V. A. & Naik, V. M. 2013 Investigation on dynamics of double emulsion droplet in a uniform electric field. J. Electrostat. 71 (3), 471–477.Google Scholar
Stefano, G. & Valentina, P. 2010 Droplet deformation under confined Poiseuille flow. Adv. Colloid Interface Sci. 161 (1–2), 89–101.Google Scholar
Stone, H. A., Stroock, A. D. & Ajdari, A. 2004 Engineering flows in small devices: microfluidics toward a Lab-on-a-Chip. Annu. Rev. Fluid Mech. 36 (1), 381–411.Google Scholar
Tasoglu, S., Kaynak, G., Szeri, A. J., Demirci, U. & Muradoglu, M. 2010 Impact of a compound droplet on a flat surface: A model for single cell epitaxy. Phys. Fluids 22 (8), 82103.Google Scholar
Taylor, G. 1966 Studies in electrohydrodynamics. I. The circulation produced in a drop by electrical field. Proc. R. Soc. Lond. A 291 (1425), 159–166.Google Scholar
Teh, S.-Y., Lin, R., Hung, L.-H. & Lee, A. P. 2008 Droplet microfluidics. Lab on a Chip 8 (2), 198–220.Google Scholar
Torza, S., Cox, R. G. & Mason, S. G. 1971 Electrohydrodynamic deformation and burst of liquid drops. Phil. Trans. R. Soc. Lond. A 269 (1198), 295–319.Google Scholar
Tsukada, T., Katayama, T., Ito, Y. & Hozawa, M. 1993 Theoretical and experimental studies of circulations inside and outside a deformed drop under a uniform electric field. J. Chem. Engng Japan 26 (6), 698–703.Google Scholar
Tsukada, T., Mayama, J., Sato, M. & Hozawa, M. 1997 Theoretical and experimental studies on the behavior of a compound drop under a uniform DC electric field. J. Chem. Engng Japan 30 (2), 215–222.Google Scholar
Tsukada, T., Yamamoto, Y., Katayama, T. & Hozawa, M. 1994 Effect of an electric field on the behavior of a drop moving in a quiescent liquid. J. Chem. Engng Japan 27 (5), 662–666.Google Scholar
Tucker-Schwartz, A. K., Bei, Z., Garrell, R. L. & Jones, T. B. 2010 Polymerization of electric field-centered double emulsion droplets to create polyacrylate shells. Langmuir 26 (24), 18606–18611.Google Scholar
Supplementary material: File

Santra et. al supplementary material

Santra et. al supplementary material

Download Santra et. al supplementary material(File)
File 1.7 MB