Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-17T13:21:34.933Z Has data issue: false hasContentIssue false
  • English
  • Français

The structure and budget of turbulent kinetic energy in front of a wall-mounted cylinder

Published online by Cambridge University Press:  22 August 2017

Wolfgang Schanderl ,
Ulrich Jenssen ,
Claudia Strobl  and
Michael Manhart Open the ORCID record for Michael Manhart [Opens in a new window]
Wolfgang Schanderl
Affiliation:
Chair of Hydromechanics, Department of Civil, Geo and Environmental Engineering, Technische Universität München, Arcisstr. 21, 80333 München, Germany
Ulrich Jenssen
Affiliation:
Chair of Hydromechanics, Department of Civil, Geo and Environmental Engineering, Technische Universität München, Arcisstr. 21, 80333 München, Germany
Claudia Strobl
Affiliation:
Chair of Hydromechanics, Department of Civil, Geo and Environmental Engineering, Technische Universität München, Arcisstr. 21, 80333 München, Germany
Michael Manhart*
Affiliation:
Chair of Hydromechanics, Department of Civil, Geo and Environmental Engineering, Technische Universität München, Arcisstr. 21, 80333 München, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the flow and turbulence structure in front of a cylinder mounted on a flat plate by a combined study using highly resolved large-eddy simulation and particle image velocimetry. The Reynolds number based on the bulk velocity and cylinder diameter is 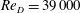$Re_{D}=39\,000$. As the cylinder is placed in an open channel, we take special care to simulate open-channel flow as the inflow condition, including secondary flows that match the inflow in the experiment. Due to the high numerical resolution, subgrid contributions to the Reynolds stresses are negligible and the modelled dissipation plays a minor role in major parts of the flow field. The accordance of the experimental and numerical results is good. The shear in the approach flow creates a vertical pressure gradient, inducing a downflow in the cylinder front. This downflow, when deflected in the upstream direction at the bottom plate, gives rise to a so-called horseshoe vortex system. The most upstream point of flow reversal at the wall is found to be a stagnation point which appears as a sink instead of a separation point in the symmetry plane in front of the cylinder. The wall shear stress is largest between the main (horseshoe) vortex and the cylinder, and seems to be mainly governed by the strong downflow in front of the cylinder as turbulent stresses are small in this region. Due to a strong acceleration along the streamlines, a region of relatively small turbulent kinetic energy is found between the horseshoe vortex and the cylinder. When passing under the horseshoe vortex, the upstream-directed jet formed by the deflected downflow undergoes a deceleration which gives rise to a strong production of turbulent kinetic energy. We find that pressure transport of turbulent kinetic energy is important for the initiation of the large production rates by increasing the turbulence level in the upstream jet near the wall. The distribution of the dissipation of turbulent kinetic energy is similar to that of the turbulent kinetic energy. Large values of dissipation occur around the centre of the horseshoe vortex and near the wall in the region where the jet decelerates. While the small scales are nearly isotropic in the horseshoe vortex centre, they are anistotropic near the wall. This can be explained by a vertical flapping of the upstream-directed jet. The distribution and level of dissipation, turbulent and pressure transport of turbulent kinetic energy are of crucial interest to turbulence modelling in the Reynolds-averaged context. To the best of our knowledge, this is the first time that these terms have been documented in this kind of flow.

JFM classification

Wakes/Jets: Separated flows Turbulent Flows: Turbulent boundary layers Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 827 , 25 September 2017 , pp. 285 - 321
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Apsilidis, N., Diplas, P., Dancey, C. L. & Bouratsis, P. 2015 Time-resolved flow dynamics and Reynolds number effects at a wall–cylinder junction. J. Fluid Mech. 776, 475–511.CrossRefGoogle Scholar
Apsilidis, N., Khosronejad, A., Sotiropoulos, F., Dancey, C. L. & Diplas, P. 2012 Physical and numerical modeling of the turbulent flow field upstream of a bridge pier. In International Conference on Scour and Erosion 6, Paris, Ecole des Arts et Metiers - Paris Tech.Google Scholar
Baker, C. J. 1979 The laminar horseshoe vortex. J. Fluid Mech. 95, 347–367.CrossRefGoogle Scholar
Bruns, J., Dengel, P. & Fernholz, H. H.1992 Mean flow and turbulence measurements in an incompressible two-dimensional turbulent boundary layer. Part I: data. Tech. Rep., Herman-Föttinger-Institut für Thermo- und Fluiddynamik, TU Berlin.Google Scholar
Clauser, F. H. 1954 Turbulent boundary layer in adverse pressure gradients. J. Aero. Sci. 21, 91–108.Google Scholar
Dargahi, B. 1989 The turbulent flow field around a circular cylinder. Exp. Fluids 8 (1–2), 1–12.CrossRefGoogle Scholar
Demuren, A. O. & Rodi, W. 1984 Calculation of turbulence-driven secondary motion in non-circular ducts. J. Fluid Mech. 140, 189–222.CrossRefGoogle Scholar
Devenport, W. J. & Simpson, R. L. 1990 Time-dependent and time-averaged turbulence structure near the nose of a wing–body junction. J. Fluid Mech. 210, 23–55.CrossRefGoogle Scholar
Dey, S. & Raikar, R. V. 2007 Characteristics of horseshoe vortex in developing scour holes at piers. J. Hydraul Engng 133 (4), 399–413.CrossRefGoogle Scholar
Escauriaza, C. & Sotiropoulos, F. 2011 Reynolds number effects on the coherent dynamics of the turbulent horseshoe vortex system. Flow Turbul. Combust. 86 (2), 231–262.Google Scholar
Fernholz, H. H. & Finley, P. J. 1996 The incompressible zero-pressure-gradient turbulent boundary layer: an assessment of the data. Prog. Aerosp. Sci. 32 (4), 245–311.CrossRefGoogle Scholar
Graf, W. H. & Istiarto, I. 2002 Flow pattern in the scour hole around a cylinder. J. Hydraul Res. 40 (1), 13–20.Google Scholar
Gresho, P. M. & Lee, R. 1981 Don’t suppress the wiggles – they’re telling you something. Comput. Fluids 9, 223–253.Google Scholar
Kähler, C. J., Scharnowski, S. & Cierpka, C. 2012 On the uncertainty of digital PIV and PTV near walls. Exp. Fluids 52 (6), 1641–1656.CrossRefGoogle Scholar
Kähler, C. J., Scholz, U. & Ortmanns, J. 2006 Wall-shear-stress and near-wall turbulence measurements up to single pixel resolution by means of long-distance micro-PIV. Exp. Fluids 41 (2), 327–341.CrossRefGoogle Scholar
Kirkil, G. & Constantinescu, G. 2015 Effects of cylinder Reynolds number on the turbulent horseshoe vortex system and near wake of a surface-mounted circular cylinder. Phys. Fluids 27, 075102.Google Scholar
Kumar, A. & Kothyari, U. C. 2011 Three-dimensional flow characteristics within the scour hole around circular uniform and compound piers. J. Hydraul Engng 138 (5), 420–429.CrossRefGoogle Scholar
Lilly, D. K. 1967 The representation of small-scale turbulence in numerical simulation experiments. In Proceedings of the IBM Scientific Computing Symposium on Environmental Sciences, IBM Form No. 320–1951, pp. 195–210. IBM Data Processing Division.Google Scholar
Manhart, M. 2004 A zonal grid algorithm for DNS of turbulent boundary layers. Comput. Fluids 33 (3), 435–461.Google Scholar
Melville, B. W. & Raudkivi, A. J. 1977 Flow characteristics in local scour at bridge piers. J. Hydraul. Res. 15 (4), 373–380.Google Scholar
Nezu, I. & Nakagawa, H. 1993 Turbulence in Open-Channel Flows. A.A. Balkema.Google Scholar
Nicoud, F. & Ducros, F. 1999 Subgrid-scale stress modelling based on the square of the velocity gradient tensor. Flow Turbul. Combust. 62 (3), 183–200.CrossRefGoogle Scholar
Paik, J., Escauriaza, C. & Sotiropoulos, F. 2007 On the bimodal dynamics of the turbulent horseshoe vortex system in a wing–body junction. Phys. Fluids 19, 045107.Google Scholar
Peller, N.2010 Numerische Simulation turbulenter Strömungen mit Immersed Boundaries. PhD thesis, Technische Universität München.Google Scholar
Peller, N., Duc, A. L., Tremblay, F. & Manhart, M. 2006 High-order stable interpolations for immersed boundary methods. Intl J. Numer. Meth. Fluids 52, 1175–1193.Google Scholar
Pfleger, F.2011 Experimentelle Untersuchung der Auskolkung um einen zylindrischen Brückenpfeiler. PhD thesis, Technische Universität München.Google Scholar
Pope, S. B. 2011 Turbulent Flows. Cambridge University Press.Google Scholar
Raffel, M., Willert, C., Wereley, S. & Kompenhans, J. 2007 Particle Image Velocimetry – A Practical Guide, 2nd edn. Springer.Google Scholar
Roulund, A., Sumer, B. M., Fredsoe, J. & Michelsen, J. 2005 Numerical and experimental investigation of flow and scour around a circular pile. J. Fluid Mech. 534, 351–401.Google Scholar
Ryu, S., Emory, M., Iaccarino, G., Campos, A. & Duraisamy, K. 2016 Large-eddy simulation of a wing–body junction flow. AIAA J. 54 (3), 793–804.CrossRefGoogle Scholar
Schanderl, W. & Manhart, M. 2015 Non-equilibrium near wall velocity profiles in the flow around a cylinder mounted on a flat plate. In 15th European Turbulence Conference, TU Delft.Google Scholar
Schanderl, W. & Manhart, M. 2016 Reliability of wall shear stress estimations of the flow around a wall-mounted cylinder. Comput. Fluids 128, 16–29.CrossRefGoogle Scholar
Schlichting, H. & Gersten, K. 2006 Boundary Layer Theory. Springer.Google Scholar
Simpson, R. L. 2001 Junction flows. Annu. Rev. Fluid Mech. 33, 415–443.CrossRefGoogle Scholar
Strobl, C., Jenssen, U. & Manhart, M.2016 Reconstructing velocity statistics from single pixel ensemble correlation PIV. Exp. Fluids (submitted).Google Scholar
Tanaka, T. & Eaton, J. K. 2007 A correction method for measuring turbulence kinetic energy dissipation rate by PIV. Exp. Fluids 42 (6), 893–902.CrossRefGoogle Scholar
Unger, J. & Hager, W. H. 2007 Down-flow and horseshoe vortex characteristics of sediment embedded bridge piers. Exp. Fluids 42, 1–19.Google Scholar
Werner, H.1991 Grobstruktursimulation der turbulenten Strömung über eine querliegende Rippe in einem Plattenkanal bei hoher Reynoldszahl. PhD thesis, Technische Universität München.Google Scholar
Westerweel, J., Geelhoed, P. F. & Lindken, R. 2004 Single-pixel resolution ensemble correlation for micro-PIV applications. Exp. Fluids 37 (3), 375–384.Google Scholar