Published online by Cambridge University Press: 10 November 2023
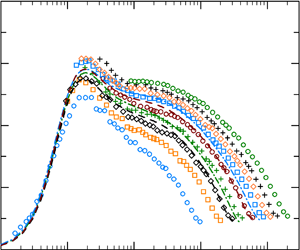
Measurement data sets are presented for the turbulence intensity profile of three velocity components ( $u$,
$u$,  $v$ and
$v$ and  $w$) and turbulent kinetic energy (TKE,
$w$) and turbulent kinetic energy (TKE,  $k$) over a wide range of Reynolds numbers from
$k$) over a wide range of Reynolds numbers from  $Re_\tau =990$ to 20 750 in a pipe flow. The turbulence intensity profiles of the
$Re_\tau =990$ to 20 750 in a pipe flow. The turbulence intensity profiles of the  $u$- and
$u$- and  $w$-component show logarithmic behaviour, and that of the
$w$-component show logarithmic behaviour, and that of the  $v$-component shows a constant region at high Reynolds numbers,
$v$-component shows a constant region at high Reynolds numbers,  $Re_\tau >10\,000$. Furthermore, a logarithmic region is also observed in the TKE profile at
$Re_\tau >10\,000$. Furthermore, a logarithmic region is also observed in the TKE profile at  $y/R=0.055\unicode{x2013}0.25$. The Reynolds number dependences of peak values of
$y/R=0.055\unicode{x2013}0.25$. The Reynolds number dependences of peak values of  $u$-,
$u$-,  $w$-component and TKE fit to both a logarithmic law (Marusic et al., Phys. Rev. Fluids, vol. 2, 2017, 100502) and an asymptotic law (Chen and Sreenivasan, J. Fluid Mech., vol. 908, 2020, R3), within the uncertainty of measurement. The Reynolds number dependence of the bulk TKE
$w$-component and TKE fit to both a logarithmic law (Marusic et al., Phys. Rev. Fluids, vol. 2, 2017, 100502) and an asymptotic law (Chen and Sreenivasan, J. Fluid Mech., vol. 908, 2020, R3), within the uncertainty of measurement. The Reynolds number dependence of the bulk TKE  $k^+_{bulk}$, which is the total amount of TKE in the cross-sectional area of the pipe also fits to both laws. When the asymptotic law is applied to the
$k^+_{bulk}$, which is the total amount of TKE in the cross-sectional area of the pipe also fits to both laws. When the asymptotic law is applied to the  $k^+_{bulk}$, it asymptotically increases to the finite value
$k^+_{bulk}$, it asymptotically increases to the finite value  $k^+_{bulk}=11$ as the Reynolds number increases. The contribution ratio
$k^+_{bulk}=11$ as the Reynolds number increases. The contribution ratio  $\langle u'^2\rangle /k$ reaches a plateau, and the value tends to be constant within
$\langle u'^2\rangle /k$ reaches a plateau, and the value tends to be constant within  $100< y^+<1000$ at
$100< y^+<1000$ at  $Re_\tau >10\ 000$. Therefore, the local balance of each velocity component also indicates asymptotic behaviour. The contribution ratios are balanced in this region at high Reynolds numbers as
$Re_\tau >10\ 000$. Therefore, the local balance of each velocity component also indicates asymptotic behaviour. The contribution ratios are balanced in this region at high Reynolds numbers as  $\langle u'^2\rangle /k\simeq 1.25$,
$\langle u'^2\rangle /k\simeq 1.25$,  $\langle w'^2\rangle /k\simeq 0.5$ and
$\langle w'^2\rangle /k\simeq 0.5$ and  $\langle v'^2\rangle /k \simeq 0.25$.
$\langle v'^2\rangle /k \simeq 0.25$.