Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mazur, B.
Tate, J.
and
Teitelbaum, J.
1986.
Onp-adic analogues of the conjectures of Birch and Swinnerton-Dyer.
Inventiones Mathematicae,
Vol. 84,
Issue. 1,
p.
1.
K�hler, G�nter
1988.
Theta series on the Hecke groupsG( $$\sqrt 2 $$ ) andG ( $$\sqrt 3 $$ ).
Mathematische Zeitschrift,
Vol. 197,
Issue. 1,
p.
69.
von Köhler, G
1988.
Theta series on the theta group.
Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg,
Vol. 58,
Issue. 1,
p.
15.
Gordon, Basil
and
Sinor, Dale
1989.
Number Theory, Madras 1987.
Vol. 1395,
Issue. ,
p.
173.
Borcherds, Richard E.
1994.
First European Congress of Mathematics Paris, July 6–10, 1992.
p.
411.
Borcherds, Richard E.
1994.
First European Congress of Mathematics.
p.
411.
Ono, Ken
1994.
Shimura sums related to imaginary quadratic fields.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 70,
Issue. 5,
Gordon, Basil
and
Robins, Sinai
1995.
Lacunarity of Dedekind η-products.
Glasgow Mathematical Journal,
Vol. 37,
Issue. 1,
p.
1.
Ono, Ken
Robins, Sinai
and
Wahl, Patrick T.
1995.
On the representation of integers as sums of triangular numbers.
Aequationes Mathematicae,
Vol. 50,
Issue. 1-2,
p.
73.
Sze, Lawrence
1998.
Calculatingp(n) Modulo Small Primes Using Quadratic Forms.
Journal of Number Theory,
Vol. 70,
Issue. 2,
p.
121.
Kahl, Helmut
and
Köhler, Günter
1999.
Components of Hecke Theta Series.
Journal of Mathematical Analysis and Applications,
Vol. 232,
Issue. 2,
p.
312.
Ahlgren, Scott
2001.
Multiplicative Relations in Powers of Euler's Product.
Journal of Number Theory,
Vol. 89,
Issue. 2,
p.
222.
Ahlgren, Scott
2002.
The Points of a Certain Fivefold over Finite Fields and the Twelfth Power of the Eta Function.
Finite Fields and Their Applications,
Vol. 8,
Issue. 1,
p.
18.
Baier, Harald
and
Köhler, Gunter
2003.
How to Compute the Coefficients of the Elliptic Modular Functionj(z).
Experimental Mathematics,
Vol. 12,
Issue. 1,
p.
115.
Boylan, Matthew
2003.
Exceptional congruences for the coefficients of certain eta-product newforms.
Journal of Number Theory,
Vol. 98,
Issue. 2,
p.
377.
PACHE, CLAUDE
2005.
SHELLS OF SELFDUAL LATTICES VIEWED AS SPHERICAL DESIGNS.
International Journal of Algebra and Computation,
Vol. 15,
Issue. 05n06,
p.
1085.
Chan, Heng Huat
Cooper, Shaun
and
Toh, Pee Choon
2006.
The 26th power of Dedekind's η-function.
Advances in Mathematics,
Vol. 207,
Issue. 2,
p.
532.
Chan, Heng Huat
Cooper, Shaun
and
Toh, Pee Choon
2007.
Ramanujan's Eisenstein series and powers of Dedekind's eta-function.
Journal of the London Mathematical Society,
Vol. 75,
Issue. 1,
p.
225.
Dandurand, Brian
and
Penniston, David
2009.
ℓ-Divisibility of ℓ-regular partition functions.
The Ramanujan Journal,
Vol. 19,
Issue. 1,
p.
63.
Bannai, Eiichi
and
Bannai, Etsuko
2009.
A survey on spherical designs and algebraic combinatorics on spheres.
European Journal of Combinatorics,
Vol. 30,
Issue. 6,
p.
1392.


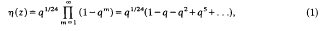
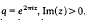 , Im(
, Im(

