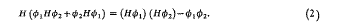Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-23T05:07:58.000Z Has data issue: false hasContentIssue false
  • English
  • Français

On Tricomi's relation for the Hilbert transformation

Published online by Cambridge University Press:  18 May 2009

P. G. Rooney
P. G. Rooney
Affiliation:
University of Toronto
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Tricomi [2] has shown that if φi ∈ Lpi (− ∞, ∞), i = 1, 2, where 1 < pi < ∞, (pi)–l + (P2)–1 < 1, and if H denotes the Hilbert transformation, that is

where the symbol (P) denotes that the integral is taken in the Cauchy principal value sense, then

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 16 , Issue 1 , March 1975 , pp. 52 - 56
Copyright
Copyright © Glasgow Mathematical Journal Trust 1975

References

REFERENCES

1.Titchmarsh, E. C., Theory of Fourier integrals, Second Edition (Oxford, 1948).Google Scholar
2.Tricomi, F. G., On the finite Hilbert transformation, Quart. J. Math. (2) 2, 1951, 199–211 (see also F. G. Tricomi, Integral equations, Interscience Publ. (New York, 1957), § 4.3).CrossRefGoogle Scholar