Published online by Cambridge University Press: 27 February 2017
Let (M, g(t)) be a compact Riemannian manifold and the metric g(t) evolve by the Ricci flow. In the paper, we prove that the eigenvalues of geometric operator −Δφ + 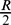 $\frac{R}{2}$ are non-decreasing under the Ricci flow for manifold M with some curvature conditions, where Δφ is the Witten Laplacian operator, φ ∈ C2(M), and R is the scalar curvature with respect to the metric g(t). We also derive the evolution of eigenvalues under the normalized Ricci flow. As a consequence, we show that compact steady Ricci breather with these curvature conditions must be trivial.
$\frac{R}{2}$ are non-decreasing under the Ricci flow for manifold M with some curvature conditions, where Δφ is the Witten Laplacian operator, φ ∈ C2(M), and R is the scalar curvature with respect to the metric g(t). We also derive the evolution of eigenvalues under the normalized Ricci flow. As a consequence, we show that compact steady Ricci breather with these curvature conditions must be trivial.