Hostname: page-component-6bf8c574d5-h6jzd Total loading time: 0.001 Render date: 2025-02-28T21:31:07.478Z Has data issue: false hasContentIssue false
  • English
  • Français

A CLASS OF CRITICAL KIRCHHOFF PROBLEM ON THE HYPERBOLIC SPACE 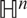$\mathbb{H}^{{\it n}}$

Part of: Partial differential equations on manifolds; differential operators Partial differential equations Miscellaneous topics - Partial differential equations Elliptic equations and systems

Published online by Cambridge University Press:  21 January 2019

PAULO CESAR CARRIÃO ,
AUGUSTO CÉSAR DOS REIS COSTA  and
OLIMPIO HIROSHI MIYAGAKI
PAULO CESAR CARRIÃO
Affiliation:
Departamento de Matemática, Universidade Federal de Minas Gerais, 31270-901 Belo Horizonte, Minas Gerais, Brazil e-mail: [email protected]
AUGUSTO CÉSAR DOS REIS COSTA
Affiliation:
Faculdade de Matemática, Instituto de Ciências Exatas e Naturais, Universidade Federal do Pará, 66075-110 Belém, PA, Brazil e-mail: [email protected]
OLIMPIO HIROSHI MIYAGAKI*
Affiliation:
Departamento de Matemática, Universidade Federal de Juiz de Fora, 36036-330 Juiz de Fora, Minas Gerais, Brazil e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We investigate questions on the existence of nontrivial solution for a class of the critical Kirchhoff-type problems in Hyperbolic space. By the use of the stereographic projection the problem becomes a singular problem on the boundary of the open ball 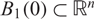$B_1(0)\subset \mathbb{R}^n$ Combining a version of the Hardy inequality, due to Brezis–Marcus, with the mountain pass theorem due to Ambrosetti–Rabinowitz are used to obtain the nontrivial solution. One of the difficulties is to find a range where the Palais Smale converges, because our equation involves a nonlocal term coming from the Kirchhoff term.

MSC classification

Primary: 58J05: Elliptic equations on manifolds, general theory
Secondary: 35R01: Partial differential equations on manifolds 35J60: Nonlinear elliptic equations 35B33: Critical exponents
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 62 , Issue 1 , January 2020 , pp. 109 - 122
Copyright
Copyright © Glasgow Mathematical Journal Trust 2019 

References

Alves, C. O., Corrêa, F. J. S. A. and Ma, T. F., Positive solutions for a quasilinear elliptic equation of Kirchhoff type, Comput. Math. Appl. 49 (2005), 85–93.CrossRefGoogle Scholar
Alves, C. O. and Figueiredo, G. M., Nonlinear perturbations of a periodic Kirchhoff equation in $\mathbb{R}^N $, Nonlinear Anal. 75 (2012), 2750–2759.CrossRefGoogle Scholar
Ambrosetti, A. and Struwe, M., A note on the problem Δu = λu + u|u|2*−2 Manuscripta Math. 54 (1986), 373–379.CrossRefGoogle Scholar
Arosio, A., and Panizzi, S., On the well-posedness of the Kirchhoff string, Trans. Amer. Math. Soc. 348 (1996), 305–330.CrossRefGoogle Scholar
Atkinson, F. V. and Peletier, A., Emden–Fowler equations involving critical exponents, Nonlinear Anal. 10 (1986), 755–176.CrossRefGoogle Scholar
Aubin, T. and Ekeland, I., Applied nonlinear analysis (Dover Publication, New York, 1984).Google Scholar
Autuori, G. and Pucci, P., Elliptic problems involving the fractional Laplacian in $\mathbb{R}^N$, J. Differ. Equ. 255 (2013), 2340–2362.CrossRefGoogle Scholar
Bandle, C. and Benguria, R., The Brezis–Nirenberg problem on ${\mathcal{S}}^N$, J. Differ. Equ. 178(1) (2002), 264–279.CrossRefGoogle Scholar
Bandle, C. and Kabeya, Y., On the positive, “radial” solutions of a semilinear elliptic equation in ${\mathbb{H}}^N$, Adv. Nonlinear Anal. 1(1) (2012), 1–25.CrossRefGoogle Scholar
Benci, V. and Cerami, G., Existence of positive solutions of the equation in $\Delta u + a(x)u = u^{\frac{n+2}{n-2}}$ in $\mathbb{R}^N$, J. Funct. Anal. 86 (1996), 90–117.Google Scholar
Benguria, S., The solution gap of the Brezis–Nirenberg problem on the hyperbolic space, Monatsh. Math. 181(3) (2016), 537–559.CrossRefGoogle Scholar
Benguria, R. and Benguria, S., The Brezis–Nirenberg problem on ${\mathcal{S}}^N$ in spaces of fractional dimension, arXiv:1503.06347 (2015).Google Scholar
Bhakta, M. and Sandeep, K., Poincaré–Sobolev equations in the hyperbolic spaces, Calc. Var. Partial Differ. Equ. 44 (2012), 247–269.CrossRefGoogle Scholar
Bianchi, G., Chabrowski, J. and Szulkin, A., On symmetric solutions of an elliptic equation with a nonlinearity involving critical Sobolev expoent, Nonlinear Anal. TMA 25(1) (1995), 41–59.CrossRefGoogle Scholar
Bonorino, L. P. and Klaser, P. K., Existence and nonexistence results for eigenfunctions of the Laplacian in unbounded domains of ${\mathcal{H}}^N$, arXiv:1310.3133 (2013).Google Scholar
Brezis, H., Nonlinear elliptic equations involving the critical Sobolev exponent: survey and perspectives, in Directions in partial differential equations (Crandall, M. G., Rabinowitz, P. H. and Turner, R. E. L., Editors) Academic Press, New York, 1987, pp. 17–36.CrossRefGoogle Scholar
Brezis, H. and Marcus, M., Hardy’s inequalities revisited, Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4), 25(1–2) (1997), 217–237.Google Scholar
Brezis, H., Marcus, M. and Shafrir, I., Extremal functions for Hardy’s inequality with weight, J. Func. Anal. 171(1) (2000), 177–191.CrossRefGoogle Scholar
Brezis, H. and Nirenberg, L., Positive solutions of nonlinear elliptic equations involving critical Sobolev exponents, Commun. Pure Appl. Math. 36 (1983), 437–477.CrossRefGoogle Scholar
Capozzi, A., Fortunato, D. and Palmieri, G., An existence result for nonlinear elliptic problems involving critical Sobolev exponent, Ann. Inst. H. Poincaré: Analyse non Lineaire 2(6) (1985), 463–470.CrossRefGoogle Scholar
Carrião, P. C., Miyagaki, O. H. and Pádua, J. C., Radial solutions of elliptic equations with critical exponents in $\mathbb{R}^N$, Differ. Integral Equ. 11(1) (1998), 61–68.Google Scholar
Carrião, P. C., Lehrer, R., Miyagaki, O. H. and Vicente, A., A nonhomogeneous Brezis–Nirenberg problem on the hyperbolic space ${\mathbb{H}}^n$, submitted.Google Scholar
Cerami, G., Fortunato, D. and Struwe, M., Bifurcation and multiplicity results for nonlinear elliptic problems involving critical Sobolev exponents, Ann Inst. H. Poincaré: Analyse non Lineaire l(5) (1985), 341–350.Google Scholar
Cerami, G., Solimini, S. and Struwe, M., Some existence results for superlinear elliptic boundary value problems involving critical exponents, J. Funct. Anal. 69 (1986), 289–306.CrossRefGoogle Scholar
Chen, C. Y., Kuo, Y. C. and Wu, T. F., The Nehari manifold for a Kirchhoff type problem involving signchanging weight functions, J. Differ. Equ. 250 (2011), 1876–1908.CrossRefGoogle Scholar
Cheng, B., Wu, S. and Liu, J., Multiplicity of nontrivial solutions for Kirchhoff type problems, Bound. Value Probl. 2010 (2010), 268946. doi: 10.1155/2010/268946CrossRefGoogle Scholar
Colasuonno, F. and Pucci, P., Multiplicity of solutions for p(x)-polyharmonic elliptic Kirchhoff equations. Nonlinear Anal. 74(17) (2011), 5962–5974.CrossRefGoogle Scholar
Corrêa, F. J. S. A. and Nascimento, R. G., On a nonlocal elliptic system of p-Kirchhoff type under Neumann boundary condition, Math. Comput. Modell. 49 (2009), 598–604.CrossRefGoogle Scholar
Dancer, E. N., A note on an equation with critical exponent, Bull. London Math. Soc. 20 (1988), 600–602.CrossRefGoogle Scholar
D’Ancona, P. and Spagnolo, S., Global solvability for the degenerate Kirchhoff equation with real analytic data, Invent. Math. 108 (1992), 247–262.CrossRefGoogle Scholar
Deng, Y. B., Zhong, H. S. and Zhu, X. P., On the existence and L p(R N) bifurcation for the semilinear elliptic equation, J. Math. Anal. Appl. 154 (1991), 116–133.CrossRefGoogle Scholar
Ding, W. Y. and Ni, W. M., On the elliptic equation $\Delta u + Ku^{\frac{n+2}{n-2}} = 0$ and related topics, Duke Math. J. 52 (1985), 485–506.CrossRefGoogle Scholar
Egnell, H., Existence and nonexistence results for m-Laplace equations involving critical Sobolevc exponents, Arch. Ration. Mech. Anal. 104 (1988), 57–77.CrossRefGoogle Scholar
Figueiredo, G. M., Existence of positive solution for a Kirchhoff problem type with critical growth via truncation argument. J. Math. Anal. Appl. 401 (2013), 706–713.CrossRefGoogle Scholar
Figueiredo, G. M. and Santos Junior, J., Multiplicity of solutions for a Kirchhoff equation with subcritical or critical growth, Differ. Integral Equ. 25 (2012), 853–868.Google Scholar
Ganguly, D. and Sandeep, K., Sign changing solutions of the Brezis–Nirenberg problem in the hyperbolic space, Calc. Var. Partial Differ. Equ. 50(1–2) (2014), 69–91.CrossRefGoogle Scholar
Ganguly, D. and Sandeep, K., Nondegeneracy of positive solutions of semilinear elliptic problems in the hyperbolic space, Commun. Contemp. Math. 17(1) (2015), 1450019.CrossRefGoogle Scholar
Garcia Azorero, J. and Peral Alonso, I., Multiplicity of solutions for elliptic problems with critical exponent or with a nonsymmetric term, Trans. Am. Math. Soc. 323(2) (1991), 877–895.CrossRefGoogle Scholar
García-Huidobro, M. and Yarur, C. S., On quasilinear Brezis–Nirenberg type problems with weights, Adv. Differ. Equ. 15(5–6) (2010), 401–436.Google Scholar
Guedda, M. and Veron, L., Quasilinear elliptic equations involving critical Sobolev exponents, Nonlinear Anal. 13(8) (1989), 879–902.CrossRefGoogle Scholar
He, H.-Y., Supercritical elliptic equation in hyperbolic space, J. Partial Differ. Equ. 28(2) (2015), 120–127.Google Scholar
He, H.-Y. and Li, G.-B., Standing waves for a class of Kirchhoff type problems in $\mathbb{R}^3$ involving critical Sobolev exponents, Calc. Var. 54 (2015), 3067–3106.CrossRefGoogle Scholar
He, H.-Y., Li, G.-B. and Peng, S.-J., Concentrationg bound states for Kirchhoff type problems in $\mathbb{R}^3$ involving critical Sobolev exponents, Adv. Nonl. Stud. 14 (2014), 483–510.Google Scholar
He, X. and Zou, W., Infinitely many positive solutions for Kirchhoff-type problems, Nonlinear Anal. 70 (2009), 1407–1414.CrossRefGoogle Scholar
Kirchhoff, G., Mechanik (Teubner, Leipzig, 1883).Google Scholar
Lions, J., On some questions in boundary value problems of mathematical physics, in Contemporary Developments in Continuum Mechanics and Partial Differential Equations. Proc. Internat. Sympos. Inst. Mat. Univ. Fed. Rio de Janeiro (1997). vol. 30 (North-Holland Mathematics Studies, Amsterdam, 1978), 284–346.Google Scholar
Liu, Z. and Guo, S., Existence and concentration of positive ground states for a Kirchhoff equation involving critical Sobolev exponent, Z. Angew. Math. Phys. 66 (2015), 747–769.CrossRefGoogle Scholar
Ma, T. and Rivera, J., Positive solutions for a nonlinear nonlocal elliptic transmission problem, Appl. Math. Lett. 16 (2003), 243–248.CrossRefGoogle Scholar
Mancini, G., and Sandeep, K., On a semilinear elliptic equation in $\mathcal{H}^N$, Ann. Sc. Norm. Super. Pisa Cl. Sci. 7(5) (2008), 635–671.Google Scholar
Miyagaki, O. H., On a class of semilinear elliptic problems in $\mathbb{R}^N$ with critical growth, Nonlinear Anal. Theory, Meth. Appl. 29(7) (1997), 773–781.CrossRefGoogle Scholar
Noussair, E. S., Swanson, C. A. and Yang, J., Positive finite energy solutions of critical semilinear elliptic problems, Can. J. Math. 44(5) (1992), 1014–1029.CrossRefGoogle Scholar
Palais, R. S., The Principle of Symmetric Criticality, Commun. Math. Phys. 69 (1979) 19–30.CrossRefGoogle Scholar
Perera, K. and Zhang, Z., Nontrivial solutions of Kirchhoff-type problems via the Yang index, J. Differ. Equ. 21 (2006), 246–255.CrossRefGoogle Scholar
Ratcliffe, J. G., Foundations of hyperbolic manifolds. Graduate Texts in Mathematics, vol. 149 (Springer, New York, 1994).CrossRefGoogle Scholar
Ricceri, B., On an elliptic Kirchhoff-type problem depending on two parameters, J. Global Optim. 46 (2010), 543–549.CrossRefGoogle Scholar
Schechter, M. and Zou, W.-M., On the Brezis–Nirenberg problem, Arch. Ration. Mech. Anal. 197(1) (2010), 337–356.CrossRefGoogle Scholar
Stapelkamp, S., The Brezis–Nirenberg problem on BN: existence and uniqueness of solutions, in Elliptic and Parabolic Problems, Rolduc and Gaeta, 2001 (World Scientific, Singapore, 2002), 283–290.Google Scholar
Stoll, S., Harmonic function theory on real hyperbolic space, Preliminary draft, http:citeseerx.ist.psu.edu.Google Scholar
Talenti, G., Best constants in Sobolev inequality, Ann. Math. Pura Appl. 110 (1976), 353–372.CrossRefGoogle Scholar
Wang, J., Tian, L., Xu, J. and Zhang, F., Multiplicity and concentration of positive solutions for a Kirchhoff type problem with critical growth, J. Differ. Equ. 253 (2012), 2314–2351.CrossRefGoogle Scholar
Willem, M., Minimax theorems (Birkhäuser Boston, Basel, Berlin, 1996).CrossRefGoogle Scholar
Yue, X.-R. and Zou, W.-M., Remarks on a Brezis–Nirenbergś result, J. Math. Anal. Appl. 425(2) (2015), 900–910.CrossRefGoogle Scholar
Zhu, X.-P. and Yang, J., The quasilinear elliptic equations on unbounded domain involving critical Sobolev exponent, J. Partial Differ. Equ. 2(2) (1989), 53–64.Google Scholar