Published online by Cambridge University Press: 18 May 2009
In a very recent paper [1], Basil Gordon discusses generalizations of Jacobi's identity
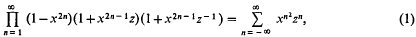
where x and z are complex numbers and |x| <1. He notes that some of its consequences, inter alia Euler's formula
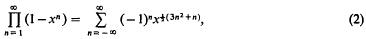
are of interest in number theory and combinatory analysis. He proves the apparently new and striking result
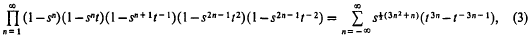
where |s|<1, and also considers the possibility of generalizations. His methods are algebraic and quite simple, but perhaps do not make obvious what underlies such formulae. It may be worth while to do so, especially since the details become simpler and the presentation more perspicuous. The method given here assumes no more knowledge than his does, although the new proof is expressed in terms of theta-functions, in simple properties of which, formulae such as (3) have their origin. Further, (3) appears in a slightly more symmetrical form.