Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-23T11:56:46.535Z Has data issue: false hasContentIssue false
  • English
  • Français

Prediction of long-term contributions and inbreeding in populations undergoing mass selection

Published online by Cambridge University Press:  14 April 2009

J. A. Woolliams ,
N. R. Wray  and
R. Thompson
J. A. Woolliams*
Affiliation:
AFRC Roslin Institute (Edinburgh), Roslin, Midlothian, EH 25 9PS, UK
N. R. Wray
Affiliation:
Livestock Improvement Unit, Victoria Department of Agriculture P.O. Box 500, East Melbourne, Victoria 3002, Australia
R. Thompson
Affiliation:
AFRC Roslin Institute (Edinburgh), Roslin, Midlothian, EH 25 9PS, UK
*
* Corresponding author.
Rights & Permissions [Opens in a new window]

Summary

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

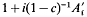For a population undergoing mass selection, derived from an unselected base population in generation zero, the expected long-term contribution to the population of an ancestor from generation 1 was shown to be equal to that expected during random selection multiplied by (where is one half the breeding value of the ancestor for the trait under selection standardized by the phenotypic standard deviation, i the intensity of selection, and 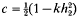is the competitiveness which is defined by 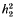the heritability in generation 2 and k the variance reduction coefficient). It was shown that the rate of inbreeding (ΔF) could be partitioned into three components arising from expected contributions, sampling errors and sampling covariances respectively. Using this result ΔF was derived and shown to be dominated by terms that describe ΔF by variance of family size in a single generation plus a term that accounts for the expected proliferation of lines over generations from superior ancestors in generation 1. The basic prediction of ΔF was given by

where M and F are the numbers of breeding males and females, T the number of offspring of each sex, ρm and ρt are correlations among half-sibs in generation 2 for males and females respectively, and K is a function of the intensity and competitiveness.

Type
Research Article
Information
Genetics Research , Volume 62 , Issue 3 , December 1993 , pp. 231 - 242
Copyright
Copyright © Cambridge University Press 1993

References

Bulmer, M. G. (1971). The effect of selection on genetic variability. American Naturalist 105, 201–221.CrossRefGoogle Scholar
Burrows, P. M. (1984 a). Inbreeding under selection for unrelated families. Biometrics 40, 357–366.CrossRefGoogle Scholar
Burrows, P. M. (1984 b). Inbreeding under selection from related families. Biometrics 40, 895–906.CrossRefGoogle Scholar
Hill, W. G. (1972). Effective population size of populations with overlapping generations. Theoretical Population Biology 3, 278–289.CrossRefGoogle ScholarPubMed
Latter, B. D. H. (1959). Genetic sampling in a random mating population of constant size and sex ratio. Australian Journal of Biological Science 12, 500–505.CrossRefGoogle Scholar
Lindgren, D. (1991). Optimal utilization of genetic resources. Forest Tree Improvement 23, 49–67.Google Scholar
Mendell, N. C. & Elston, R. C. (1974). Multifactorial traits: genetic analysis and prediction of recurrence risks. Biometrics 30, 41–57.CrossRefGoogle ScholarPubMed
Robertson, A. (1961). Inbreeding in artificial selection programmes. Genetical Research 2, 189–194.CrossRefGoogle Scholar
Tallis, G. M. (1961). The moment generating function of the truncated multi-normal distribution. Journal of the Royal Statistical Society B 23, 223–229.Google Scholar
Toro, M. A. & Neita, B. (1984). A simple method for increasing the response to artificial selection. Genetical Research 44, 347–349.CrossRefGoogle ScholarPubMed
Woolliams, J. A. (1989). Modification to MOET nucleus breeding schemes to improve rates of genetic progress and decrease rates of inbreeding in dairy cattle. Animal Production 49, 1–14.Google Scholar
Wray, N. R. (1989). Consequences of selection in finite populations with particular reference to closed nucleus herds of swine. Ph.D. Thesis, Edinburgh.Google Scholar
Wray, N. R. & Thompson, R. (1990). Prediction of rates of inbreeding in selected populations. Genetical Research 55, 41–54.CrossRefGoogle ScholarPubMed
Wray, N. R., Woolliams, J. A. & Thompson, R. (1990). Methods for predicting rates of inbreeding in selected populations. Theoretical Applied Genetics 80, 503–512.CrossRefGoogle ScholarPubMed
Verrier, E. (1989). Prédiction de l'évolution de la variance genétique dans les populations animales d'effectly limité soumises à selection. Ph.D. Thesis. INA Paris, Grignon, France.Google Scholar