Hostname: page-component-745bb68f8f-f46jp Total loading time: 0 Render date: 2025-01-10T20:41:06.544Z Has data issue: false hasContentIssue false
  • English
  • Français

CLUSTER STRUCTURES ON HIGHER TEICHMULLER SPACES FOR CLASSICAL GROUPS

Part of: Arithmetic rings and other special rings Basic linear algebra

Published online by Cambridge University Press:  08 May 2019

IAN LE
IAN LE*
Affiliation:
Perimeter Institute for Theoretical Physics, Waterloo, ON N2L 2Y5, Canada; [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

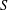Let $S$ be a surface, 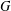$G$ a simply connected classical group, and $G^{\prime }$ the associated adjoint form of the group. We show that the moduli spaces of framed local systems 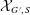${\mathcal{X}}_{G^{\prime },S}$ and 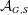${\mathcal{A}}_{G,S}$, which were constructed by Fock and Goncharov [‘Moduli spaces of local systems and higher Teichmuller theory’, Publ. Math. Inst. Hautes Études Sci.103 (2006), 1–212], have the structure of cluster varieties, and thus together form a cluster ensemble. This simplifies some of the proofs in that paper, and also allows one to quantize higher Teichmuller space, which was previously only possible when 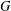$G$ was of type $A$.

MSC classification

Primary: 13F60: Cluster algebras
Secondary: 15A72: Vector and tensor algebra, theory of invariants
Type
Research Article
Information
Forum of Mathematics, Sigma , Volume 7 , 2019 , e13
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author 2019

References

Berenstein, A., Fomin, S. and Zelevinsky, A., ‘Cluster algebras III: upper bounds and double Bruhat cells’, Duke Math. J. 126(1) (2005), 1–52. arXiv:math.RT/0305434.Google Scholar
Cautis, S., Kamnitzer, J. and Morrison, S., ‘Webs and quantum skew Howe duality’, Mathe. Ann. 360(1–2) (2014), 351–390.Google Scholar
Fock, V. V. and Goncharov, A. B., ‘Moduli spaces of local systems and higher Teichmuller theory’, Publ. Math. Inst. Hautes Études Sci. 103 (2006), 1–212. arXiv:math.AG/0311149.Google Scholar
Fock, V. V. and Goncharov, A. B., ‘Cluster ensembles, quantization and the dilogarithm’, Ann. Sci. Éc. Norm. Supér. 42 (2009), 865–929. arXiv:math.AG/0311245.Google Scholar
Fock, V. V. and Goncharov, A. B., Cluster Ensembles, Quantization and the Dilogarithm II: The Intertwiner (Manin’s Festschrift, Birkhauser, 2007), arXiv:math.QA/070239.Google Scholar
Fock, V. V. and Goncharov, A. B., ‘Cluster 𝓧-varieties, amalgamation and Poisson-Lie groups’, Algebraic Geometry and Number Theory, 27–68. (Birkhauser, Boston, 2006), Honor of Vladimir Drinfeld on his 50th birthday.Google Scholar
Fock, V. V. and Goncharov, A. B., ‘The quantum dilogarithm and representations of quantum cluster varieties’, Invent. Math. 175 (2009), 223–286. arXiv:math.QA/0702397.Google Scholar
Fomin, S. and Pylyavskyy, P., ‘Webs on surfaces, rings of invariants, and clusters’, Proc. Natl. Acad. Sci. USA 111(27) (2014), 9680–9687.Google Scholar
Fomin, S. and Zelevinsky, A., ‘Double Bruhat cells and total positivity’, J. Amer. Math. Soc. 12 (1999), 335–380.Google Scholar
Fomin, S. and Zelevinsky, A., ‘Cluster algebras II: finite type classification’, Invent. Math. 154 (2003), 63–121.Google Scholar
Gaitto, D., Moore, G. and Neitzke, A., ‘Spectral networks’, Ann. Henri Poincaré 14 (2013), 1643–1731.Google Scholar
Goncharov, A.B. and Shen, L., ‘Geometry of canonical bases and mirror symmetry’, Invent. Math. 202 (2015), 487–633.Google Scholar
Goncharov, A.B. and Shen, L., ‘Donaldson-Thomas transformations of moduli spaces of G-local systems’, Invent. Math. 202 (2015), 487–633.Google Scholar
Henriques, A., An action of the cactus group, Mathematisches Forschungsinstitut Oberwolfach, Report No. 23/2007. Poisson Geom. Appl., arXiv:0705.3000.Google Scholar
Henriques, A. and Kamnitzer, J., ‘The octaheron recurrence and gl(n) crystals with A. Henriques’, Adv. Math. 206 (2006), 211–249.Google Scholar
Labourie, F., ‘Anosov flows, surface groups and curves in projective space’, Invent. Math. 165(1) (2006), 51–114.Google Scholar
Le., I., ‘Higher laminations and affine buildings’, Geom. Topol. 20(3) 1673–1735.Google Scholar
Le, I., ‘An approach to cluster structures on moduli of local systems for general groups’, Int. Math. Res. Not. rnx249.Google Scholar
Le, I. and Luo, S., ‘Generalized minors and tensor invariants’, arXiv:1901.09855.Google Scholar
Lusztig, G., ‘Total positivity and canonical bases’, Algebraic Groups and Lie Groups,(ed. Lehrer, G. I.) 281–295. (Cambridge University Press, Cambridge, 1997).Google Scholar
Lusztig, G., Total positivity in reductive groups, Lie theory and geometry, Progress in Mathematics, 123 (Birkhauser, Boston, MA, 1994), 531–568.Google Scholar
Robbins, D.P. and Rumsey, H., ‘Determinants and alternating-sign matrices’, Adv. Math. 62 (1986), 169–184.Google Scholar
Speyer, D., ‘Perfect matchings and the octahedron recurrence’, J. Algebraic Comb. 25(3) (2007), 309–348.Google Scholar
Teschner, J., ‘Quantization of moduli spaces of flat connections and Liouville theory’,arXiv:1405.0359.Google Scholar
Williams, H., ‘Cluster Ensembles and Kac–Moody Groups’, Adv. Math. 247 (2013), 1–40.Google Scholar
Zickert, C., ‘Fock-Goncharov coordinates for rank two Lie groups’, Preprint, 2016, arXiv:1605.08297.Google Scholar