Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-23T12:33:44.400Z Has data issue: false hasContentIssue false
  • English
  • Français

Coarse quantization for random interleaved sampling ofbandlimited signals∗∗∗

Published online by Cambridge University Press:  11 January 2012

Alexander M. Powell ,
Jared Tanner ,
Yang Wang  and
Özgür Yılmaz
Alexander M. Powell
Affiliation:
Department of Mathematics, Vanderbilt University, Nashville, 37240 TN, USA. [email protected]
Jared Tanner
Affiliation:
School of Mathematics, University of Edinburgh, King’s Buildings, Mayfield Road, EH9 3JL Edinburgh, UK; [email protected]
Yang Wang
Affiliation:
Department of Mathematics, Michigan State University, East Lansing, 48824 MI, USA; [email protected]
Özgür Yılmaz
Affiliation:
Department of Mathematics, University of British Columbia, 1984 Mathematics Road, Vancouver B.C., V6T 1Z2 Canada; [email protected]
Get access

Abstract

The compatibility of unsynchronized interleaved uniform sampling with Sigma-Deltaanalog-to-digital conversion is investigated. Let f be a bandlimitedsignal that is sampled on a collection of N interleaved grids {kT + Tn} k ∈ Zwith offsets \hbox{$\{T_n\}_{n=1}^N\subset [0,T]$}{Tn}n=1N⊂[0,T]. If the offsets Tn arechosen independently and uniformly at random from  [0,T]  and if thesample values of f are quantized with a first order Sigma-Deltaalgorithm, then with high probability the quantization error \hbox{$|f(t) - \widetilde{f}(t)|$}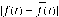|f(t)−􏽥f(t)|is at most of orderN-1log N.

Keywords

Analog-to-digital conversionbandlimited signalsinterleaved samplingrandom samplingsampling expansionsSigma-Delta quantization
Type
Research Article
Information
ESAIM: Mathematical Modelling and Numerical Analysis , Volume 46 , Issue 3: Special volume in honor of Professor David Gottlieb , May 2012 , pp. 605 - 618
Copyright
© EDP Sciences, SMAI, 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bass, R.F. and Gröchenig, K., Random sampling of multivariate trigonometric polynomials. SIAM J. Math. Anal. 36 (2005) 773–795. Google Scholar
Benedetto, J.J., Powell, A.M. and Yılmaz, Ö., Sigma-delta (ΣΔ) quantization and finite frames. IEEE Trans. Inf. Theory 52 (2006) 1990–2005. Google Scholar
Daubechies, I. and DeVore, R., Reconstructing a bandlimited function from very coarsely quantized data : A family of stable sigma-delta modulators of arbitrary order. Ann. Math. 158 (2003) 679–710. Google Scholar
H.A. David and H.N. Nagarja, Order Statistics, 3th edition. John Wiley & Sons, Hoboken, NJ (2003).
Devroye, L., Laws of the iterated logarithm for order statistics of uniform spacings. Ann. Probab. 9 (1981) 860–867. Google Scholar
R. Gervais, Q.I. Rahman and G. Schmeisser, A bandlimited function simulating a duration-limited one, in Anniversary volume on approximation theory and functional analysis (Oberwolfach, 1983), Internationale Schriftenreihe zur Numerischen Mathematik 65. Birkhäuser, Basel (1984) 355–362.
Güntürk, C.S., Approximating a bandlimited function using very coarsely quantized data : improved error estimates in sigma-delta modulation. J. Amer. Math. Soc. 17 (2004) 229–242. Google Scholar
Huestis, S., Optimum kernels for oversampled signals. J. Acoust. Soc. Amer. 92 (1992) 1172–1173. Google Scholar
Kunis, S. and Rauhut, H., Random sampling of sparse trigonometric polynomials II. orthogonal matching pursuit versus basis pursuit. Found. Comput. Math. 8 (2008) 737–763. Google Scholar
Natterer, F., Efficient evaluation of oversampled functions. J. Comput. Appl. Math. 14 (1986) 303–309. Google Scholar
Niland, R.A., Optimum oversampling. J. Acoust. Soc. Amer. 86 (1989) 1805–1812. Google Scholar
Slud, E., Entropy and maximal spacings for random partitions. Zeitschrift für Wahrscheinlichkeitstheorie und Verwandte Gebiete 41 (1977/78) 341–352. Google Scholar
Strohmer, T. and Tanner, J., Fast reconstruction methods for bandlimited functions from periodic nonuniform sampling. SIAM J. Numer. Anal. 44 (2006) 1073–1094. Google Scholar
C. Vogel and H. Johansson, Time-interleaved analog-to-digital converters : Status and future directions. Proceedings of the 2006 IEEE International Symposium on Circuits and Systems (ISCAS) (2006) 3386–3389.
J. Xu and T. Strohmer, Efficient calibration of time-interleaved adcs via separable nonlinear least squares. Technical Report, Dept. of Mathematics, University of California at Davis. http://www.math.ucdavis.edu/-strotimer/papers/2006/adc.pdf
Yılmaz, Ö., Coarse quantization of highly redundant time-frequency representations of square-integrable functions. Appl. Comput. Harmonic Anal. 14 (2003) 107–132. Google Scholar
A.I. Zayed, Advances in Shannon’s sampling theory. CRC Press, Boca Raton (1993).