Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-17T20:18:24.040Z Has data issue: false hasContentIssue false
  • English
  • Français

Self-affine sets with fibred tangents

Published online by Cambridge University Press:  28 January 2016

ANTTI KÄENMÄKI ,
HENNA KOIVUSALO  and
EINO ROSSI
ANTTI KÄENMÄKI
Affiliation:
University of Jyväskylä, Department of Mathematics and Statistics, P.O. Box 35 (MaD), FI-40014 University of Jyväskylä, Finland email [email protected], [email protected]
HENNA KOIVUSALO
Affiliation:
Department of Mathematics, University of York, Heslington, York YO10 5DD, UK email [email protected]
EINO ROSSI
Affiliation:
University of Jyväskylä, Department of Mathematics and Statistics, P.O. Box 35 (MaD), FI-40014 University of Jyväskylä, Finland email [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We study tangent sets of strictly self-affine sets in the plane. If a set in this class satisfies the strong separation condition and projects to a line segment for sufficiently many directions, then for each generic point there exists a rotation 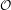${\mathcal{O}}$ such that all tangent sets at that point are either of the form 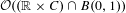${\mathcal{O}}((\mathbb{R}\times C)\cap B(0,1))$, where 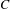$C$ is a closed porous set, or of the form ${\mathcal{O}}((\ell \times \{0\})\cap B(0,1))$, where 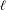$\ell$ is an interval.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 37 , Issue 6 , September 2017 , pp. 1915 - 1934
Copyright
© Cambridge University Press, 2016 

References

Bandt, C.. Local geometry of fractals given by tangent measure distributions. Monatsh. Math. 133(4) (2001), 265–280.Google Scholar
Bandt, C. and Käenmäki, A.. Local structure of self-affine sets. Ergod. Th. & Dynam. Sys. 33(5) (2013), 1326–1337.Google Scholar
Bedford, T. and Fisher, A. M.. On the magnification of Cantor sets and their limit models. Monatsh. Math. 121(1–2) (1996), 11–40.CrossRefGoogle Scholar
Bedford, T. and Fisher, A. M.. Ratio geometry, rigidity and the scenery process for hyperbolic Cantor sets. Ergod. Th. & Dynam. Sys. 17(3) (1997), 531–564.Google Scholar
Bedford, T., Fisher, A. M. and Urbański, M.. The scenery flow for hyperbolic Julia sets. Proc. Lond. Math. Soc. (3) 85(2) (2002), 467–492.Google Scholar
Buczolich, Z.. Micro tangent sets of continuous functions. Math. Bohem. 128(2) (2003), 147–167.Google Scholar
Buczolich, Z. and Ráti, C.. Micro tangent sets of typical continuous functions. Atti Semin. Mat. Fis. Univ. Modena Reggio Emilia 54(1–2) (2006), 135–166.Google Scholar
Chen, C. and Rossi, E.. Locally rich compact sets. Illinois J. Math. 58(3) (2014), 779–806.Google Scholar
Ferguson, A., Fraser, J. M. and Sahlsten, T.. Scaling scenery of (×m, ×n) invariant measures. Adv. Math. 268 (2015), 564–602.Google Scholar
Fraser, J. and Pollicott, M.. Micromeasure distributions and applications for conformally generated fractals. Math. Proc. Cambridge Philos. Soc. 159 (2015), 547–566.Google Scholar
Fraser, J. M.. Assouad type dimensions and homogeneity of fractals. Trans. Amer. Math. Soc. 366(12) (2014), 6687–6733.Google Scholar
Fraser, J. M., Henderson, A. M., Olson, E. J. and Robinson, J. C.. On the Assouad dimension of self-similar sets with overlaps. Adv. Math. 273 (2015), 188–214.Google Scholar
Furstenberg, H.. Noncommuting random products. Trans. Amer. Math. Soc. 108 (1963), 377–428.CrossRefGoogle Scholar
Furstenberg, H.. Intersections of Cantor sets and transversality of semigroups. Problems in Analysis (Symp. Salomon Bochner, Princeton University, Princeton, NJ, 1969). Princeton University Press, Princeton, NJ, 1970, pp. 41–59.Google Scholar
Furstenberg, H.. Ergodic fractal measures and dimension conservation. Ergod. Th. & Dynam. Sys. 28(2) (2008), 405–422.Google Scholar
Gavish, M.. Measures with uniform scaling scenery. Ergod. Th. & Dynam. Sys. 31(1) (2011), 33–48.Google Scholar
Hochman, M.. Dynamics on fractals and fractal distributions. Preprint, 2013, arXiv:1008.3731v2.Google Scholar
Hochman, M. and Shmerkin, P.. Equidistribution from fractal measures. Invent. Math. 202(1) (2015), 427–479.Google Scholar
Järvenpää, E., Järvenpää, M., Käenmäki, A., Rajala, T., Rogovin, S. and Suomala, V.. Packing dimension and Ahlfors regularity of porous sets in metric spaces. Math. Z. 266(1) (2010), 83–105.Google Scholar
Käenmäki, A. and Reeve, H. W. J.. Multifractal analysis of Birkhoff averages for typical infinitely generated self-affine sets. J. Fract. Geom. 1(1) (2014), 83–152.Google Scholar
Käenmäki, A., Sahlsten, T. and Shmerkin, P.. Dynamics of the scenery flow and geometry of measures. Proc. Lond. Math. Soc. (3) 110(5) (2015), 1248–1280.CrossRefGoogle Scholar
Käenmäki, A., Sahlsten, T. and Shmerkin, P.. Structure of distributions generated by the scenery flow. J. Lond. Math. Soc. (2) 91(2) (2015), 464–494.Google Scholar
Käenmäki, A. and Vilppolainen, M.. Separation conditions on controlled Moran constructions. Fund. Math. 200(1) (2008), 69–100.Google Scholar
Kempton, T.. The scenery flow for self-affine measures. Preprint, 2015, arXiv:1505.01663.Google Scholar
Mackay, J. M.. Assouad dimension of self-affine carpets. Conform. Geom. Dyn. 15 (2011), 177–187.Google Scholar
Mackay, J. M. and Tyson, J. T.. Conformal Dimension: Theory and Application (University Lecture Series, 54) . American Mathematical Society, Providence, RI, 2010.Google Scholar
O’Neil, T.. A local version of the projection theorem and other results in geometric measure theory. PhD Thesis, University College London, 1994.Google Scholar
O’Neil, T.. A measure with a large set of tangent measures. Proc. Amer. Math. Soc. 123(7) (1995), 2217–2220.CrossRefGoogle Scholar
Preiss, D.. Geometry of measures in R n : distribution, rectifiability, and densities. Ann. of Math. (2) 125(3) (1987), 537–643.Google Scholar
Ruelle, D.. Ergodic theory of differentiable dynamical systems. Inst. Hautes Études Sci. Publ. Math. 50 (1979), 27–58.Google Scholar
Sahlsten, T.. Tangent measures of typical measures. Real Anal. Exchange 40(1) (2015), 1–27.Google Scholar
Viana, M.. Lectures on Lyapunov Exponents (Cambridge Studies in Advanced Mathematics, 145) . Cambridge University Press, Cambridge, 2014.Google Scholar
Walters, P.. An Introduction to Ergodic Theory. Springer, New York, 1982.CrossRefGoogle Scholar
Xi, L.. Porosity of self-affine sets. Chin. Ann. Math. Ser. B 29(3) (2008), 333–340.Google Scholar
Zähle, U.. Self-similar random measures and carrying dimension. Proceedings of the Conference: Topology and Measure, V (Binz, 1987) Wissensch. Beitr. Ernst-Moritz-Arndt Univ, Greifswald, 1988, pp. 84–87.Google Scholar