Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T01:03:49.579Z Has data issue: false hasContentIssue false
  • English
  • Français

Mean dimension and a sharp embedding theorem: extensions of aperiodic subshifts

Published online by Cambridge University Press:  25 June 2013

YONATAN GUTMAN  and
MASAKI TSUKAMOTO
YONATAN GUTMAN
Affiliation:
Institute of Mathematics, Polish Academy of Sciences, ul. Śniadeckich 8, 00-956 Warszawa, Poland email [email protected]
MASAKI TSUKAMOTO
Affiliation:
Department of Mathematics, Kyoto University, Kyoto 606-8502, Japan email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

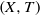We show that if $(X, T)$ is an extension of an aperiodic subshift (a subsystem of 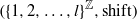$(\mathop{\{ 1, 2, \ldots , l\} }\nolimits ^{ \mathbb{Z} } , \mathrm{shift} )$ for some 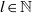$l\in \mathbb{N} $) and has mean dimension $\mathrm{mdim} (X, T)\lt (D/ 2), D\in \mathbb{N} $, then it can be equivariantly embedded in 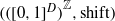$(\mathop{(\mathop{[0, 1] }\nolimits ^{D} )}\nolimits ^{ \mathbb{Z} } , \mathrm{shift} )$. The result is sharp. If 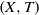$(X, T)$ is an extension of an aperiodic zero-dimensional system then it can be equivariantly embedded in 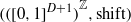$(\mathop{(\mathop{[0, 1] }\nolimits ^{D+ 1} )}\nolimits ^{ \mathbb{Z} } , \mathrm{shift} )$.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 34 , Issue 6 , December 2014 , pp. 1888 - 1896
Copyright
© Cambridge University Press, 2013 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Auslander, J.. Minimal Flows and their Extensions (North-Holland Mathematics Studies: Notas de Matemática [Mathematical Notes], 153). North-Holland, Amsterdam, 1988.Google Scholar
Gromov, M.. Topological invariants of dynamical systems and spaces of holomorphic maps. I. Math. Phys. Anal. Geom. 2 (4) (1999), 323–415.CrossRefGoogle Scholar
Gutman, Y.. Embedding ${ \mathbb{Z} }^{k} $-actions in cubical shifts and ${ \mathbb{Z} }^{k} $-symbolic extensions. Ergod. Th. & Dynam. Sys. 31 (2) (2011), 383–403.CrossRefGoogle Scholar
Gutman, Y.. Mean dimension and Jaworski-type theorems. Preprint, 2012, http://arxiv.org/abs/1208.5248.Google Scholar
Gutman, Y.. Dynamical embedding in cubical shifts and the topological Rokhlin and small boundary properties. Preprint, 2013, http://arxiv.org/abs/1301.6072.Google Scholar
Hurewicz, W. and Wallman, H.. Dimension Theory (Princeton Mathematical Series, 4). Princeton University Press, Princeton, NJ, 1941.Google Scholar
Jaworski, A.. The Kakutani–Beboutov theorem for groups. PhD Dissertation, University of Maryland, 1974.Google Scholar
Kakutani, S.. Induced measure preserving transformations. Proc. Imp. Acad. Tokyo 19 (1943), 635–641.Google Scholar
Lindenstrauss, E.. Mean dimension, small entropy factors and an embedding theorem. Publ. Math. Inst. Hautes Études Sci. 89 (1) (1999), 227–262.Google Scholar
Lindenstrauss, E. and Tsukamoto, M.. Mean dimension and an embedding problem: an example. Israel J. Math., to appear. Preprint, 2012.Google Scholar
Lindenstrauss, E. and Weiss, B.. Mean topological dimension. Israel J. Math. 115 (2000), 1–24.CrossRefGoogle Scholar
Matoušek, J.. Using the Borsuk–Ulam theorem. Universitext (Lectures on Topological Methods in Combinatorics and Geometry). Springer, Berlin, 2003, written in cooperation with Anders Björner and Günter M. Ziegler.Google Scholar
Petersen, K.. Ergodic Theory (Cambridge Studies in Advanced Mathematics, 2). Cambridge University Press, Cambridge, 1983.Google Scholar
Williams, S. G.. Introduction to symbolic dynamics. Symbolic Dynamics and its Applications (Proceedings of Symposia in Applied Mathematics, 60). American Mathematical Society, Providence, RI, 2004, pp. 1–11.CrossRefGoogle Scholar