Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bishop, Christopher J.
and
Jones, Peter W.
2002.
Compact deformations of Fuchsian groups.
Journal d'Analyse Mathématique,
Vol. 87,
Issue. 1,
p.
5.
Huo, Sheng Jin
and
Wu, Sheng Jian
2016.
Hausdorff dimensions of quasilines and differentiability of quasisymmetric homeomorphisms.
Acta Mathematica Sinica, English Series,
Vol. 32,
Issue. 4,
p.
499.
Ransford, Thomas
Younsi, Malik
and
Ai, Wen-hui
2020.
Continuity of capacity of a holomorphic motion.
Advances in Mathematics,
Vol. 374,
Issue. ,
p.
107376.
Mayer, Volker
and
Zdunik, Anna
2021.
The failure of Ruelle's property for entire functions.
Advances in Mathematics,
Vol. 384,
Issue. ,
p.
107723.
HUO, SHENGJIN
and
ZINSMEISTER, MICHEL
2022.
On Ruelle’s property.
Ergodic Theory and Dynamical Systems,
Vol. 42,
Issue. 4,
p.
1474.
Fuhrer, Aidan
Ransford, Thomas
and
Younsi, Malik
2023.
Holomorphic motions, dimension, area and quasiconformal mappings.
Journal de Mathématiques Pures et Appliquées,
Vol. 177,
Issue. ,
p.
455.


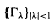 of infinitely generated quasi-Fuchsian groups such that the Hausdorff dimension of the limit set
of infinitely generated quasi-Fuchsian groups such that the Hausdorff dimension of the limit set 