Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Evans, David E.
and
Kawahigashi, Yasuyuki
1994.
Orbifold subfactors from Hecke algebras.
Communications in Mathematical Physics,
Vol. 165,
Issue. 3,
p.
445.
Elliott, George A.
1995.
Proceedings of the International Congress of Mathematicians.
p.
922.
Walters, Samuel G.
1995.
Inductive limit automorphisms of the irrational rotation algebra.
Communications in Mathematical Physics,
Vol. 171,
Issue. 2,
p.
365.
Haworth, P.A.
and
Power, S.C.
2002.
The Uniqueness of AF Diagonals in Regular Limit Algebras.
Journal of Functional Analysis,
Vol. 195,
Issue. 2,
p.
207.
Phillips, N. Christopher
2005.
Crossed Products of the Cantor Set by Free Minimal Actions of ?d.
Communications in Mathematical Physics,
Vol. 256,
Issue. 1,
p.
1.
THOMSEN, KLAUS
2010.
The homoclinic and heteroclinic C*-algebras of a generalized one-dimensional solenoid.
Ergodic Theory and Dynamical Systems,
Vol. 30,
Issue. 1,
p.
263.
Evans, D. Gwion
and
Sims, Aidan
2012.
When is the Cuntz–Krieger algebra of a higher-rank graph approximately finite-dimensional?.
Journal of Functional Analysis,
Vol. 263,
Issue. 1,
p.
183.
SCARPARO, EDUARDO
2020.
Homology of odometers.
Ergodic Theory and Dynamical Systems,
Vol. 40,
Issue. 9,
p.
2541.
PROIETTI, VALERIO
and
YAMASHITA, MAKOTO
2022.
Homology andK-theory of dynamical systems I. Torsion-free ample groupoids.
Ergodic Theory and Dynamical Systems,
Vol. 42,
Issue. 8,
p.
2630.


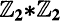
 is an AF-algebra.
is an AF-algebra.