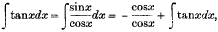Research Article
On Extraneous Values in Simultaneous Equations
-
- Published online by Cambridge University Press:
- 31 October 2008, pp. 183-184
-
- Article
-
- You have access
- Export citation
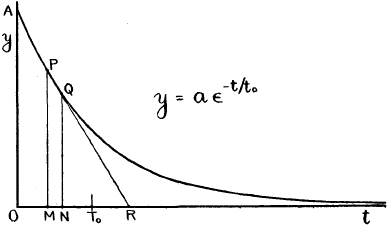
An Exact Geometrical Construction for the Exponential Curve
-
- Published online by Cambridge University Press:
- 31 October 2008, pp. 184-186
-
- Article
-
- You have access
- Export citation
Integration by Parts. A Failing Case
-
- Published online by Cambridge University Press:
- 31 October 2008, pp. 186-187
-
- Article
-
- You have access
- Export citation
Vectors as applied to Problems in Velocity
-
- Published online by Cambridge University Press:
- 31 October 2008, pp. 187-190
-
- Article
-
- You have access
- Export citation
Front matter
MAN volume 16 Cover and Front matter
-
- Published online by Cambridge University Press:
- 31 October 2008, pp. f1-f2
-
- Article
-
- You have access
- Export citation