Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-26T11:53:56.908Z Has data issue: false hasContentIssue false
  • English
  • Français

NONPARAMETRIC IDENTIFICATION AND ESTIMATION OF A GENERALIZED ADDITIVE MODEL WITH A FLEXIBLE ADDITIVE STRUCTURE AND UNKNOWN LINK

Published online by Cambridge University Press:  04 December 2024

Songnian Chen Open the ORCID record for Songnian Chen [Opens in a new window] ,
Nianqing Liu ,
Jian Zhang Open the ORCID record for Jian Zhang [Opens in a new window]  and
Yahong Zhou
Songnian Chen
Affiliation:
Zhejiang University
Nianqing Liu
Affiliation:
Xiamen University
Jian Zhang*
Affiliation:
Nankai University
Yahong Zhou
Affiliation:
Shanghai University of Finance and Economics and Shanghai Institute for Mathematics and Interdisciplinary Sciences
*
Address correspondence to Jian Zhang, School of Economics, Nankai University, Tianjin, China; e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper proposes a nonparametric approach to identify and estimate the generalized additive model with a flexible additive structure and with possibly discrete variables when the link function is unknown. Our approach allows for a flexible additive structure which provides applied researchers the flexibility to specify their model according to economic theory or practical experience. Motivated by the concerns from empirical research, our method also allows for multiple discrete variables in the covariates. By transforming our model into a generalized additive model with univariate component functions, our identification and estimation thereby follows a procedure adapted from the case with univariate components. The estimators converge to normal distributions in large sample with a one-dimensional convergence rate for the link function and a $d_k$-dimensional convergence rate for the component function 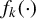$f_k(\cdot )$ defined on 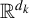${\mathbb R}^{d_k}$ for all k.

Type
ARTICLES
Information
Econometric Theory , First View , pp. 1 - 27
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Footnotes

We thank the Co-Editor (Liangjun Su) and three anonymous referees for comments that have greatly improved the article. We also thank the Editor (Peter C.B. Phillips) for his help on improving the presentation of this article. We are very grateful to Chunrong Ai, Xiaohong Chen, Yanqin Fan, LungFei Lee, and Arthur Lewbel for their constructive comments and suggestions. We are also grateful to Liang Chen, Timothy Christensen, Qingfeng Liu, Ryo Okui, Quang Vuong, Hanghui Zhang, Xinyu Zhang, and seminar/conference participants at HKUST, SUFE, Xiamen University, 2019 Asian Meeting of Econometric Society, 2019 China Meeting of Econometric Society, and 2019 Young Econometrician Asia Pacific (YEAP) for helpful comments. The usual disclaimer applies. Liu acknowledges support from the National Natural Science Foundation of China (Grant No. 72394392). Zhou acknowledges support from the National Natural Science Foundation of China (Grant Nos. 72342034 and 72173083). Zequn Jin provided capable research assistance.

References

REFERENCES

Ai, C., & Chen, X. (2003). Efficient estimation of models with conditional moment restrictions containing unknown functions. Econometrica , 71(6), 1795–1843.CrossRefGoogle Scholar
Antras, P. (2004). Is the US aggregate production function Cobb–Douglas? New estimates of the elasticity of substitution. Contributions in Macroeconomics , 4(1), Article 20121005.CrossRefGoogle Scholar
Athey, S., & Haile, P. A. (2002). Identification of standard auction models. Econometrica , 70(6), 2107–2140.CrossRefGoogle Scholar
Berkowitz, D., Ma, H., & Nishioka, S. (2017). Recasting the iron rice bowl: The reform of China’s state-owned enterprises. The Review of Economics and Statistics , 99(4), 735–747.CrossRefGoogle Scholar
Chen, R., Härdle, W., Linton, O. B., & Severance-Lossin, E. (1996). Nonparametric estimation of additive separable regression models. In Statistical theory and computational aspects of smoothing (pp. 247–265). Springer.CrossRefGoogle Scholar
Chen, S. (2002). Rank estimation of transformation models. Econometrica , 70(4), 1683–1697.CrossRefGoogle Scholar
Chen, S. (2010a). An integrated maximum score estimator for a generalized censored quantile regression model. Journal of Econometrics , 155(1), 90–98.CrossRefGoogle Scholar
Chen, S. (2010b). Root-N-consistent estimation of fixed-effect panel data transformation models with censoring. Journal of Econometrics , 159(1), 222–234.CrossRefGoogle Scholar
Chen, S. (2012). Distribution-free estimation of the Box–Cox regression model with censoring. Econometric Theory , 28(3), 680–695.CrossRefGoogle Scholar
Chen, S., & Zhang, H. (2020). Root-N-prediction of generalized heteroscedastic transformation regression models. Journal of Econometrics , 215(2), 305–340.CrossRefGoogle Scholar
Chen, X. (2007). Large sample sieve estimation of semi-nonparametric models. Handbook of Econometrics , 6, 5549–5632.CrossRefGoogle Scholar
Chesher, A. (2003). Identification in nonseparable models. Econometrica , 71(5), 1405–1441.CrossRefGoogle Scholar
Chung, K. L. (2001). A course in probability theory . Academic Press.Google Scholar
De Paula, A., & Tang, X. (2012). Inference of signs of interaction effects in simultaneous games with incomplete information. Econometrica , 80(1), 143–172.Google Scholar
Fan, J., & Gijbels, I. (1992). Variable bandwidth and local linear regression smoothers. Annals of Statistics , 20(4), 2008–2036.CrossRefGoogle Scholar
Gentry, M., & Li, T. (2014). Identification in auctions with selective entry. Econometrica , 82(1), 315–344.Google Scholar
Grieco, P. L. (2014). Discrete games with flexible information structures: An application to local grocery markets. The RAND Journal of Economics , 45(2), 303–340.CrossRefGoogle Scholar
Guerre, E., Perrigne, I., & Vuong, Q. (2000). Optimal nonparametric estimation of first-price auctions. Econometrica , 68(3), 525–574.CrossRefGoogle Scholar
Guerre, E., Perrigne, I., & Vuong, Q. (2009). Nonparametric identification of risk aversion in first-price auctions under exclusion restrictions. Econometrica , 77(4), 1193–1227.Google Scholar
Hahn, J., Liao, Z., & Ridder, G. (2018). Nonparametric two-step sieve M estimation and inference. Econometric Theory , 34(6), 1281–1324.CrossRefGoogle Scholar
Hall, P. (1992). On bootstrap confidence intervals in nonparametric regression. Annals of Statistics , 20(2), 695–711.CrossRefGoogle Scholar
Hall, P., & Horowitz, J. (2013). A simple bootstrap method for constructing nonparametric confidence bands for functions. Annals of Statistics , 41(4), 1892–1921.CrossRefGoogle Scholar
Hansen, B. E. (2009). Lecture notes on nonparametrics . University of Wisconsin–Madison.Google Scholar
Härdle, W., & Bowman, A. W. (1988). Bootstrapping in nonparametric regression: Local adaptive smoothing and confidence bands. Journal of the American Statistical Association , 83(401), 102–110.Google Scholar
Hoderlein, S., Su, L., White, H., & Yang, T. T. (2016). Testing for monotonicity in unobservables under unconfoundedness. Journal of Econometrics , 193(1), 183–202.CrossRefGoogle Scholar
Hodges, D. J. (1969). A note on estimation of Cobb–Douglas and CES production function models. Econometrica , 37(4), 721–725.CrossRefGoogle Scholar
Horowitz, J. (1998). Nonparametric estimation of a generalized additive model with an unknown link function [Working paper]. University of Iowa.Google Scholar
Horowitz, J. L. (2001). Nonparametric estimation of a generalized additive model with an unknown link function. Econometrica , 69(2), 499–513.CrossRefGoogle Scholar
Horowitz, J. L., & Mammen, E. (2004). Nonparametric estimation of an additive model with a link function. Annals of Statistics , 32(6), 2412–2443.CrossRefGoogle Scholar
Horowitz, J. L., & Mammen, E. (2007). Rate-optimal estimation for a general class of nonparametric regression models with unknown link functions. Annals of Statistics , 35(6), 2589–2619.CrossRefGoogle Scholar
Horowitz, J. L., & Mammen, E. (2011). Oracle-efficient nonparametric estimation of an additive model with an unknown link function. Econometric Theory , 27(3), 582–608.CrossRefGoogle Scholar
Jacho-Chávez, D., Lewbel, A., & Linton, O. (2010). Identification and nonparametric estimation of a transformed additively separable model. Journal of Econometrics , 156(2), 392–407.CrossRefGoogle Scholar
Khan, S. (2001). Two-stage rank estimation of quantile index models. Journal of Econometrics , 100(2), 319–355.CrossRefGoogle Scholar
Klump, R., McAdam, P., & Willman, A. (2007). Factor substitution and factor-augmenting technical progress in the United States: A normalized supply-side system approach. The Review of Economics and Statistics , 89(1), 183–192.CrossRefGoogle Scholar
Kmenta, J. (1967). On estimation of the CES production function. International Economic Review , 8(2), 180–189.CrossRefGoogle Scholar
Kohler, M., & Krzyżak, A. (2017). Nonparametric regression based on hierarchical interaction models. IEEE Transactions on Information Theory , 63(3), 1620–1630.CrossRefGoogle Scholar
Kong, E., Linton, O., & Xia, Y. (2010). Uniform Bahadur representation for local polynomial estimates of M-regression and its application to the additive model. Econometric Theory , 26(5), 1529–1564.CrossRefGoogle Scholar
Lewbel, A., Lu, X., & Su, L. (2015). Specification testing for transformation models with an application to generalized accelerated failure-time models. Journal of Econometrics , 184(1), 81–96.CrossRefGoogle Scholar
Lewbel, A., & Tang, X. (2015). Identification and estimation of games with incomplete information using excluded regressors. Journal of Econometrics , 189(1), 229–244.CrossRefGoogle Scholar
Li, H., & Liu, N. (2018). Nonparamametric identification and estimation of double auctions with bargaining [Working paper]. Shanghai University of Finance and Economics.Google Scholar
Li, Q., & Racine, J. S. (2007). Nonparametric econometrics: Theory and practice . Princeton University Press.Google Scholar
Li, T., & Zheng, X. (2009). Entry and competition effects in first-price auctions: Theory and evidence from procurement auctions. The Review of Economic Studies , 76(4), 1397–1429.CrossRefGoogle Scholar
Lin, H., Pan, L., Lv, S., & Zhang, W. (2018). Efficient estimation and computation for the generalised additive models with unknown link function. Journal of Econometrics , 202(2), 230–244.CrossRefGoogle Scholar
Linton, O., & Härdle, W. (1996). Estimation of additive regression models with known links. Biometrika , 83(3), 529–540.CrossRefGoogle Scholar
Liu, N., & Luo, Y. (2017). A nonparametric test for comparing valuation distributions in first-price auctions. International Economic Review , 58(3), 857–888.CrossRefGoogle Scholar
Liu, N., & Vuong, Q. (2020). Nonparametric tests for monotonicity of strategies in games of incomplete information [Working paper]. New York University.Google Scholar
Liu, N., Vuong, Q., & Xu, H. (2017). Rationalization and identification of binary games with correlated types. Journal of Econometrics , 201(2), 249–268.CrossRefGoogle Scholar
Ma, S. (2012). Two-step spline estimating equations for generalized additive partially linear models with large cluster sizes. Annals of Statistics , 40(6), 2943–2972.CrossRefGoogle Scholar
Ma, S., & Song, P. X.-K. (2015). Varying index coefficient models. Journal of the American Statistical Association , 110(509), 341–356.CrossRefGoogle Scholar
Marmer, V., & Shneyerov, A. (2012). Quantile-based nonparametric inference for first-price auctions. Journal of Econometrics , 167(2), 345–357.CrossRefGoogle Scholar
Masry, E. (1996). Multivariate local polynomial regression for time series: uniform strong consistency and rates. Journal of Time Series Analysis , 17(6), 571–599.CrossRefGoogle Scholar
Matzkin, R. L. (2003). Nonparametric estimation of nonadditive random functions. Econometrica , 71(5), 1339–1375.CrossRefGoogle Scholar
Paraskevopoulos, C. C. (1979). Alternative estimates of the elasticity of substitution: An inter-metropolitan CES production function analysis of US manufacturing industries, 1958–1972. The Review of Economics and Statistics , 61(3), 439–442.CrossRefGoogle Scholar
Pinkse, J. (2001). Nonparametric regression estimation using weak separability [Working paper]. University of British Columbia.Google Scholar
Pollard, D. (1984). Convergence of stochastic processes . Springer-Verlag.CrossRefGoogle Scholar
Powell, J. L., Stock, J. H., & Stoker, T. M. (1989). Semiparametric estimation of index coefficients. Econometrica , 57(6), 1403–1430.CrossRefGoogle Scholar
Schmidt-Hieber, J. (2020). Nonparametric regression using deep neural networks with ReLU activation function. Annals of Statistics , 48(4), 1875–1897.Google Scholar
Silverman, B. W. (1986). Density estimation for statistics and data analysis . Chapman and Hall/CRC.Google Scholar
Tang, X. (2010). Estimating simultaneous games with incomplete information under median restrictions. Economics Letters , 108(3), 273–276.CrossRefGoogle Scholar
Supplementary material: File

Chen et al. supplementary material

Chen et al. supplementary material
Download Chen et al. supplementary material(File)
File 225.5 KB