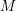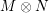Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Oh, Se-jin
2016.
Auslander-Reiten quiver of type A and generalized quantum affine Schur-Weyl duality.
Transactions of the American Mathematical Society,
Vol. 369,
Issue. 3,
p.
1895.
Kang, Seok-Jin
Kashiwara, Masaki
Kim, Myungho
and
Oh, Se-Jin
2016.
Symmetric quiver Hecke algebras and R-matrices of quantum affine algebras IV.
Selecta Mathematica,
Vol. 22,
Issue. 4,
p.
1987.
Lapid, Erez
and
Mínguez, Alberto
2016.
On parabolic induction on inner forms of the general linear group over a non-archimedean local field.
Selecta Mathematica,
Vol. 22,
Issue. 4,
p.
2347.
Kang, Seok-Jin
Kashiwara, Masaki
Kim, Myungho
and
Oh, Se-jin
2017.
Monoidal categorification of cluster algebras.
Journal of the American Mathematical Society,
Vol. 31,
Issue. 2,
p.
349.
McNamara, Peter J.
2017.
Representations of Khovanov–Lauda–Rouquier algebras III: symmetric affine type.
Mathematische Zeitschrift,
Vol. 287,
Issue. 1-2,
p.
243.
Lapid, Erez
and
Mínguez, Alberto
2018.
Geometric conditions for □-irreducibility of certain representations of the general linear group over a non-archimedean local field.
Advances in Mathematics,
Vol. 339,
Issue. ,
p.
113.
Kashiwara, Masaki
Kim, Myungho
Oh, Se-jin
and
Park, Euiyong
2018.
Monoidal categories associated with strata of flag manifolds.
Advances in Mathematics,
Vol. 328,
Issue. ,
p.
959.
MOURA, A.
and
PEREIRA, F.
2018.
ON TENSOR PRODUCTS OF A MINIMAL AFFINIZATION WITH AN EXTREME KIRILLOV-RESHETIKHIN MODULE FOR TYPE A.
Transformation Groups,
Vol. 23,
Issue. 4,
p.
989.
McNamara, Peter J.
2019.
Folding KLR algebras.
Journal of the London Mathematical Society,
Vol. 100,
Issue. 2,
p.
447.
Kashiwara, Masaki
Kim, Myungho
and
Oh, Se-jin
2019.
Monoidal categories of modules over quantum affine algebras of type A and B.
Proceedings of the London Mathematical Society,
Vol. 118,
Issue. 1,
p.
43.
Kashiwara, Masaki
and
Oh, Se-jin
2019.
Categorical relations between Langlands dual quantum affine algebras: doubly laced types.
Journal of Algebraic Combinatorics,
Vol. 49,
Issue. 4,
p.
401.
Walker, Ruari
2019.
Convolution products and R-matrices for type B quiver Hecke algebras.
Communications in Algebra,
Vol. 47,
Issue. 7,
p.
2634.
Oh, Se-jin
2019.
Auslander–Reiten quiver and representation theories related to KLR-type Schur–Weyl duality.
Mathematische Zeitschrift,
Vol. 291,
Issue. 1-2,
p.
499.
Hernandez, David
and
Oya, Hironori
2019.
Quantum Grothendieck ring isomorphisms, cluster algebras and Kazhdan-Lusztig algorithm.
Advances in Mathematics,
Vol. 347,
Issue. ,
p.
192.
Oh, Se-jin
and
Scrimshaw, Travis
2019.
Categorical Relations Between Langlands Dual Quantum Affine Algebras: Exceptional Cases.
Communications in Mathematical Physics,
Vol. 368,
Issue. 1,
p.
295.
Kashiwara, Masaki
and
Kim, Myungho
2019.
Laurent phenomenon and simple modules of quiver Hecke algebras.
Compositio Mathematica,
Vol. 155,
Issue. 12,
p.
2263.
Suh, Uhi Rinn
and
Oh, Se-jin
2019.
Twisted and folded Auslander-Reiten quivers and applications to the representation theory of quantum affine algebras.
Journal of Algebra,
Vol. 535,
Issue. ,
p.
53.
Mitra, Arnab
and
Sayag, Eitan
2020.
Models of Representations and Langlands Functoriality.
Canadian Journal of Mathematics,
Vol. 72,
Issue. 3,
p.
676.
Lapid, Erez
and
Mínguez, Alberto
2020.
Conjectures and results about parabolic induction of representations of $${\text {GL}}_n(F)$$.
Inventiones mathematicae,
Vol. 222,
Issue. 3,
p.
695.
Kim, Myungho
and
Park, Euiyong
2020.
A remark on convolution products for quiver Hecke algebras.
International Journal of Mathematics,
Vol. 31,
Issue. 11,
p.
2050092.